Big Ideas Math Algebra 2 Answers Chapter 11 Data Analysis and Statistics

Big Ideas Math Algebra 2 Answers Chapter 11 Data Analysis and Statistics assists students to learn strong fundamentals of concepts jotted down in this chapter. Download the BIM Algebra 2 Solution Book of Ch 11 Pdf for free of cost and kickstart your preparation with the related lessons of Data Analysis and Statistics. These BigIdeas Math Algebra 2 Chapter 11 Solution Key is aligned according to the Common Core Standards BIM Textbooks by experienced subject professionals. Hence, you can solve all the concepts of Data Analysis and Statistics questions during homework, assignments, alongside in the exams with utmost confidence.

Big Ideas Math Book Algebra 2 Answer Key Chapter 11 Data Analysis and Statistics

After doing deep research and meets the Common Core Curriculum, subject experts solved the questions covered in Big Ideas Math Book Algebra 2 Solutions Chapter 11 Data Analysis and Statistics in an explanative manner. With the help of step-by-step explanative solutions, students can easily grasp the concepts and memorize the method to solve the rest of the questions. So, simply click on the below available links for downloading the BIM Textbook Algebra 2 Ch 11 Data Analysis and Statistics and clarify your queries regarding the subject in seconds.

• Data Analysis and Statistics Maintaining Mathematical Proficiency – Page 593
• Data Analysis and Statistics Mathematical Practices – Page 594
• Lesson 11.1 Using Normal Distributions – Page(597-602)
• Using Normal Distributions 11.1 Exercises – Page(600 – 602)
• Lesson 11.2 Populations, Samples, and Hypotheses – Page(603 – 608)
• Populations, Samples, and Hypotheses 11.2 Exercises – Page(607 – 608)
• Lesson 11.3 Collecting Data – Page(609 – 616)
• Collecting Data 11.3 Exercises – Page(614 – 616)
• Data Analysis and Statistics Study Skills: Reworking Your Notes – Page 617
• Data Analysis and Statistics 11.1–11.3 Quiz – Page 618
• Lesson 11.4 Experimental Design – Page(619 – 624)
• Experimental Design 11.4 Exercises – Page(623 – 624)
• Lesson 11.5 Making Inferences from Sample Surveys – Page(625 – 632)
• Making Inferences from Sample Surveys 11.5 Exercises – Page(630 – 632)
• Lesson 11.6 Making Inferences from Experiments – Page(633 – 638)
• Making Inferences from Experiments 11.6 Exercises – Page(637 – 638)
• Data Analysis and Statistics Performance Task: Curving the Test – Page 639
• Data Analysis and Statistics Chapter Review – Page(640 – 642)
• Data Analysis and Statistics Chapter Test – Page 643
• Data Analysis and Statistics Cumulative Assessment – Page(644 – 646)

Data Analysis and Statistics Maintaining Mathematical Proficiency

Find the mean, median, and mode of the data set. Then determine which measure of center best represents the data. Explain.
Question 1.
36, 82, 94, 83, 86, 82
Answer:

Question 2.
74, 89, 71, 70, 68, 70
Answer:

Question 3.
1, 18, 12, 16, 11, 15, 17, 44, 44
Answer:

Find and interpret the standard deviation of the data set.
Question 4.
43, 48, 41, 51, 42
Answer:

Question 5.
28, 26, 21, 44, 29, 32
Answer:

Question 6.
65, 56, 49, 66, 62, 52, 53, 49
Answer:

Question 7.
ABSTRACT REASONING
Describe a data set that has a standard deviation of zero. Can a standard deviation be negative? Explain your reasoning.
Answer:

Data Analysis and Statistics Mathematical Practices

Mathematically proficient students use diagrams and graphs to show relationships between data. They also analyze data to draw conclusions.

Monitoring Progress

Use the Internet or some other reference to determine which age pyramid is that of Canada, Japan, and Mexico. Compare the mean, median, and mode of the three age pyramids.
Question 1.

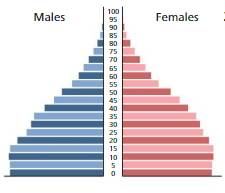
Answer:

Question 2.

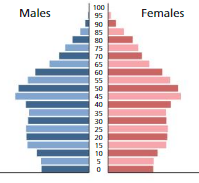
Answer:

Question 3.

Answer:

Lesson 11.1 Using Normal Distributions

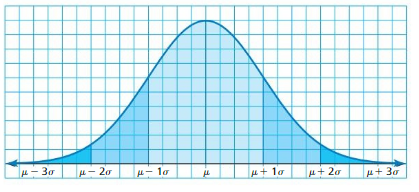
Essential Question In a normal distribution, about what percent of the data lies within one, two, and three standard deviations of the mean?
Recall that the standard deviation σ of a numerical data set is given by
σ = \(\sqrt{\frac{\left(x_{1}-\mu\right)^{2}+\left(x_{2}-\mu\right)^{2}+\cdots+\left(x_{n}-\mu\right)^{2}}{n}}\)
where n is the number of values in the data set and μ is the mean of the data set.

EXPLORATION 1

Analyzing a Normal Distribution
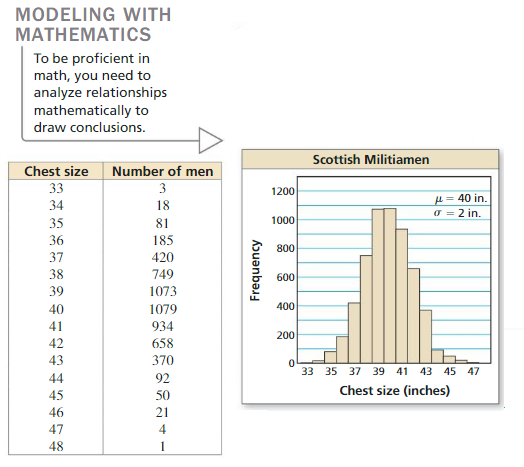
Work with a partner. In many naturally occurring data sets, the histogram of the data is bell-shaped. In statistics, such data sets are said to have a normal distribution. For the normal distribution shown below, estimate the percent of the data that lies within one, two, and three standard deviations of the mean. Each square on the grid represents 1%.

EXPLORATION 2

Analyzing a Data Set
Work with a partner. A famous data set was collected in Scotland in the mid-1800s. It contains the chest sizes (in inches) of 5738 men in the Scottish Militia. Do the data fit a normal distribution? Explain.

Communicate Your Answer

Question 3.
In a normal distribution, about what percent of the data lies within one, two, and three standard deviations of the mean?
Answer:

Question 4.
Use the Internet or some other reference to find another data set that is normally distributed. Display your data in a histogram.
Answer:

A normal distribution has mean μ and standard deviation σ. Find the indicated probability for a randomly selected x-value from the distribution.
Question 1.
P(x ≤ μ)
Answer:

Question 2.
P(x ≥ μ)
Answer:

Question 3.
P(μ ≤ x ≤ μ + 2σ)
Answer:

Question 4.
P(μ − σ ≤ x ≤ μ)
Answer:

Question 5.
P(x ≤ μ − 3σ)
Answer:

Question 6.
P(x ≥ μ + σ)
Answer:

Question 7.
WHAT IF?
In Example 2, about what percent of the people taking the test have scores between 43 and 79?
Answer:

Question 8.
WHAT IF?
In Example 3, what is the probability that the infant weighs 3990 grams or more?
Answer:

Question 9.
Explain why it makes sense that P(z ≤ 0) = 0.5.
Answer:

Question 10.
Determine whether the histogram has a normal distribution.

Answer:

Using Normal Distributions 11.1 Exercises

Vocabulary and Core Concept Check
Question 1.
WRITING
Describe how to use the standard normal table to find P(z ≤ 1.4).
Answer:

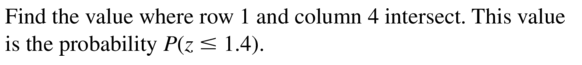
Question 2.
WHICH ONE DOESN’T BELONG?
Which histogram does not belong with the other three? Explain your reasoning.

Answer:

Monitoring Progress and Modeling with Mathematics

ATTENDING TO PRECISION In Exercises 3–6, give the percent of the area under the normal curve represented by the shaded region(s).
Question 3.

Answer:

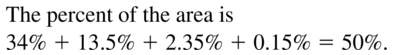
Question 4.

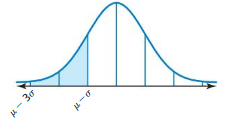
Answer:

Question 5.

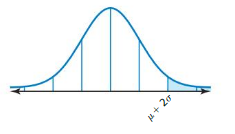
Answer:

Question 6.

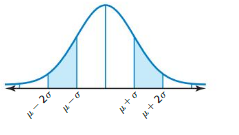
Answer:

In Exercises 7–12, a normal distribution has mean μ and standard deviation σ. Find the indicated probability for a randomly selected x-value from the distribution.
Question 7.
P(x ≤ μ − σ)
Answer:

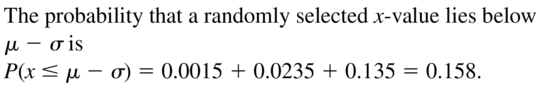
Question 8.
P(x ≥ μ − σ)
Answer:

Question 9.
P(x ≥ μ + 2σ)
Answer:

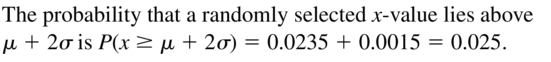
Question 10.
P(x ≤ μ + σ)
Answer:

Question 11.
P(μ − σ ≤ x ≤ μ + σ)
Answer:

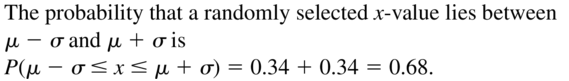
Question 12.
P(μ − 3σ ≤ x ≤ μ)
Answer:

In Exercises 13–18, a normal distribution has a mean of 33 and a standard deviation of 4. Find the probability that a randomly selected x-value from the distribution is in the given interval.
Question 13.
between 29 and 37
Answer:

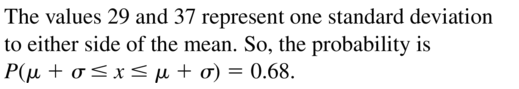
Question 14.
between 33 and 45
Answer:

Question 15.
at least 25
Answer:

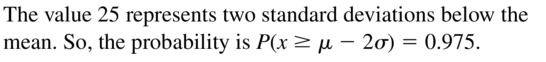
Question 16.
at least 29
Answer:

Question 17.
at most 37
Answer:

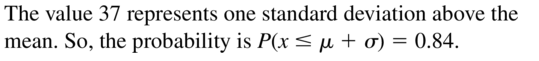
Question 18.
at most 21
Answer:

Question 19.
PROBLEM SOLVING
The wing lengths of houseflies are normally distributed with a mean of 4.6 millimeters and a standard deviation of 0.4 millimeter.

a. About what percent of houseflies have wing lengths between 3.8 millimeters and 5.0 millimeters?
b. About what percent of houseflies have wing lengths longer than 5.8 millimeters?
Answer:

Question 20.
PROBLEM SOLVING
The times a fire department takes to arrive at the scene of an emergency are normally distributed with a mean of 6 minutes and a standard deviation of 1 minute.
a. For about what percent of emergencies does the fire department arrive at the scene in 8 minutes or less?
b. The goal of the fire department is to reach the scene of an emergency in 5 minutes or less. About what percent of the time does the fire department achieve its goal?
Answer:

ERROR ANALYSIS In Exercises 21 and 22, a normal distribution has a mean of 25 and a standard deviation of 2. Describe and correct the error in finding the probability that a randomly selected x-value is in the given interval.
Question 21.
between 23 and 27

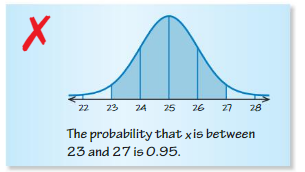
Answer:

Question 22.
at least 21

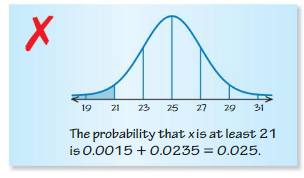
Answer:

Question 23.
PROBLEM SOLVING
A busy time to visit a bank is during its Friday evening rush hours. For these hours, the waiting times at the drive-through window are normally distributed with a mean of 8 minutes and a standard deviation of 2 minutes. You have no more than 11 minutes to do your banking and still make it to your meeting on time. What is the probability that you will be late for the meeting?
Answer:

Question 24.
PROBLEM SOLVING
Scientists conducted aerial surveys of a seal sanctuary and recorded the number x of seals they observed during each survey. The numbers of seals observed were normally distributed with a mean of 73 seals and a standard deviation of 14.1 seals. Find the probability that at most 50 seals were observed during a randomly chosen survey.

Answer:

In Exercises 25 and 26, determine whether the histogram has a normal distribution.
Question 25.

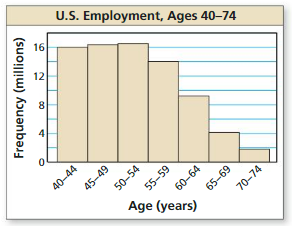
Answer:

Question 26.

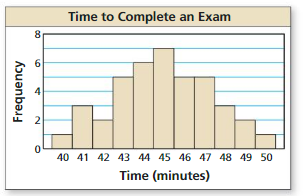
Answer:

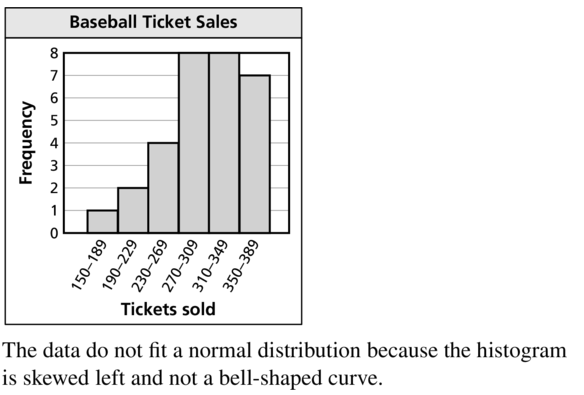
Question 27.
ANALYZING RELATIONSHIPS
The table shows the numbers of tickets that are sold for various baseball games in a league over an entire season. Display the data in a histogram. Do the data fit a normal distribution? Explain.

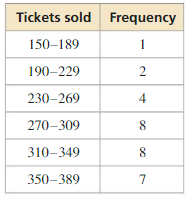
Answer:

Question 28.
PROBLEM SOLVING
The guayule plant, which grows in the southwestern United States and in Mexico, is one of several plants that can be used as a source of rubber. In a large group of guayule plants, the heights of the plants are normally distributed with a mean of 12 inches and a standard deviation of 2 inches.

a. What percent of the plants are taller than 16 inches?
b. What percent of the plants are at most 13 inches?
c. What percent of the plants are between 7 inches and 14 inches?
d. What percent of the plants are at least 3 inches taller than or at least 3 inches shorter than the mean height?
Answer:

Question 29.
REASONING
Boxes of cereal are filled by a machine. Tests show that the amount of cereal in each box varies. The weights are normally distributed with a mean of 20 ounces and a standard deviation of 0.25 ounce. Four boxes of cereal are randomly chosen.
a. What is the probability that all four boxes contain no more than 19.4 ounces of cereal?
b. Do you think the machine is functioning properly? Explain.
Answer:

Question 30.
THOUGHT PROVOKING
Sketch the graph of the standard normal distribution function, given by
f (x) = \(\frac{1}{\sqrt{2} \pi}\)e^−x2/2.
Estimate the area of the region bounded by the x-axis, the graph of f, and the vertical lines x =−3 and x = 3.
Answer:

Question 31.
REASONING
For normally distributed data, describe the value that represents the 84th percentile in terms of the mean and standard deviation.
Answer:

Question 32.
HOW DO YOU SEE IT?
In the figure, the shaded region represents 47.5% of the area under a normal curve. What are the mean and standard deviation of the normal distribution?

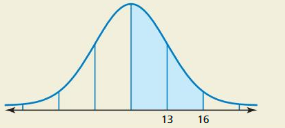
Answer:

Question 33.
DRAWING CONCLUSIONS
You take both the SAT (Scholastic Aptitude Test) and the ACT (American College Test). You score 650 on the mathematics section of the SAT and 29 on the mathematics section of the ACT. The SAT test scores and the ACT test scores are each normally distributed. For the SAT, the mean is 514 and the standard deviation is 118. For the ACT, the mean is 21.0 and the standard deviation is 5.3.
a. What percentile is your SAT math score?
b. What percentile is your ACT math score?c. On which test did you perform better? Explain your reasoning.
Answer:

Question 34.
WRITING
Explain how you can convert ACT scores into corresponding SAT scores when you know the mean and standard deviation of each distribution.
Answer:

Question 35.
MAKING AN ARGUMENT
A data set has a median of 80 and a mean of 90. Your friend claims that the distribution of the data is skewed left. Is your friend correct? Explain your reasoning.
Answer:

Question 36.
CRITICAL THINKING
The average scores on a statistics test are normally distributed with a mean of 75 and a standard deviation of 10. You randomly select a test score x. Find P (∣x−μ∣≥ 15 ).
Answer:

Maintaining Mathematical Proficiency

Graph the function. Identify the x-intercepts and the points where the local maximums and local minimums occur. Determine the intervals for which the function is increasing or decreasing.
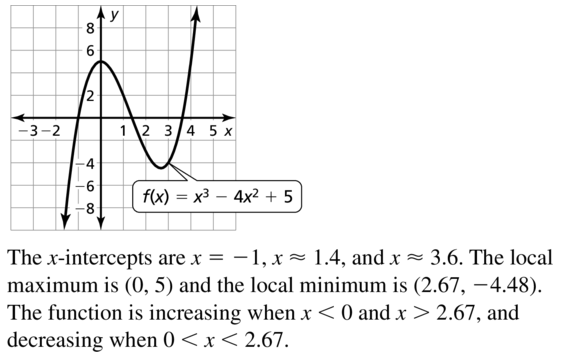
Question 37.
f(x) = x³ − 4x²+ 5
Answer:

Question 38.
g(x) = \(\frac{1}{4}\)x⁴ − 2x²−x − 3
Answer:

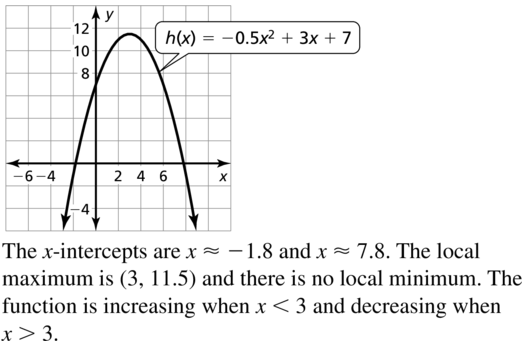
Question 39.
h(x) = −0.5x²+ 3x + 7
Answer:

Question 40.
f(x) = −x⁴ + 6x² − 13
Answer:

Lesson 11.2 Populations, Samples, and Hypotheses

Essential Question How can you test theoretical probability using sample data?

EXPLORATION 1

Using Sample Data
Work with a partner.
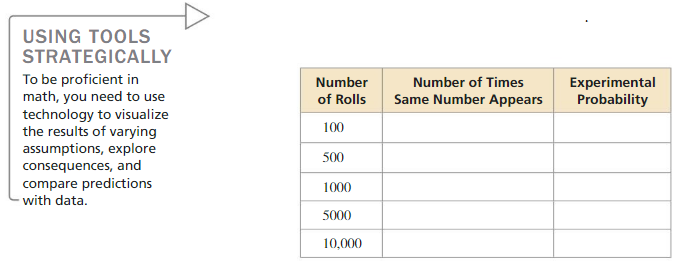
a. When two six-sided dice are rolled, what is the theoretical probability that you roll the same number on both dice?

b. Conduct an experiment to check your answer in part (a). What sample size did you use? Explain your reasoning.
c. Use the dice rolling simulator at BigIdeasMath.com to complete the table. Do your experimental data check the theoretical probability you found in part (a)? Explain. What happens as you increase the sample size?

EXPLORATION 2

Using Sample Data
Work with a partner.
a. When three six-sided dice are rolled, what is the theoretical probability that you roll the same number on all three dice?

b. Compare the theoretical probability you found in part (a) with the theoretical probability you found in Exploration 1(a).
c. Conduct an experiment to check your answer in part (a). How does adding a die affect the sample size that you use? Explain your reasoning.
d. Use the dice rolling simulator at BigIdeasMath.com to check your answer to part (a). What happens as you increase the sample size?

Communicate Your Answer

Question 3.
How can you test theoretical probability using sample data?
Answer:

Question 4.
Conduct an experiment to determine the probability of rolling a sum of 7 when two six-sided dice are rolled. Then find the theoretical probability and compare your answers.
Answer:

Monitoring Progress

In Monitoring Progress Questions 1 and 2, identify the population and the sample.
Question 1.
To estimate the retail prices for three grades of gasoline sold in the United States, the Energy Information Association calls 800 retail gasoline outlets, records the prices, and then determines the average price for each grade.
Answer:

Question 2.
A survey of 4464 shoppers in the United States found that they spent an average of $407.02 from Thursday through Sunday during a recent Thanksgiving holiday.
Answer:

Question 3.
A survey found that the median salary of 1068 statisticians is about $72,800. Is the median salary a parameter or a statistic? Explain your reasoning.
Answer:

Question 4.
The mean age of U.S. representatives at the start of the 113th Congress was about 57 years. Is the mean age a parameter or a statistic? Explain your reasoning.
Answer:

Question 5.
WHAT IF?
In Example 3, what should you conclude when you roll the actual die 50 times and get (a) 24 odd numbers and (b) 31 odd numbers?
Answer:

Populations, Samples, and Hypotheses 11.2 Exercises

Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
A portion of a population that can be studied in order to make predictions about the entire population is a(n) ___________.
Answer:

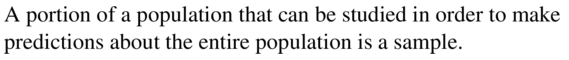
Question 2.
WRITING
Describe the difference between a parameter and a statistic. Give an example of each.
Answer:

Question 3.
VOCABULARY
What is a hypothesis in statistics?
Answer:

Question 4.
WRITING
Describe two ways you can make an incorrect decision when analyzing a hypothesis.
Answer:

Monitoring Progress and Modeling with Mathematics

In Exercises 5–8, determine whether the data are collected from a population or a sample. Explain your reasoning.
Question 5.
the number of high school students in the United States
Answer:

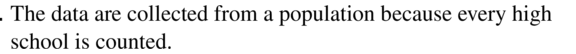
Question 6.
the color of every third car that passes your house
Answer:

Question 7.
a survey of 100 spectators at a sporting event with 1800 spectators
Answer:

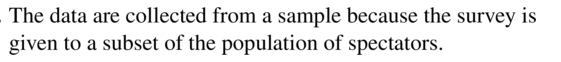
Question 8.
the age of each dentist in the United States
Answer:

In Exercises 9–12, identify the population and sample. Describe the sample.
Question 9.
In the United States, a survey of 1152 adults ages 18 and over found that 403 of them pretend to use their smartphones to avoid talking to someone.

Answer:

Question 10.
In the United States, a survey of 1777 adults ages 18 and over found that 1279 of them do some kind of spring cleaning every year.
Answer:

Question 11.
In a school district, a survey of 1300 high school students found that 1001 of them like the new, healthy cafeteria food choices.
Answer:

Question 12.
In the United States, a survey of 2000 households with at least one child found that 1280 of them eat dinner together every night.

Answer:

In Exercises 13–16, determine whether the numerical value is a parameter or a statistic. Explain your reasoning.
Question 13.
The average annual salary of some physical therapists in a state is $76,210.

Answer:

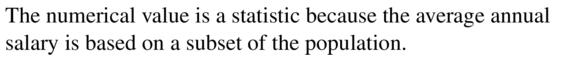
Question 14.
In a recent year, 53% of the senators in the United States Senate were Democrats.
Answer:

Question 15.
Seventy-three percent of all the students in a school would prefer to have school dances on Saturday.
Answer:

Question 16.
A survey of U.S. adults found that 10% believe a cleaning product they use is not safe for the environment.
Answer:

Question 17.
ERROR ANALYSIS
A survey of 1270 high school students found that 965 students felt added stress because of their workload. Describe and correct the error in identifying the population and the sample.

Answer:

Question 18.
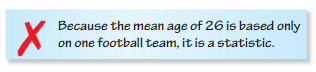
ERROR ANALYSIS
Of all the players on a National Football League team, the mean age is 26 years. Describe and correct the error in determining whether the mean age represents a parameter or statistic. Because the mean age of 26 is based only on one football team, it is a statistic.

Answer:

Question 19.
MODELING WITH MATHEMATICS
You flip a coin 4 times and do not get a tails. You suspect this coin favors heads. The coin maker claims that the coin doesnot favor heads or tails. You simulate flipping the coin 50 times by repeatedly drawing 200 random samples of size 50. The histogram shows the results. What should you conclude when you flip the actual coin 50 times and get (a) 27 heads and (b) 33 heads?

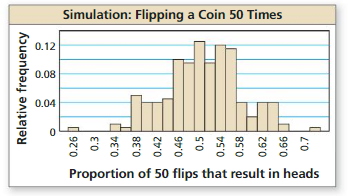
Answer:

Question 20.
MODELING WITH MATHEMATICS
Use the histogram in Exercise 19 to determine what you should conclude when you flip the actual coin 50 times and get (a) 17 heads and (b) 23 heads.
Answer:

Question 21.
MAKING AN ARGUMENT
A random sample of five people at a movie theater from a population of 200 people gave the film 4 out of 4 stars. Your friend concludes that everyone in the movie theater would give the film 4 stars. Is your friend correct? Explain your reasoning.
Answer:

Question 22.
HOW DO YOU SEE IT?
Use the Venn diagram to identify the population and sample. Explain your reasoning.

Answer:

Question 23.
OPEN-ENDED
Find a newspaper or magazine article that describes a survey. Identify the population and sample. Describe the sample.
Answer:

Question 24.
THOUGHT PROVOKING
You choose a random sample of 200 from a population of 2000. Each person in the sample is asked how many hours of sleep he or she gets each night. The mean of your sample is 8 hours. Is it possible that the mean of the entire population is only 7.5 hours of sleep each night? Explain.
Answer:

Question 25.
DRAWING CONCLUSIONS
You perform two simulations of repeatedly selecting a marble out of a bag with replacement that contains three red marbles and three blue marbles. The first simulation uses 20 random samples of size 10, and the second uses 400 random samples of size 10. The histograms show the results. Which simulation should you use to accurately analyze a hypothesis? Explain.

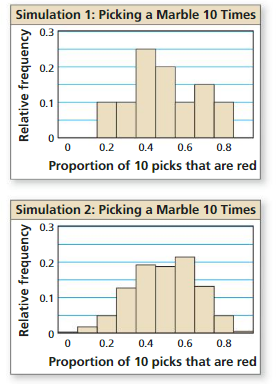
Answer:

Question 26.
PROBLEM SOLVING
You roll an eight-sided die five times and get a four every time. You suspect that the die favors the number four. The die maker claims that the die does not favor any number.
a. Perform a simulation involving 50 trials of rolling the actual die and getting a four to test the die maker’s claim. Display the results in a histogram.
b. What should you conclude when you roll the actual die 50 times and get 20 fours? 7 fours?
Answer:

Maintaining Mathematical Proficiency

Solve the equation by completing the square.
Question 27.
x²− 10x − 4 = 0
Answer:

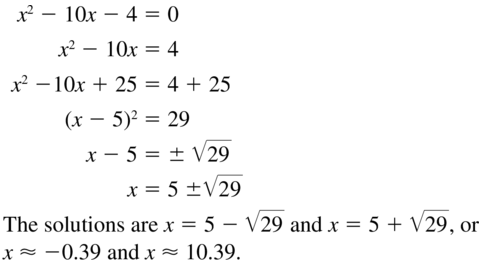
Question 28.
3t² + 6t = 18
Answer:

Question 29.
s² + 10s + 8 = 0
Answer:

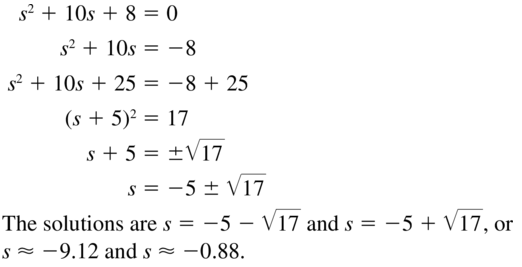
Solve the equation using the Quadratic Formula.
Question 30.
n²+ 2n + 2 = 0
Answer:

Question 31.
4z² + 28z = 15
Answer:

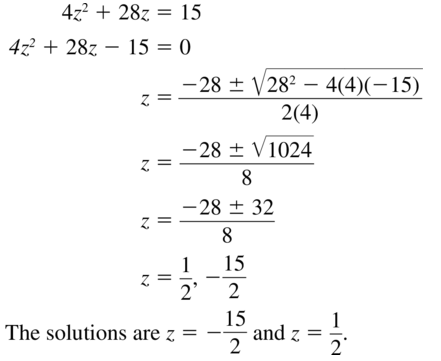
Question 32.
5w − w² = −11
Answer:

Lesson 11.3 Collecting Data

Essential Question What are some considerations when undertaking a statistical study? The goal of any statistical study is to collect data and then use the data to make a decision. Any decision you make using the results of a statistical study is only as reliable as the process used to obtain the data. If the process is flawed, then the resulting decision is questionable.

EXPLORATION 1

Analyzing Sampling Techniques
Work with a partner. Determine whether each sample is representative of the population. Explain your reasoning.

a. To determine the number of hours people exercise during a week, researchers use random-digit dialing and call 1500 people.
b. To determine how many text messages high school students send in a week, researchers post a survey on a website and receive 750 responses.
c. To determine how much money college students spend on clothes each semester,a researcher surveys 450 college students as they leave the university library.
d. To determine the quality of service customers receive, an airline sends an e-mail survey to each customer after the completion of a flight.

EXPLORATION 2

Analyzing Survey Questions
Work with a partner. Determine whether each survey question is biased. Explain your reasoning. If so, suggest an unbiased rewording of the question.
a. Does eating nutritious, whole-grain foods improve your health?
b. Do you ever attempt the dangerous activity of texting while driving?
c. How many hours do you sleep each night?
d. How can the mayor of your city improve his or her public image?

EXPLORATION 3

Analyzing Survey Randomness and Truthfulness
Work with a partner. Discuss each potential problem in obtaining a random survey of a population. Include suggestions for overcoming the problem.
a. The people selected might not be a random sample of the population.
b. The people selected might not be willing to participate in the survey.
c. The people selected might not be truthful when answering the question.
d. The people selected might not understand the survey question.

Communicate Your Answer

Question 4.
What are some considerations when undertaking a statistical study?
Answer:

Question 5.
Find a real-life example of a biased survey question. Then suggest an unbiased rewording of the question.
Answer:

Monitoring Progress

Question 1.
WHAT IF?
In Example 1, you divide the students in your school according to their zip codes, then select all of the students that live in one zip code. What type of sample are you using?
Answer:

Question 2.
Describe another method you can use to obtain a stratified sample in Example 1.
Answer:

Question 3.
The manager of a concert hall wants to know how often people in the community attend concerts. The manager asks 45 people standing in line for a rock concert how many concerts they attend per year. Identify the type of sample the manager is using and explain why the sample is biased.
Answer:

Question 4.
In Example 3, what is another method you can use to generate a random sample of 50 students? Explain why your sampling method is random.
Answer:

Identify the method of data collection the situation describes.
Question 5.
Members of a student council at your school ask every eighth student who enters the cafeteria whether they like the snacks in the school’s vending machines.
Answer:

Question 6.
A park ranger measures and records the heights of trees in a park as they grow.
Answer:

Question 7.
A researcher uses a computer program to help determine how fast an influenza virus might spread within a city.
Answer:

Question 8.
Explain why the survey question below may be biased or otherwise introduce bias into the survey. Then describe a way to correct the flaw.
“Do you agree that our school cafeteria should switch to a healthier menu?”
Answer:

Collecting Data 11.3 Exercises

Vocabulary and Core Concept Check
Question 1.
VOCABULARY
Describe the difference between a stratified sample and a cluster sample.
Answer:

Question 2.
COMPLETE THE SENTENCE
A sample for which each member of a population has an equal chance of being selected is a(n) __________ sample.
Answer:

Question 3.
WRITING
Describe a situation in which you would use a simulation to collect data.
Answer:

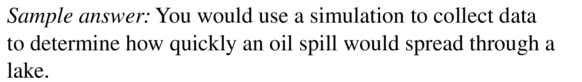
Question 4.
WRITING
Describe the difference between an unbiased sample and a biased sample. Give one example of each.
Answer:

Monitoring Progress and Modeling with Mathematics

In Exercises 5–8, identify the type of sample described.
Question 5.
The owners of a chain of 260 retail stores want to assess employee job satisfaction. Employees from 12 stores near the headquarters are surveyed.
Answer:

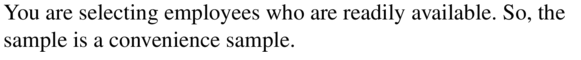
Question 6.
Each employee in a company writes their name on a card and places it in a hat. The employees whose names are on the first two cards drawn each win a gift card.
Answer:

Question 7.
A taxicab company wants to know whether its customers are satisfied with the service. Drivers survey every tenth customer during the day.
Answer:

Question 8.
The owner of a community pool wants to ask patrons whether they think the water should be colder. Patrons are divided into four age groups, and a sample is randomly surveyed from each age group.
Answer:

In Exercises 9–12, identify the type of sample and explain why the sample is biased.
Question 9.
A town council wants to know whether residents support having an off-leash area for dogs in the town park. Eighty dog owners are surveyed at the park.

Answer:

Question 10.
A sportswriter wants to determine whether baseball coaches think wooden bats should be mandatory in collegiate baseball. The sportswriter mails surveys to all collegiate coaches and uses the surveys that are returned.
Answer:

Question 11.
You want to find out whether booth holders at a convention were pleased with their booth locations. You divide the convention center into six sections and survey every booth holder in the fifth section.
Answer:

Question 12.
Every tenth employee who arrives at a company health fair answers a survey that asks for opinions about new health-related programs.
Answer:

Question 13.
ERROR ANALYSIS
Surveys are mailed to every other household in a neighborhood. Each survey that is returned is used. Describe and correct the error in identifying the type of sample that is used.

Answer:

Question 14.
ERROR ANALYSIS
A researcher wants to know whether the U.S. workforce supports raising the minimum wage. Fifty high school students chosen at random are surveyed. Describe and correct the error in determining whether the sample is biased.

Answer:

In Exercises 15–18, determine whether the sample is biased. Explain your reasoning.
Question 15.
Every third person who enters an athletic event is asked whether he or she supports the use of instant replay in officiating the event.
Answer:

Question 16.
A governor wants to know whether voters in the state support building a highway that will pass through a state forest. Business owners in a town near the proposed highway are randomly surveyed.
Answer:

Question 17.
To assess customers’ experiences making purchases online, a rating company e-mails purchasers and asks that they click on a link and complete a survey.
Answer:

Question 18.
Your school principal randomly selects five students from each grade to complete a survey about classroom participation.
Answer:

Question 19.
WRITING
The staff of a student newsletter wants to conduct a survey of the students’ favorite television shows. There are 1225 students in the school. Describe a method for selecting a random sample of 250 students to survey.

Answer:

Question 20.
WRITING
A national collegiate athletic association wants to survey 15 of the 120 head football coaches in a division about a proposed rules change. Describe a method for selecting a random sample of coaches to survey.
Answer:

In Exercises 21–24, identify the method of data collection the situation describes.
Question 21.
A researcher uses technology to estimate the damage that will be done if a volcano erupts.

Answer:

Question 22.
The owner of a restaurant asks 20 customers whether they are satisfied with the quality of their meals.
Answer:

Question 23.
A researcher compares incomes of people who live in rural areas with those who live in large urban areas.
Answer:

Question 24.
A researcher places bacteria samples in two different climates. The researcher then measures the bacteria growth in each sample after 3 days.
Answer:

In Exercises 25–28, explain why the survey question may be biased or otherwise introduce bias into the survey. Then describe a way to correct the flaw.
Question 25.
“Do you agree that the budget of our city should be cut?”
Answer:

Question 26.
“Would you rather watch the latest award-winning movie or just read some book?”
Answer:

Question 27.
“The tap water coming from our western water supply contains twice the level of arsenic of water from our eastern supply. Do you think the government should address this health problem?”
Answer:

Question 28.
A child asks, “Do you support the construction of a new children’s hospital?”
Answer:

In Exercises 29–32, determine whether the survey question may be biased or otherwise introduce bias into the survey. Explain your reasoning.
Question 29.
“Do you favor government funding to help prevent acid rain?”
Answer:

Question 30.
“Do you think that renovating the old town hall would be a mistake?”
Answer:

Question 31.
A police officer asks mall visitors, “Do you wear your seat belt regularly?”
Answer:

Question 32.
“Do you agree with the amendments to the Clean Air Act?”
Answer:

Question 33.
REASONING
A researcher studies the effect of fiber supplements on heart disease. The researcher identified 175 people who take fiber supplements and 175 people who do not take fiber supplements. The study found that those who took the supplements had 19.6% fewer heart attacks. The researcher concludes that taking fiber supplements reduces the chance of heart attacks.

a. Explain why the researcher’s conclusion may not be valid.
b. Describe how the researcher could have conducted the study differently to produce valid results.
Answer:

Question 34.
HOW DO YOU SEE IT?
A poll is conducted to predict the results of a statewide election in New Mexico before all the votes are counted. Fifty voters in each of the state’s 33 counties are asked how they voted as they leave the polls.
a. Identify the type of sample described.
b. Explain how the diagram shows that the polling method could result in a biased sample.

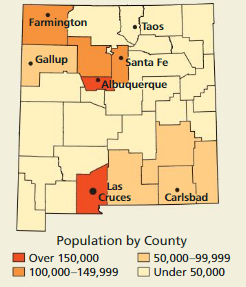
Answer:

Question 35.
WRITING
Consider each type of sample listed on page 610. Which of the samples are most likely to lead to biased results? Explain.
Answer:

Question 36.
THOUGHT PROVOKING
What is the difference between a “blind experiment” and a “double-blind experiment?” Describe a possible advantage of the second type of experiment over the first.
Answer:

Question 37.
WRITING
A college wants to survey its graduating seniors to find out how many have already found jobs in their field of study after graduation.
a. What is the objective of the survey?
b. Describe the population for the survey.
c. Write two unbiased questions for the survey.
Answer:

Question 38.
REASONING
About 3.2% of U.S. adults follow a vegetarian-based diet. Two randomly selected groups of people were asked whether they follow such a diet. The first sample consists of 20 people and the second sample consists of 200 people. Which sample proportion is more likely to be representative of the national percentage? Explain.
Answer:

Question 39.
MAKING AN ARGUMENT
The U.S. Census is taken every10 years to gather data from the population. Your friend claims that the sample cannot be biased. Is your friend correct? Explain.
Answer:

Question 40.
OPEN-ENDED
An airline wants to know whether travelers have enough leg room on its planes.

a. What method of data collection is appropriate for this situation?
b. Describe a sampling method that is likely to give biased results. Explain.
c. Describe a sampling method that is not likely to give biased results. Explain.
d. Write one biased question and one unbiased question for this situation.
Answer:

Question 41.
REASONING
A website contains a link to a survey that asks how much time each person spends on the Internet each week.
a. What type of sampling method is used in this situation?
b. Which population is likely to respond to the survey? What can you conclude?
Answer:

Maintaining Mathematical Proficiency

Evaluate the expression without using a calculator.
Question 42.
4^5/2
Answer:

Question 43.
27^2/3
Answer:

Question 44.
−64^1/3
Answer:

Question 45.
8^-2/3
Answer:

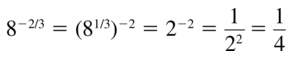
Simplify the expression.
Question 46.
(4^3/2 • 4^1/4)⁴
Answer:

Question 47.
(6^1/3 • 3^1/3)^-2
Answer:

Question 48.
\(\sqrt [ 3 ]{ 4 }\) • \(\sqrt [ 3 ]{ 16 }\)
Answer:

Question 49.
\(\frac{\sqrt[4]{405}}{\sqrt[4]{5}}\)
Answer:

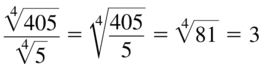
Data Analysis and Statistics Study Skills: Reworking Your Notes

11.1–11.3 What Did You Learn?

Core Vocabulary

Core Concepts

Mathematical Practices

Question 1.
What previously established results, if any, did you use to solve Exercise 31 on page 602?
Answer:

Question 2.
What external resources, if any, did you use to answer Exercise 36 on page 616?
Answer:

Study Skills: Reworking Your Notes

It’s almost impossible to write down in your notes all the detailed information you are taught in class. A good way to reinforce the concepts and put them into your long-term memory is to rework your notes. When you take notes, leave extra space on the pages. You can go back after class and fill in:

• important definitions and rules
• additional examples
• questions you have about the material
  

Data Analysis and Statistics 11.1–11.3 Quiz

A normal distribution has a mean of 32 and a standard deviation of 4. Find the probability that a randomly selected x-value from the distribution is in the given interval.
Question 1.
at least 28
Answer:

Question 2.
between 20 and 32
Answer:

Question 3.
at most 26
Answer:

Question 4.
at most 35
Answer:

Determine whether the histogram has a normal distribution.
Question 5.

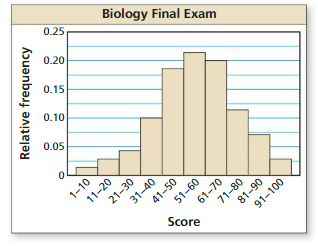
Answer:

Question 6.

Answer:

Question 7.
A survey of 1654 high school seniors determined that 1125 plan to attend college. Identify the population and the sample. Describe the sample.
Answer:

Question 8.
A survey of all employees at a company found that the mean one-way daily commute to work of the employees is 25.5 minutes. Is the mean time a parameter or a statistic? Explain your reasoning.
Answer:

Question 9.
A researcher records the number of bacteria present in several samples in a laboratory. Identify the method of data collection.
Answer:

Question 10.
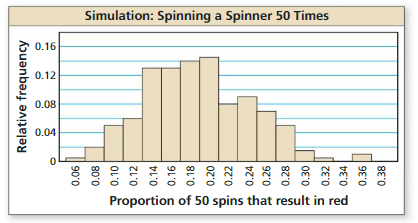
You spin a five-color spinner, which is divided into equal parts, five times and every time the spinner lands on red. You suspect the spinner favors red. The maker of the spinner claims that the spinner does not favor any color. You simulate spinning the spinner 50 times by repeatedly drawing 200 random samples of size 50. The histogram shows the results. Use the histogram to determine what you should conclude when you spin the actual spinner 50 times and the spinner lands on red (a) 9 times and (b) 19 times.

Answer:

Question 11.
A local television station wants to find the number of hours per week people in the viewing area watch sporting events on television. The station surveys people at a nearby sports stadium.
a. Identify the type of sample described.
b. Is the sample biased? Explain your reasoning.
c. Describe a method for selecting a random sample of 200 people to survey.
Answer:

Lesson 11.4 Experimental Design

Essential Question How can you use an experiment to test a conjecture?

EXPLORATION 1

Using an Experiment
Work with a partner. Standard white playing dice are manufactured with black dots that are indentations, as shown. So, the side with six indentations is the lightest side and the side with one indentation is the heaviest side.

You make a conjecture that when you roll a standard playing die, the number 6 will come up more often than the number 1 because 6 is the lightest side. To test your conjecture, roll a standard playing die 25 times. Record the results in the table. Does the experiment confirm your conjecture? Explain your reasoning.

EXPLORATION 2

Analyzing an Experiment
Work with a partner. To overcome the imbalance of standard playing dice, one of the authors of this book invented and patented 12-sided dice, on which each number from 1 through 6 appears twice (on opposing sides). See BigIdeasMath.com.
As part of the patent process, a standard playing die was rolled 27,090 times. The results are shown below.

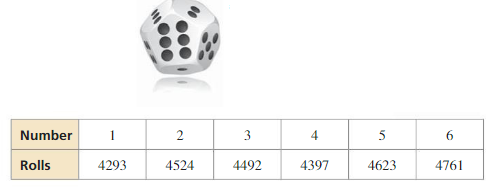
What can you conclude from the results of this experiment? Explain your reasoning.

Communicate Your Answer

Question 3.
How can you use an experiment to test a conjecture?
Answer:

Question 4.
Exploration 2 shows the results of rolling a standard playing die 27,090 times to test the conjecture in Exploration 1. Why do you think the number of trials was so large?
Answer:

Question 5.
Make a conjecture about the outcomes of rolling the 12-sided die in Exploration 2. Then use the Internet to find a 12-sided die rolling simulator. Use the simulator to complete a table similar to the one shown below. How many times did you simulate rolling the die? Explain your reasoning.

Answer:

Monitoring Progress
Question 1.
Determine whether the study is a randomized comparative experiment. If it is, describe the treatment, the treatment group, and the control group. If it is not, explain why not and discuss whether the conclusions drawn from the study are valid.

Answer:

Question 2.
Determine whether the following research topic is best investigated through an experiment or an observational study. Then describe the design of the experiment or observational study.
You want to know whether flowers sprayed twice per day with a mist of water stay fresh longer than flowers that are not sprayed.
Answer:

Question 3.
In Example 3, the company identifies 250 people who are overweight. The subjects are randomly assigned to a treatment group or a control group. In addition, each subject is given a DVD that documents the dangers of obesity. After 3 months, most of the subjects placed in the treatment group have lost weight. Identify a potential problem with the experimental design. Then describe how you can improve it.
Answer:

Question 4.
You design an experiment to test the effectiveness of a vaccine against a strain of influenza. In the experiment, 100,000 people receive the vaccine and another 100,000 people receive a placebo. Identify a potential problem with the experimental design. Then describe how you can improve it.
Answer:

Experimental Design 11.4 Exercises

Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
Repetition of an experiment under the same or similar conditions is called _________.
Answer:

Question 2.
WRITING
Describe the difference between the control group and the treatment group in a controlled experiment.
Answer:

Monitoring Progress and Modeling with Mathematics

In Exercises 3 and 4, determine whether the study is a randomized comparative experiment. If it is, describe the treatment, the treatment group, and the control group. If it is not, explain why not and discuss whether the conclusions drawn from the study are valid.
Question 3.

Answer:

Question 4.

Answer:

ERROR ANALYSIS In Exercises 5 and 6, describe and correct the error in describing the study.

A company’s researchers want to study the effects of adding shea butter to their existing hair conditioner. They monitor the hair quality of 30 randomly selected customers using the regular conditioner and 30 randomly selected customers using the new shea butter conditioner.
Question 5.

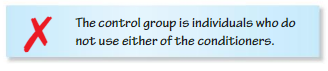
Answer:

Question 6.

Answer:

In Exercises 7–10, explain whether the research topic is best investigated through an experiment or an observational study. Then describe the design of the experiment or observational study.
Question 7.
A researcher wants to compare the body mass index of smokers and nonsmokers.
Answer:

Question 8.
A restaurant chef wants to know which pasta sauce recipe is preferred by more diners.
Answer:

Question 9.
A farmer wants to know whether a new fertilizer affects the weight of the fruit produced by strawberry plants.
Answer:

Question 10.
You want to know whether homes that are close to parks or schools have higher property values.
Answer:

Question 11.
DRAWING CONCLUSIONS
A company wants to test whether a nutritional supplement has an adverse effect on an athlete’s heart rate while exercising. Identify a potential problem, if any, with each experimental design. Then describe how you can improve it.
a. The company randomly selects 250 athletes. Half of the athletes receive the supplement and their heart rates are monitored while they run on a treadmill. The other half of the athletes are given a placebo and their heart rates are monitored while they lift weights. The heart rates of the athletes who took the supplement significantly increased while exercising.

b. The company selects 1000 athletes. The athletes are divided into two groups based on age. Within each age group, the athletes are randomly assigned to receive the supplement or the placebo. The athletes’ heart rates are monitored while they run on a treadmill. There was no significant difference in the increases in heart rates between the two groups.
Answer:

Question 12.
DRAWING CONCLUSIONS
A researcher wants to test the effectiveness of reading novels on raising intelligence quotient (IQ) scores. Identify a potential problem, if any, with each experimental design. Then describe how you can improve it.
a. The researcher selects 500 adults and randomly divides them into two groups. One group reads novels daily and one group does not read novels. At the end of 1 year, each adult is evaluated and it is determined that neither group had an increase in IQ scores.

b. Fifty adults volunteer to spend time reading novels every day for 1 year. Fifty other adults volunteer to refrain from reading novels for 1 year. Each adult is evaluated and it is determined that the adults who read novels raised their IQ scores by 3 points more than the other group.

Answer:

Question 13.
DRAWING CONCLUSIONS
A fitness company claims that its workout program will increase vertical jump heights in 6 weeks. To test the workout program, 10 athletes are divided into two groups. The double bar graph shows the results of the experiment. Identify the potential problems with the experimental design. Then describe how you can improve it.

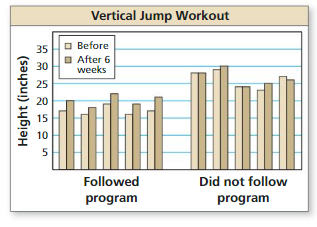
Answer:

Question 14.
WRITING
Explain why observational studies, rather than experiments, are usually used in astronomy.
Answer:

Question 15.
MAKING AN ARGUMENT
Your friend wants to determine whether the number of siblings has an effect on a student’s grades. Your friend claims to be able to show causality between the number of siblings and grades. Is your friend correct? Explain.
Answer:

Question 16.
HOW DO YOU SEE IT?
To test the effect political advertisements have on voter preferences, a researcher selects 400 potential voters and randomly divides them into two groups. The circle graphs show the results of the study.

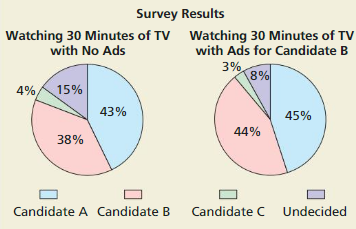
a. Is the study a randomized comparative experiment? Explain.
b. Describe the treatment.
c. Can you conclude that the political advertisements were effective? Explain.
Answer:

Question 17.
WRITING
Describe the placebo effect and how it affects the results of an experiment. Explain how a researcher can minimize the placebo effect.
Answer:

Question 18.
THOUGHT PROVOKING
Make a hypothesis about something that interests you. Design an experiment that could show that your hypothesis is probably true.
Answer:

Question 19.
REASONING
Will replicating an experiment on many individuals produce data that are more likely to accurately represent a population than performing the experiment only once? Explain.
Answer:

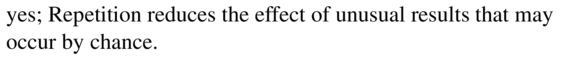
Maintaining Mathematical Proficiency

Draw a dot plot that represents the data. Identify the shape of the distribution.
Question 20.
Ages: 24, 21, 22, 26, 22, 23, 25, 23, 23, 24, 20, 25
Answer:

Question 21.
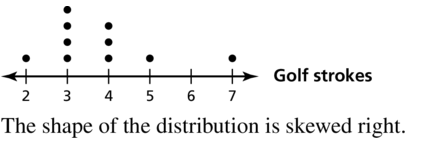
Golf strokes: 4, 3, 4, 3, 3, 2, 7, 5, 3, 4
Answer:

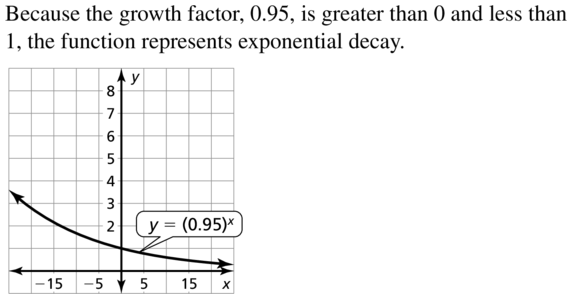
Tell whether the function represents exponential growth or exponential decay. Then graph the function.
Question 22.
y = 4^x
Answer:

Question 23.
y = (0.95)^x
Answer:

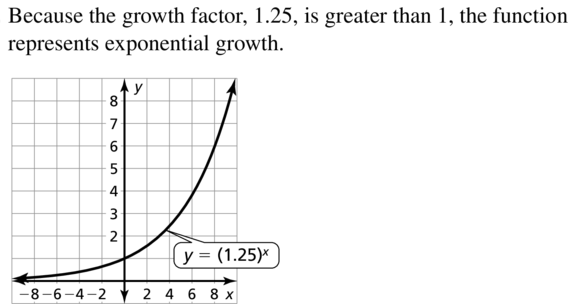
Question 24.
y = (0.2)^x
Answer:

Question 25.
y = (1.25)^x
Answer:

Lesson 11.5 Making Inferences from Sample Surveys

Essential Question How can you use a sample survey to infer a conclusion about a population?

EXPLORATION 1

Making an Inference from a Sample
Work with a partner. You conduct a study to determine what percent of the high school students in your city would prefer an upgraded model of their current cell phone. Based on your intuition and talking with a few acquaintances, you think that 50% of high school students would prefer an upgrade. You survey50 randomly chosen high school students and find that 20 of them prefer an upgraded model.

a. Based on your sample survey, what percent of the high school students in your city would prefer an upgraded model? Explain your reasoning.
b. In spite of your sample survey, is it still possible that 50% of the high school students in your city prefer an upgraded model? Explain your reasoning.

c. To investigate the likelihood that you could have selected a sample of 50 from a population in which 50% of the population does prefer an upgraded model, you create a binomial distribution as shown below. From the distribution, estimate the probability that exactly 20 students surveyed prefer an upgraded model. Is this event likely to occur? Explain your reasoning.

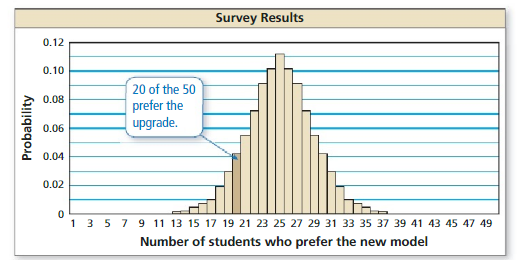
d. When making inferences from sample surveys, the sample must be random. In the situation described above, describe how you could design and conduct a survey using a random sample of 50 high school students who live in a large city.

Communicate Your Answer

Question 2.
How can you use a sample survey to infer a conclusion about a population?
Answer:

Question 3.
In Exploration 1(c), what is the probability that exactly 25 students you survey prefer an upgraded model?
Answer:

Monitoring Progress

Question 1.
The data from another random sample of 30 teen users of the social networking website are shown in the table. Estimate the population mean μ.

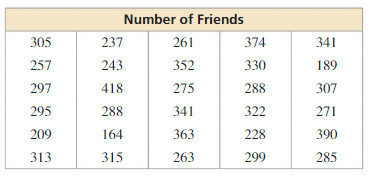
Answer:

Question 2.
Two candidates are running for class president. The table shows the results of four surveys of random students in the class. The students were asked whether they will vote for the incumbent. Do you think the incumbent will be reelected? Explain.

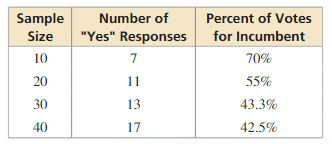
Answer:

Question 3.
WHAT IF?
In Example 3, what can you conclude about the accuracy of the claim that the population proportion is 0.34 when 21 adults in your random sample say mathematics is the most valuable subject?
Answer:

Question 4.
In a survey of 1028 people in the U.S., 87% reported using the Internet. Give an interval that is likely to contain the exact percent of all people in the U.S. who use the Internet.
Answer:

Making Inferences from Sample Surveys 11.5 Exercises

Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
The ___________ gives a limit on how much the responses of the sample would differ from the responses of the population.
Answer:

Question 2.
WRITING
What is the difference between descriptive and inferential statistics?
Answer:

Monitoring Progress and Modeling with Mathematics

Question 3.
PROBLEM SOLVING
The numbers of text messages sent each day by a random sample of 30 teen cellphone users are shown in the table. Estimate the population mean μ.

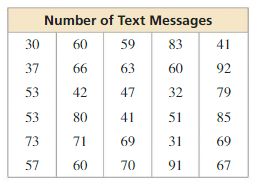
Answer:

Question 4.
PROBLEM SOLVING
The incomes for a random sample of 35 U.S. households are shown in the table. Estimate the population mean μ.

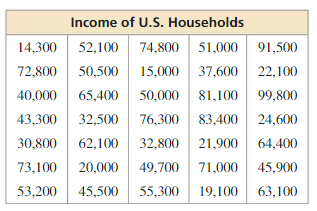
Answer:

Question 5.
PROBLEM SOLVING
Use the data in Exercise 3 to answer each question.
a. Estimate the population proportion ρ of teen cellphone users who send more than 70 text messages each day.
b. Estimate the population proportion ρ of teen cellphone users who send fewer than 50 text messages each day.
Answer:

Question 6.
WRITING
A survey asks a random sample of U.S. teenagers how many hours of television they watch each night. The survey reveals that the sample mean is 3 hours per night. How condent are you that the average of all U.S. teenagers is exactly 3 hours per night? Explain your reasoning.

Answer:

Question 7.
DRAWING CONCLUSIONS
When the President of the United States vetoes a bill, the Congress can override the veto by a two-thirds majority vote in each House. Five news organizations conduct individual random surveys of U.S. Senators. The senators are asked whether they will vote to override the veto. The results are shown in the table.

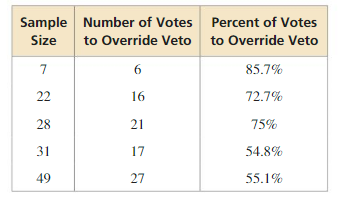
a. Based on the results of the first two surveys, do you think the Senate will vote to override the veto? Explain.
b. Based on the results in the table, do you think the Senate will vote to override the veto? Explain.
Answer:

Question 8.
DRAWING CONCLUSIONS
Your teacher lets the students decide whether to have their test on Friday or Monday. The table shows the results from four surveys of randomly selected students in your grade who are taking the same class. The students are asked whether they want to have the test on Friday.

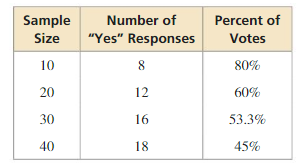
a. Based on the results of the first two surveys, do you think the test will be on Friday? Explain.
b. Based on the results in the table, do you think the test will be on Friday? Explain.
Answer:

Question 9.
MODELING WITH MATHEMATICS
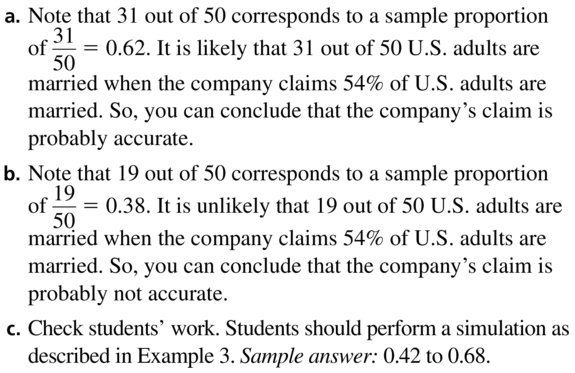
A national polling company claims that 54% of U.S. adults are married. You survey a random sample of 50 adults.
a. What can you conclude about the accuracy of the claim that the population proportion is 0.54 when 31 adults in your survey are married?
b. What can you conclude about the accuracy of the claim that the population proportion is 0.54 when 19 adults in your survey are married?
c. Assume that the true population proportion is 0.54. Estimate the variation among sample proportions for samples of size 50.
Answer:

Question 10.
MODELING WITH MATHEMATICS
Employee engagement is the level of commitment and involvement an employee has toward the company and its values. A national polling company claims that only 29% of U.S. employees feel engaged at work. You survey a random sample of 50 U.S. employees.
a. What can you conclude about the accuracy of the claim that the population proportion is 0.29 when 16 employees feel engaged at work?
b. What can you conclude about the accuracy of the claim that the population proportion is 0.29 when 23 employees feel engaged at work?
c. Assume that the true population proportion is 0.29. Estimate the variation among sample proportions for samples of size 50.
Answer:

In Exercises 11–16, find the margin of error for a survey that has the given sample size. Round your answer to the nearest tenth of a percent.
Question 11.
260
Answer:

Question 12.
1000
Answer:

Question 13.
2024
Answer:

Question 14.
6400
Answer:

Question 15.
3275
Answer:

Question 16.
750
Answer:

Question 17.
ATTENDING TO PRECISION
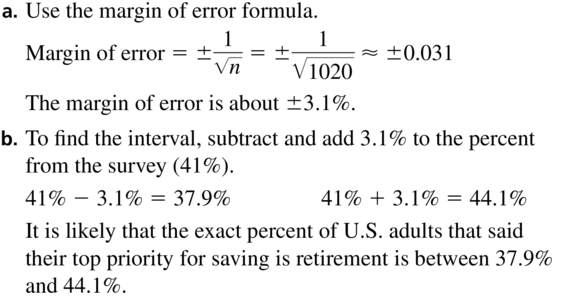
In a survey of 1020 U.S. adults, 41% said that their top priority for saving is retirement.
a. What is the margin of error for the survey?
b. Give an interval that is likely to contain the exact percent of all U.S. adults whose top priority for saving is retirement.
Answer:

Question 18.
ATTENDING TO PRECISION
In a survey of 1022 U.S. adults, 76% said that more emphasis should be placed on producing domestic energy from solar power.

a. What is the margin of error for the survey?
b. Give an interval that is likely to contain the exact percent of all U.S. adults who think more emphasis should be placed on producing domestic energy from solar power.
Answer:

Question 19.
ERROR ANALYSIS
In a survey, 8% of adult Internet users said they participate in sports fantasy leagues online. The margin of error is ±4%. Describe and correct the error in calculating the sample size.

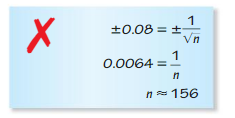
Answer:

Question 20.
ERROR ANALYSIS
In a random sample of 2500 consumers, 61% prefer Game A over Game B. Describe and correct the error in giving an interval that is likely to contain the exact percent of all consumers who prefer Game A over Game B.

Answer:

Question 21.
MAKING AN ARGUMENT
Your friend states that it is possible to have a margin of error between 0 and 100 percent, not including 0 or 100 percent. Is your friend correct? Explain your reasoning.
Answer:

Question 22.
HOW DO YOU SEE IT?
The figure shows the distribution of the sample proportions from three simulations using different sample sizes. Which simulation has the least margin of error? the greatest? Explain your reasoning.

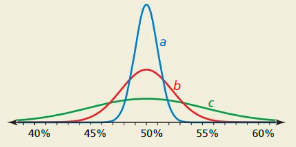
Answer:

Question 23.
REASONING
A developer claims that the percent of city residents who favor building a new football stadium is likely between 52.3% and 61.7%. How many residents were surveyed?

Answer:

Question 24.
ABSTRACT REASONING
Suppose a random sample of size n is required to produce a margin of error of ±E. Write an expression in terms of n for the sample size needed to reduce the margin of error to ±1—2E. How many times must the sample size be increased to cut the margin of error in half? Explain.
Answer:

Question 25.
PROBLEM SOLVING
A survey reported that 47% of the voters surveyed, or about 235 voters, said they voted for Candidate A and the remainder said they voted for Candidate B.
a. How many voters were surveyed?
b. What is the margin of error for the survey?
c. For each candidate, find an interval that is likely to contain the exact percent of all voters who voted for the candidate.
d. Based on your intervals in part (c), can you be confident that Candidate B won? If not, how many people in the sample would need to vote for Candidate B for you to be condent that Candidate B won? (Hint: Find the least number of voters for Candidate B so that the intervals do not overlap.)
Answer:

Question 26.
THOUGHT PROVOKING
Consider a large population in which ρ percent (in decimal form) have a certain characteristic. To be reasonably sure that you are choosing a sample that is representative of a population, you should choose a random sample of n people where n > 9\(\left(\frac{1-\rho}{\rho}\right)\) .
a. Suppose ρ= 0.5. How large does n need to be?
b. Suppose ρ= 0.01. How large does n need to be?
c. What can you conclude from parts (a) and (b)?
Answer:

Question 27.
CRITICAL THINKING
In a survey, 52% of the respondents said they prefer sports drink X and 48% said they prefer sports drink Y. How many people would have to be surveyed for you to be confident that sports drink X is truly preferred by more than half the population? Explain.
Answer:

Maintaining Mathematical Proficiency

Find the inverse of the function.
Question 28.
y= 10^x-3
Answer:

Question 29.
y= 2^x – 5
Answer:

Question 30.
y= ln (x+ 5)
Answer:

Question 31.
y= log₆x− 1
Answer:

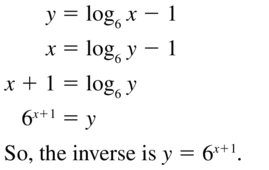
Determine whether the graph represents an arithmetic sequence or a geometric sequence. Then write a rule for the nth term.
Question 32.

Answer:

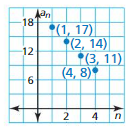
Question 33.

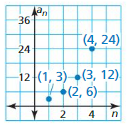
Answer:

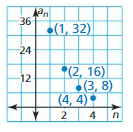
Question 34.

Answer:

Lesson 11.6 Making Inferences from Experiments

Essential Question How can you test a hypothesis about an experiment?

EXPLORATION 1

Resampling Data
Work with a partner. A randomized comparative experiment tests whether water with dissolved calcium affects the yields of yellow squash plants. The table shows the results.

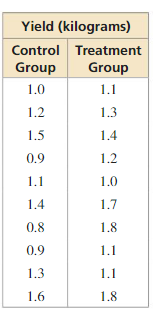
a. Find the mean yield of the control group and the mean yield of the treatment group. Then find the difference of the two means. Record the results.
b. Write each yield measurement from the table on an equal-sized piece of paper. Place the pieces of paper in a bag, shake, and randomly choose 10 pieces of paper. Call this the “control” group, and call the 10 pieces in the bag the “treatment” group. Then repeat part (a) and return the pieces to the bag. Perform this resampling experiment five times.
c. How does the difference in the means of the control and treatment groups compare with the differences resulting from chance?

EXPLORATION 2

Evaluating Results
Work as a class. To conclude that the treatment is responsible for the difference in yield, you need strong evidence to reject the hypothesis:
Water dissolved in calcium has no effect on the yields of yellow squash plants.
To evaluate this hypothesis, compare the experimental difference of means with the resampling differences.
a. Collect all the resampling differences of means found in Exploration 1(b) for the whole class and display these values in a histogram.
b. Draw a vertical line on your class histogram to represent the experimental difference of means found in Exploration 1(a).
c. Where on the histogram should the experimental difference of means lie to give evidence for rejecting the hypothesis?
d. Is your class able to reject the hypothesis? Explain your reasoning.

Communicate Your Answer

Question 3.
How can you test a hypothesis about an experiment?
Answer:

Question 4.
The randomized comparative experiment described in Exploration 1 is replicated and the results are shown in the table. Repeat Explorations 1 and 2 using this data set. Explain any differences in your answers.

Answer:

Monitoring Progress

Question 1.
In Example 1, interpret the meaning of \(\bar{x}_{\text {treatment }}\) \(\bar{x}_{\text {control }}\) control when the difference is (a) negative, (b) zero, and (c) positive.
Answer:

Question 2.
In Example 3, what are the consequences of concluding that the hypothesis is false when it is actually true?
Answer:

Making Inferences from Experiments 11.6 Exercises

Vocabulary and Core Concept Check
Question 1.
COMPLETE THE SENTENCE
A method in which new samples are repeatedly drawn from the data set is called ____________.
Answer:

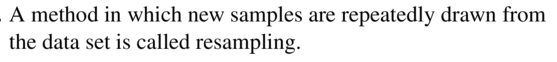
Question 2.
DIFFERENT WORDS, SAME QUESTION
Which is different? Find “both” answers.

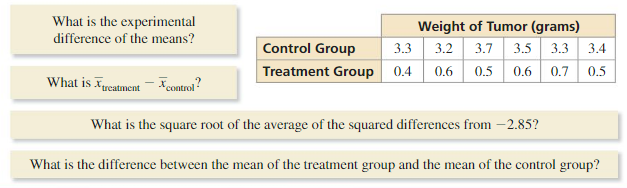
Answer:

Monitoring Progress and Modeling with Mathematics

Question 3.
PROBLEM SOLVING
A randomized comparative experiment tests whether music therapy affects the depression scores of college students. The depression scores range from 20 to 80, with scores greater than 50 being associated with depression. The control group has eight students and the treatment group, which receives the music therapy, has eight students. The table shows the results.

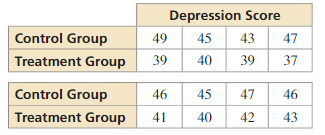
a. Find the mean score of the control group.
b. Find the mean score of the treatment group.
c. Find the experimental difference of the means.
d. Display the data in a double dot plot.
e. What can you conclude?

Answer:

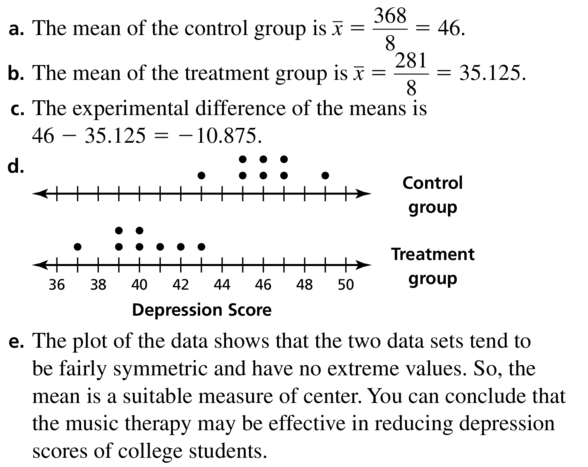
Question 4.
PROBLEM SOLVING
A randomized comparative experiment tests whether low-level laser therapy affects the waist circumference of adults. The control group has eight adults and the treatment group, which receives the low-level laser therapy, has eight adults. The table shows the results.

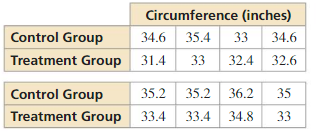
a. Find the mean circumference of the control group.
b. Find the mean circumference of the treatment group.
c. Find the experimental difference of the means.
d. Display the data in a double dot plot.
e. What can you conclude?
Answer:

Question 5.
ERROR ANALYSIS
In a randomized comparative experiment, the mean score of the treatment group is 11 and the mean score of the control group is 16. Describe and correct the error in interpreting the experimental difference of the means.

Answer:

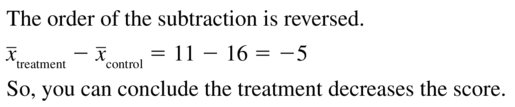
Question 6.
REASONING
In Exercise 4, interpret the meaning of \(\bar{x}_{\text {treatment }}-\bar{x}_{\text {control }}\) when the difference is positive, negative, and zero.
Answer:

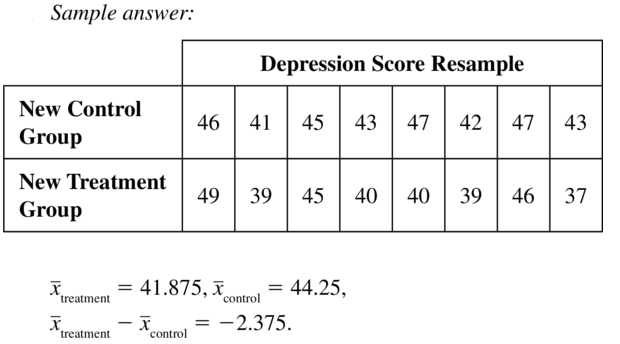
Question 7.
MODELING WITH MATHEMATICS
Resample the data in Exercise 3 using a simulation. Use the means of the new control and treatment groups to calculate the difference of the means.
Answer:

Question 8.
MODELING WITH MATHEMATICS
Resample the data in Exercise 4 using a simulation. Use the means of the new control and treatment groups to calculate the difference of the means.
Answer:

Question 9.
DRAWING CONCLUSIONS
To analyze the hypothesis below, use the histogram which shows the results from 200 resamplings of the data in Exercise 3.
Music therapy has no effect on the depression score.
Compare the experimental difference in Exercise 3 with the resampling differences. What can you conclude about the hypothesis? Does music therapy have an effect on the depression score?

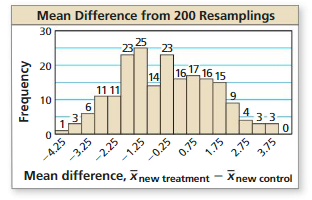
Answer:

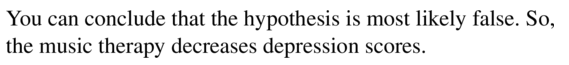
Question 10.
DRAWING CONCLUSIONS
Suppose the experimental difference of the means in Exercise 3 had been −0.75. Compare this experimental difference of means with the resampling differences in the histogram in Exercise 9. What can you conclude about the hypothesis? Does music therapy have an effect on the depression score?
Answer:

Question 11.
WRITING
Compare the histogram in Exercise 9 to the histogram below. Determine which one provides stronger evidence against the hypothesis, Music therapy has no effect on the depression score. Explain.

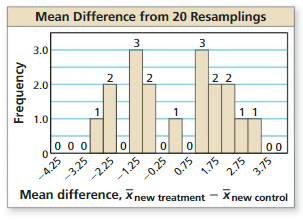
Answer:

Question 12.
HOW DO YOU SEE IT?
Without calculating, determine whether the experimental difference, \(\bar{x}_{\text {treatment }}-\bar{x}_{\text {control }}\), is positive, negative, or zero. What can you conclude about the effect of the treatment? Explain.

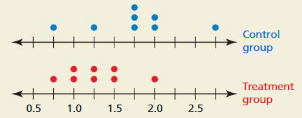
Answer:

Question 13.
MAKING AN ARGUMENT
Your friend states that the mean of the resampling differences of the means should be close to 0 as the number of resamplings increase. Is your friend correct? Explain your reasoning.
Answer:

Question 14.
THOUGHT PROVOKING
Describe an example of an observation that can be made from an experiment. Then give four possible inferences that could be made from the observation.
Answer:

Question 15.
CRITICAL THINKING
In Exercise 4, how many resamplings of the treatment and control groups are theoretically possible? Explain.
Answer:

Maintaining Mathematical Proficiency

Factor the polynomial completely.
Question 16.
5x³ − 15x²
Answer:

Question 17.
y³ − 8
Answer:

Question 18.
z³ + 5z² − 9z − 45
Answer:

Question 19.
81w⁴ − 16
Answer:

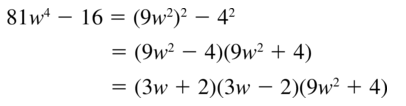
Determine whether the inverse of f is a function. Then find the inverse.
Question 20.
f(x) = \(\frac{3}{x+5}\)
Answer:

Question 21.
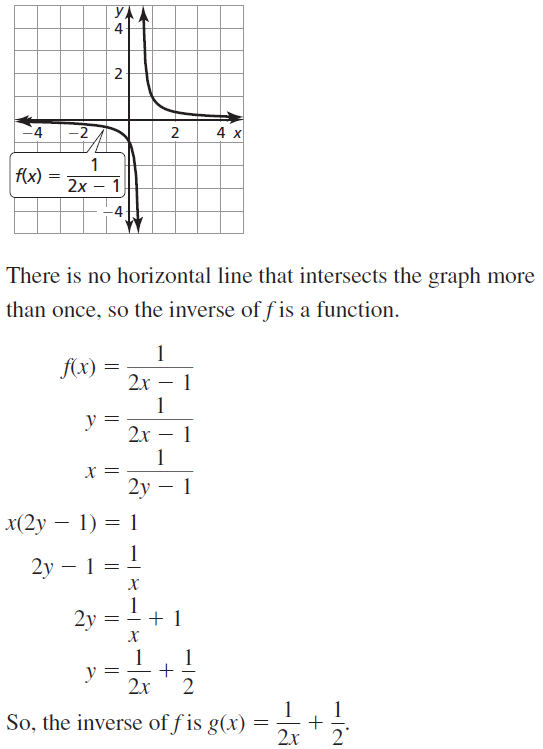
f(x) = \(\frac{1}{2x-1}\)
Answer:

Question 22.
f(x) = \(\frac{2}{x}\) − 4
Answer:

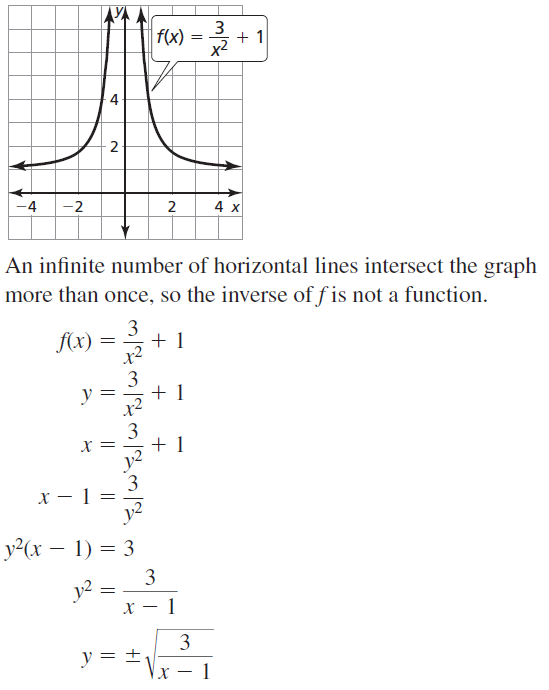
Question 23.
f(x) = \(\frac{3}{x^{2}}\) + 1
Answer:

Data Analysis and Statistics Performance Task: Curving the Test

11.4–11.6 What Did You Learn?

Core Vocabulary

Core Concepts
Section 11.4
Randomization in Experiments and Observational Studies, p. 621
Comparative Studies and Causality, p. 621
Analyzing Experimental Designs, p. 622

Section 11.5
Estimating Population Parameters, p. 626
Analyzing Estimated Population Parameters, p. 628

Section 11.6
Experiments with Two Samples, p. 634
Resampling Data Using Simulations, p. 635
Making Inferences About Treatments, p. 636

Mathematical Practices
Question 1.
In Exercise 7 on page 623, find a partner and discuss your answers. What questions should you ask your partner to determine whether an observational study or an experiment is more appropriate?
Answer:

Question 2.
In Exercise 23 on page 632, how did you use the given interval to find the sample size?
Answer:

Performance Task: Curving the Test
Test scores are sometimes curved for different reasons using different techniques. Curving began with the assumption that a good test would result in scores that were normally distributed about a C average. Is this assumption valid? Are test scores in your class normally distributed? If not, how are they distributed? Which curving algorithms preserve the distribution and which algorithms change it?
To explore the answers to these questions and more, go to BigIdeasMath.com.

Data Analysis and Statistics Chapter Review

11.1 Using Normal Distributions (pp. 595–602)

Question 1.
A normal distribution has mean μ and standard deviation σ. An x-value is randomly selected from the distribution. Find P(x ≤ μ − 3σ).
Answer:

Question 2.
The scores received by juniors on the math portion of the PSAT are normally distributed with a mean of 48.6 and a standard deviation of 11.4. What is the probability that a randomly selected score is at least 76?
Answer:

11.2 Populations, Samples, and Hypotheses (pp. 603−608)

Question 3.
To estimate the average number of miles driven by U.S. motorists each year, a researcher conducts a survey of 1000 drivers, records the number of miles they drive in a year, and then determines the average. Identify the population and the sample.
Answer:

Question 4.
A pitcher throws 40 fastballs in a game. A baseball analyst records the speeds of 10 fastballs and finds that the mean speed is 92.4 miles per hour. Is the mean speed a parameter or a statistic? Explain.
Answer:

Question 5.
A prize on a game show is placed behind either Door A or Door B. You suspect the prize is more often behind Door A. The show host claims the prize is randomly placed behind either door. What should you conclude when the prize is behind Door A for 32 out of 50 contestants?
Answer:

11.3 Collecting Data (pp. 609−616)

Question 6.
A researcher wants to determine how many people in a city support the construction of a new road connecting the high school to the north side of the city. Fifty residents from each side of the city are surveyed. Identify the type of sample described and determine whether the sample is biased.
Answer:

Question 7.
A researcher records the number of people who use a coupon when they dine at a certain restaurant. Identify the method of data collection.
Answer:

Question 8.
Explain why the survey question below may be biased or otherwise introduce bias into the survey. Then describe a way to correct the flaw.
“Do you think the city should replace the outdated police cars it is using?”
Answer:

11.4 Experimental Design (pp. 619–624)

Question 9.
A restaurant manager wants to know which type of sandwich bread attracts the most repeat customers. Is the topic best investigated through an experiment or an observational study? Describe how you would design the experiment or observational study.
Answer:

Question 10.
A researcher wants to test the effectiveness of a sleeping pill. Identify a potential problem, if any, with the experimental design below. Then describe how you can improve it.
The researcher asks for 16 volunteers who have insomnia. Eight volunteers are given the sleeping pill and the other 8 volunteers are given a placebo. Results are recorded for 1 month.
Answer:

Question 11.
Determine whether the study is a randomized comparative experiment. If it is, describe the treatment, the treatment group, and the control group. If it is not, explain why not and discuss whether the conclusions drawn from the study are valid.

Answer:

11.5 Making Inferences from Sample Surveys (pp. 625−632)

Question 12.
In a survey of 1017 U.S. adults, 62% said that they prefer saving money over spending it. Give an interval that is likely to contain the exact percent of all U.S. adults who prefer saving money over spending it.
Answer:

Question 13.
There are two candidates for homecoming king. The table shows the results from four random surveys of the students in the school. The students were asked whether they will vote for Candidate A.Do you think Candidate A will be the homecoming king? Explain.

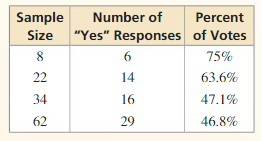
Answer:

11.6 Making Inferences from Experiments (pp. 633−638)

Question 14.
Describe how to use a simulation to resample the data in the example above. Explain how this allows you to make inferences about the data when the sample size is small.
Answer:

Data Analysis and Statistics Chapter Test

Question 1.
Market researchers want to know whether more men or women buy their product. Explain whether this research topic is best investigated through an experiment or an observational study. Then describe the design of the experiment or observational study.
Answer:

Question 2.
You want to survey 100 of the 2774 four-year colleges in the United States about their tuition cost. Describe a method for selecting a random sample of colleges to survey.
Answer:

Question 3.
The grade point averages of all the students in a high school are normally distributed with a mean of 2.95 and a standard deviation of 0.72. Are these numerical values parameters or statistics? Explain.
Answer:

A normal distribution has a mean of 72 and a standard deviation of 5. Find the probability that a randomly selected x-value from the distribution is in the given interval.
Question 4.
between 67 and 77
Answer:

Question 5.
at least 75
Answer:

Question 6.
at most 82
Answer:

Question 7.
A researcher wants to test the effectiveness of a new medication designed to lower blood pressure. Identify a potential problem, if any, with the experimental design. Then describe how you can improve it.
The researcher identifies 30 people with high blood pressure. Fifteen people with the highest blood pressures are given the medication and the other 15 are given a placebo. After 1 month, the subjects are evaluated.
Answer:

Question 8.
A randomized comparative experiment tests whether a vitamin supplement increases human bone density (in grams per square centimeter). The control group has eight people and the treatment group, which receives the vitamin supplement, has eight people. The table shows the results.

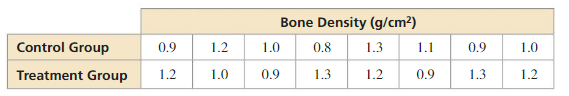
a. Find the mean yields of the control group, \(\bar{x}_{\text {control }}\), and the treatment group, \(\bar{x}_{\text {treatment }}\).
b. Find the experimental difference of the means, \(\bar{x}_{\text {treatment }}-\bar{x}_{\text {control }}\).
c. Display the data in a double dot plot. What can you conclude?
d. Five hundred resamplings of the data are simulated. Out of the 500 resampling differences, 231 are greater than the experimental difference in part (b). What can you conclude about the hypothesis, The vitamin supplement has no effect on human bone density? Explain your reasoning.
Answer:

Question 9.
In a recent survey of 1600 randomly selected U.S. adults, 81% said they have purchased a product online.

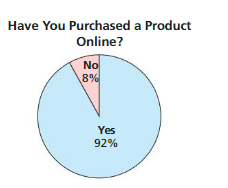
a. Identify the population and the sample. Describe the sample.
b. Find the margin of error for the survey.
c. Give an interval that is likely to contain the exact percent of all U.S. adults who have purchased a product online.
d. You survey 75 teachers at your school. The results are shown in the graph. Would you use the recent survey or your survey to estimate the percent of U.S. adults who have purchased a product online? Explain.
Answer:

Data Analysis and Statistics Cumulative Assessment

Question 1.
Your friend claims any system formed by three of the following equations will have exactly one solution.

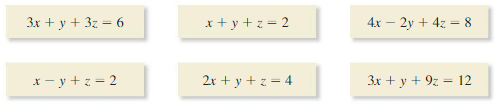
a. Write a linear system that would support your friend’s claim.
b. Write a linear system that shows your friend’s claim is incorrect.
Answer:

Question 2.
Which of the following samples are biased? If the sample is biased, explain why it is biased.
A. A restaurant asks customers to participate in a survey about the food sold at the restaurant. The restaurant uses the surveys that are returned.
B. You want to know the favorite sport of students at your school. You randomly select athletes to survey at the winter sports banquet.
C. The owner of a store wants to know whether the store should stay open 1 hour later each night. Each cashier surveys every fifth customer.
D. The owner of a movie theater wants to know whether the volume of its movies is too loud. Patrons under the age of 18 are randomly surveyed.
Answer:

Question 3.
A survey asks adults about their favorite way to eat ice cream. The results of the survey are displayed in the table shown.

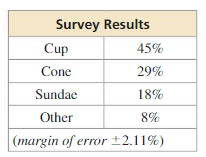
a. How many people were surveyed?
b. Why might the conclusion, “Adults generally do not prefer to eat their ice cream in a cone” be inaccurate to draw from this data?
c. You decide to test the results of the poll by surveying adults chosen at random. What is the probability that at least three out of the six people you survey prefer to eat ice cream in a cone?
d. Four of the six respondents in your study said they prefer to eat their ice cream in a cone. You conclude that the other survey is inaccurate. Why might this conclusion be incorrect?
e. What is the margin of error for your survey?
Answer:

Question 4.
You are making a lampshade out of fabric for the lamp shown. The pattern for the lampshade is shown in the diagram on the left.
a. Use the smaller sector to write an equation that relates θ and x.
b. Use the larger sector to write an equation that relates θ and x+ 10.
c. Solve the system of equations from parts (a) and (b) for x and θ.
d. Find the amount of fabric (in square inches) that you will use to make the lampshade.

Answer:

Question 5.
For all students taking the Medical College Admission Test over a period of 3 years, the mean score was 25.1. During the same 3 years, a group of 1000 students who took the test had a mean score of 25.3. Classify each mean as a parameter or a statistic. Explain.
Answer:

Question 6.
Complete the table for the four equations. Explain your reasoning.

Answer:

Question 7.
The normal distribution shown has mean 63 and standard deviation 8. Find the percent of the area under the normal curve that is represented by the shaded region. Then describe another interval underthe normal curve that has the same area.

Answer:

Question 8.
Which of the rational expressions cannot be simplified? (HSA-APR.D.6)

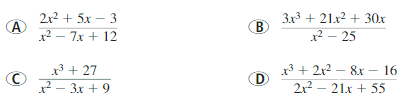
Answer: