Engage NY Eureka Math 6th Grade Module 4 Lesson 12 Answer Key
Eureka Math Grade 6 Module 4 Lesson 12 Example Answer Key
Example 1.
Write an expression that is equivalent to 2(a + b).
Answer:
→ In this example, we have been given the factored form of the expression.
→ To answer this question, we can create a model to represent 2(a + b).
→ Let’s start by creating a model to represent (a + b).
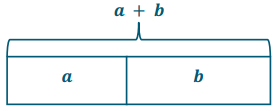
Create a model to represent (a + b).
Answer:
The expression 2(a + b) tells us that we have 2 of the (a + b)’s. Create a model that shows 2 groups of (a + b).
Answer:
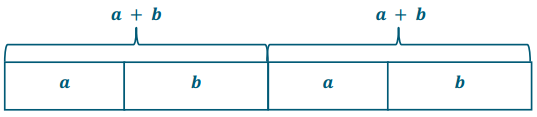
How many a’s and how many b’s do you see in the diagram?
Answer:
There are 2 a’s and 2 b’s.
How would the model look if we grouped together the a’s and then grouped together the b’s?
Answer:
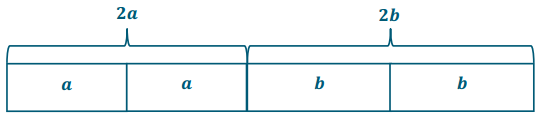
What expression could we write to represent the new diagram?
Answer:
2a + 2b
What conclusion can we draw from the models about equivalent expressions?
Answer:
2(a + b) = 2a + 2b
Let a = 3 and b = 4.
Answer:
2(a+b) 2a + 2b
2(3 + 4) 2(3) + 2(4)
2(7) 6 + 8
14 14
What happens when we double (a + b)?
Answer:
We double a, and we double b.
Example 2.
Write an expression that Is equivalent to double (3x + 4y).
How can we rewrite double (3x + 4y)?
Answer:
Double is the same as multiplying by two.
2(3x + 4y) or 6x + 8y
Is this expression In factored form, expanded form, or neither?
Answer:
The first expression is in factored form, and the second expression is in expanded form.
Let’s start this problem the same way that we started the first example. What should we do?
Answer:
We can make a model of 3x + 4y.
![]()
Are there terms that we can combine in this example?
Answer:

Yes. There are 6 x’s and 8y’s.
So, the model is showing 6x + 8y.
What is an equivalent expression that we can use to represent 2(3x + 4y)?
Answer:
2(3x + 4y) = 6x + 8y
This is the same as 2(3x) + 2(4y).
Summarize how you would solve this question without the model.
Answer:
When there is a number outside the parentheses, I would multiply it by all the terms on the inside of the parentheses.
Example 3.
Write an expression in expanded form that is equivalent to the model below.
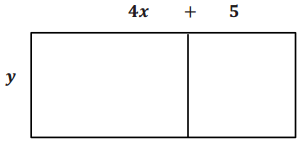
What factored expression is represented in the model?
Answer:
y(4x + 5)
How can we rewrite this expression in expanded form?
Answer:
y(4x) + y(5)
4xy + 5y
Example 4.
Write an expression in expanded form that is equivalent to 3(7d + 4e).
Answer:
We will multiply 3 × 7d and 3 × 4e.
We would get 21d + 12e. So, 3(7d+ 4e) = 21d + 12e.
Eureka Math Grade 6 Module 4 Lesson 12 Exercise Answer Key
Exercises
Create a model for each expression below. Then, write another equivalent expression using the distributive property.
Exercise 1.
3(x + y)
Answer:
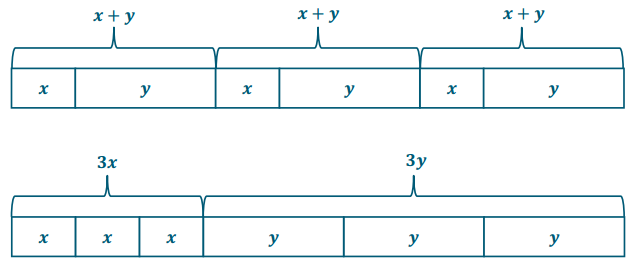
3x + 3y
Exercise 2.
4(2h + g)
Answer:
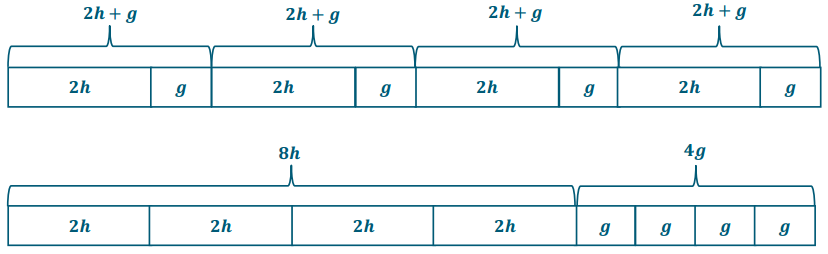
8h + 4g
Apply the distributive property to write equivalent expressions in expanded form.
Exercise 3.
8(h + 3)
Answer:
8k + 24
Exercise 4.
3(2h + 7)
Answer:
6k + 21
Exercise 5.
5(3x + 9y)
Answer:
15x + 45y
Exercise 6.
4(11k + 3g)
Answer:
44h + 12g
Exercise 7.
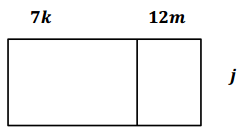
Answer:
7jk + 12jm
Exercise 8.
a(9b + 13)
Answer:
9ab + 13a
Eureka Math Grade 6 Module 4 Lesson 12 Problem Set Answer Key
Question 1.
Use the distributive property to write the following expressions in expanded form.
a. 4(x + y)
Answer:
4x + 4y
b. 8(a + 3b)
Answer:
8a + 24h
c. 3(2x + 11y)
Answer:
6x + 33y
d. 9(7a + 6b)
Answer:
63a + 54b
e. c(3a + b)
Answer:
3ac + bc
f. y(2x + 11z)
Answer:
2xy + 11yz
Question 2.
Create a model to show that 2(2x + 3y) = 4x + 6y.
Answer:
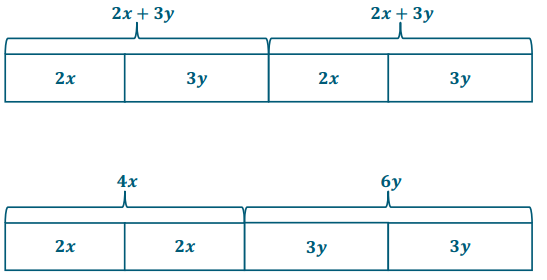
Eureka Math Grade 6 Module 4 Lesson 12 Exit Ticket Answer Key
Use the distributive property to write the following expressions in expanded form.
Question 1.
2(b + c)
2h + 2c
Question 2.
5(7h + 3m)
Answer:
35h + 15m
Question 3.
e(f + g)
Answer:
ef + eg
Eureka Math Grade 6 Module 4 Lesson 12 Opening Exercise Answer Key
a. Create a model to show 2 × 5.
Answer:

b. Create a model to show 2 × b, or 2b
Answer:
