Engage NY Eureka Math 8th Grade Module 2 Lesson 14 Answer Key
Eureka Math Grade 8 Module 2 Lesson 14 Example Answer Key
Example 1
Find the measure of angle x.
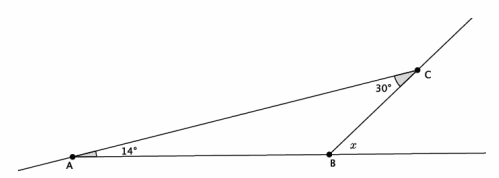
Answer:
We need to find the sum of the measures of the remote interior angles to find the measure of the exterior angle x:
14+30=44. Therefore, the measure of ∠x is 44°.
→ Present an informal argument that proves you are correct.
→ We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠ABC must be 136°, which means that ∠x is 44°.
Example 2.
Find the measure of angle x.
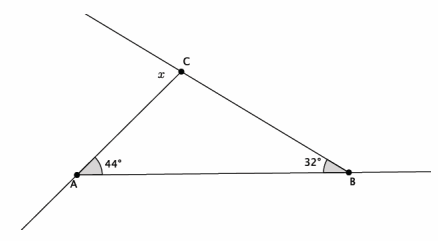
Answer:
We need to find the sum of the measures of the remote interior angles to find the measure of the exterior angle x: 44+32=76. Therefore, the measure of ∠x is 76°.
→ Present an informal argument that proves you are correct.
→ We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠ACB must be 104°, which means that ∠x is 76°.
Example 3.
Find the measure of angle x.
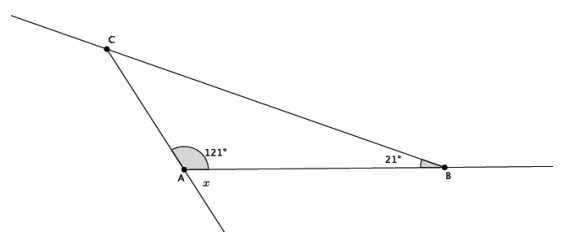
Answer:
180-121=59. Therefore, the measure of ∠x is 59°.
Students should notice that they are not given the two remote interior angles associated with the exterior angle x.
For that reason, they must use what they know about straight angles (or supplementary angles) to find the measure of
angle x.
Example 4.
Find the measure of angle x.
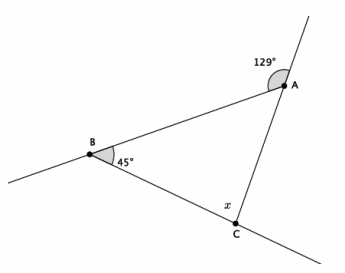
Answer:
129-45=84. Therefore, the measure of ∠x is 84°.
Eureka Math Grade 8 Module 2 Lesson 14 Exercise Answer Key
Exercises 1–4
Use the diagram below to complete Exercises 1–4.
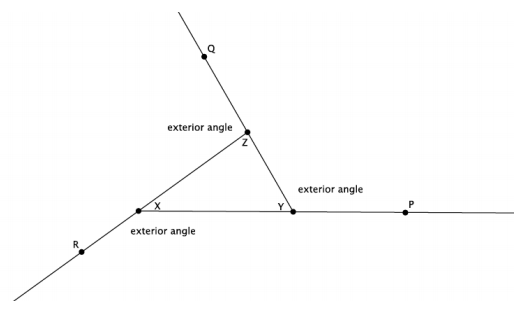
Exercise 1.
Name an exterior angle and the related remote interior angles.
Answer:
The exterior angle is ∠ZYP, and the related remote interior angles are ∠YZX and ∠ZXY.
Exercise 2.
Name a second exterior angle and the related remote interior angles.
Answer:
The exterior angle is ∠XZQ, and the related remote interior angles are ∠ZYX and ∠ZXY.
Exercise 3.
Name a third exterior angle and the related remote interior angles.
Answer:
The exterior angle is ∠RXY, and the related remote interior angles are ∠ZYX and ∠XZY.
Exercise 4.
Show that the measure of an exterior angle is equal to the sum of the measures of the related remote interior angles.
Answer:
Triangle XYZ has interior angles ∠XYZ, ∠YZX, and ∠ZXY. The sum of those angles is 180°. The straight angle ∠XYP also has a measure of 180° and is made up of angles ∠XYZ and ∠ZYP. Since the triangle and the straight angle have the same number of degrees, we can write the sum of their respective angles as an equality:
∠XYZ+∠YZX+∠ZXY=∠XYZ+ZYP.
Both the triangle and the straight angle share ∠XYZ. We can subtract the measure of that angle from the triangle and the straight angle. Then, we have
∠YZX+∠ZXY=∠ZYP,
where the angle ∠ZYP is the exterior angle, and the angles ∠YZX and ∠ZXY are the related remote interior angles of the triangle. Therefore, the sum of the measures of the remote interior angles of a triangle are equal to the measure of the exterior angle.
Exercise 5–10
Question 5.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
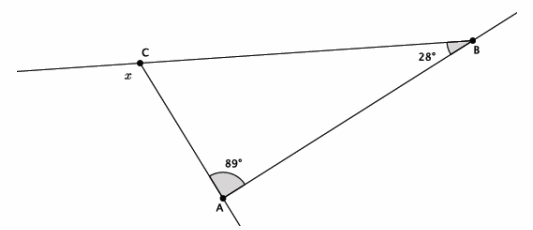
Answer:
Since 89+28 = 117, the measure of angle x is 117°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠ACB must be 63°, which means that ∠x is 117°.
Question 6.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
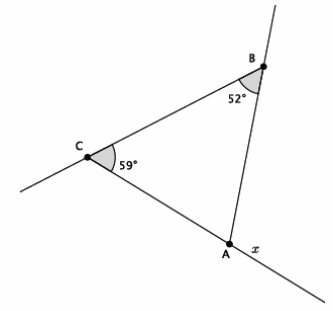
Answer:
Since 59+52=111, the measure of angle x is 111°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠CAB must be 69°, which means that ∠x is 111°.
Question 7.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
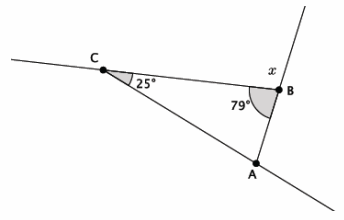
Answer:
Since 180-79=101, the measure of angle x is 101°. We know that straight angles are 180°, and the straight angle in the diagram is made up of ∠ABC and ∠x. ∠ABC is 79°, which means that ∠x is 101°.
Question 8.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
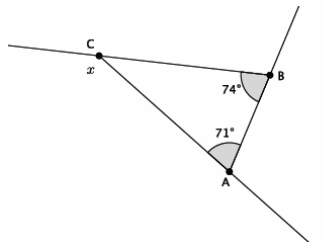
Answer:
Since 71+74=145, the measure of angle x is 145°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠ACB must be 35°, which means that ∠x is 145°.
Question 9.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
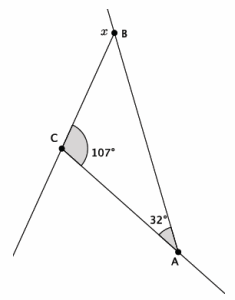
Answer:
Since 107+32=139, the measure of angle x is 139°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠CBA must be 41°, which means that x is 139°.
Question 10.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
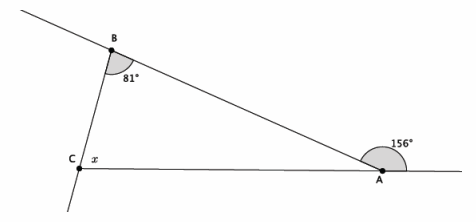
Answer:
Since 156-81 = 75, the measure of angle x is 75°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠BAC must be 24° because it is part of the straight angle. Then, ∠x=180°-(81°+24°), which means ∠x is 75°.
Eureka Math Grade 8 Module 2 Lesson 14 Exit Ticket Answer Key
Question 1.
Find the measure of angle p. Present an informal argument showing that your answer is correct.
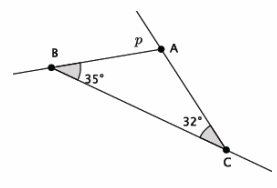
Answer:
The measure of angle p is 67°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠BAC must be 113°, which means that ∠p is 67°.
Question 2.
Find the measure of angle q. Present an informal argument showing that your answer is correct.
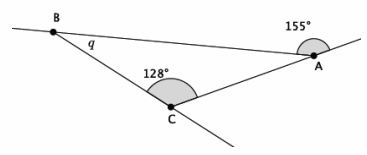
Answer:
The measure of angle q is 27°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠CAB must be 25°, which means that ∠q is 27°.
Question 3.
Find the measure of angle r. Present an informal argument showing that your answer is correct.
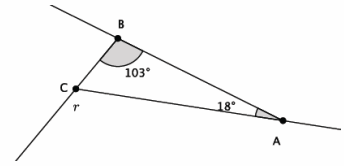
Answer:
The measure of angle r is 121°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠BCA must be 59°, which means that ∠r is 121°.
Eureka Math Grade 8 Module 2 Lesson 14 Problem Set Answer Key
Students practice finding missing angle measures of triangles.
For each of the problems below, use the diagram to find the missing angle measure. Show your work.
Question 1.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
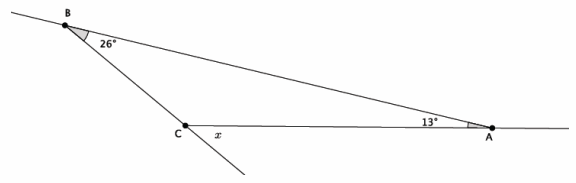
Answer:
Since 26+13=39, the measure of angle x is 39°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠BCA must be 141°, which means that ∠x is 39°.
Question 2.
Find the measure of angle x.
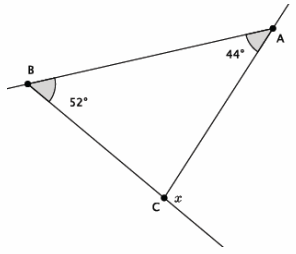
Answer:
Since 52+44=96, the measure of angle x is 96°.
Question 3.
Find the measure of angle x. Present an informal argument showing that your answer is correct.
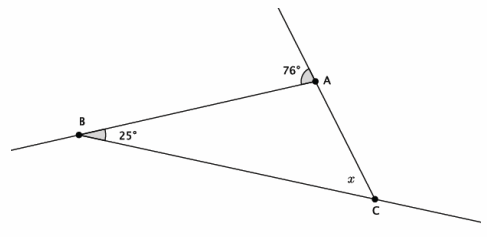
Answer:
Since 76-25=51, the measure of ∠x is 51°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°. ∠BAC must be 104° because it is part of the straight angle. Then, x=180°-(104°+25°), which means ∠x is 51°.
Question 4.
Find the measure of angle x.
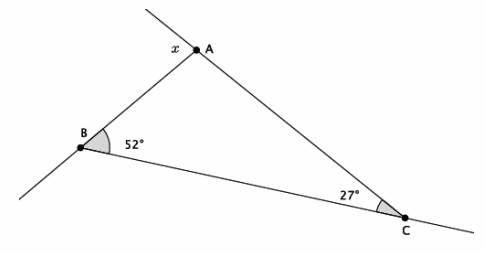
Answer:
Since 27+52 =79, the measure of angle x is 79°.
Question 5.
Find the measure of angle x.
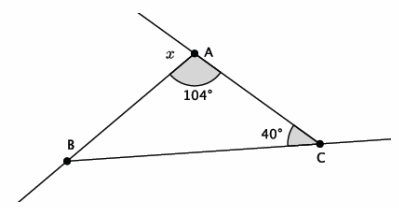
Answer:
Since 180-104=76, the measure of angle x is 76°.
Question 6.
Find the measure of angle x.
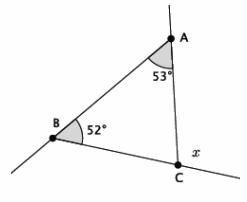
Answer:
Since 52+53=105, the measure of angle x is 105°.
Question 7.
Find the measure of angle x.
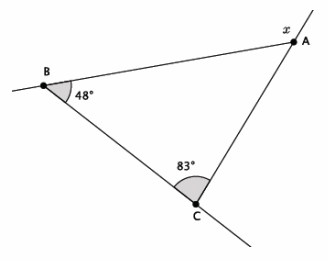
Answer:
Since 48+83=131, the measure of angle x is 131°.
Question 8.
Find the measure of angle x.
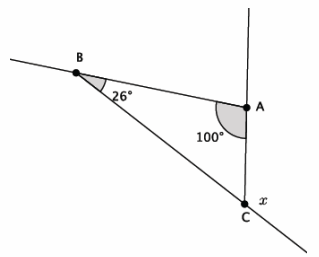
Answer:
Since 100+26=126, the measure of angle x is 126°.
Question 9.
Find the measure of angle x.
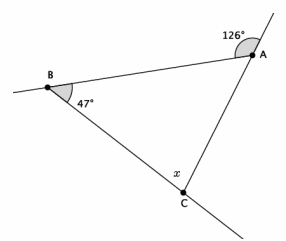
Answer:
Since 126-47=79, the measure of angle x is 79°.
Question 10.
Write an equation that would allow you to find the measure of angle x. Present an informal argument showing that your answer is correct.
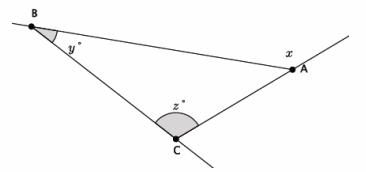
Answer:
Since y+z=x, the measure of angle x is (y+z)°. We know that triangles have a sum of interior angles that is equal to 180°. We also know that straight angles are 180°.
Then, ∠y+∠z+∠BAC=180°, and ∠x+∠BAC=180°. Since both equations are equal to 180°,
then ∠y+∠z+∠BAC=∠x+∠BAC. Subtract ∠BAC from each side of the equation, and you get ∠y+∠z=∠x.