The solutions of Grade 6 Go Math Answer Key for Chapter 7 Exponents are available in simple PDFs here. With the help off the HMH Go Math Grade 6 Chapter 7 Exponents Answer Ley can be easily downloaded by the students by using the provided links. You can understand the concept of the standard form in this article. So, Download a free pdf of Go Math Grade 6 Answer Key Chapter 7 Exponents.
Go Math Grade 6 Answer Key Chapter 7 Exponents
Our main aim is to provide a brief explanation of all the questions. We have provided the table of contents of chapter 7 Exponents in the below section. So, once go through the topics before you start your preparation. This will help you to know in which topic you are lagging. Hence make use of the resources provided on this page and try to score good marks in the exams. After your preparation we suggest the students to test your skills by solving the questions in the mid-chapter checkpoint and review test.
Lesson 1: Exponents
- Share and Show – Page No. 359
- Bacterial Growth – Page No. 360
- Exponents – Page No. 361
- Lesson Check – Page No. 362
Lesson 2: Evaluate Expressions Involving Exponents
- Share and Show – Page No. 365
- Problem Solving + Applications – Page No. 366
- Evaluate Expressions Involving Exponents – Page No. 367
- Lesson Check – Page No. 368
Lesson 3: Write Algebraic Expressions
- Share and Show – Page No. 371
- Unlock the Problem – Page No. 372
- Write Algebraic Expressions – Page No. 373
- Lesson Check – Page No. 374
Lesson 4: Identify Parts of Expressions
- Share and Show – Page No. 377
- Problem Solving + Applications – Page No. 378
- Identify Parts of Expressions – Page No. 379
- Lesson Check – Page No. 380
Lesson 5: Evaluate Algebraic Expressions and Formulas
- Share and Show – Page No. 383
- Problem Solving + Applications – Page No. 384
- Evaluate Algebraic Expressions and Formulas – Page No. 385
- Lesson Check – Page No. 386
Mid-Chapter Checkpoint
Lesson 6: Use Algebraic Expressions
- Share and Show – Page No. 391
- Problem Solving + Applications – Page No. 392
- Use Algebraic Expressions – Page No. 393
- Lesson Check – Page No. 394
Lesson 7: Problem Solving • Combine Like Terms
- Share and Show – Page No. 397
- On Your Own – Page No. 398
- Problem Solving Combine Like Terms – Page No. 399
- Lesson Check – Page No. 400
- Share and Show – Page No. 403
- Problem Solving + Applications – Page No. 404
Lesson 8: Generate Equivalent Expressions
- Generate Equivalent Expressions – Page No. 405
- Lesson Check – Page No. 406
- Share and Show – Page No. 409
- Problem Solving + Applications – Page No. 410
Lesson 9: Identify Equivalent Expressions
Chapter 7 Review/Test
- Chapter 7 Review/Test – Page No. 413
- Chapter 7 Review/Test – Page No. 414
- Chapter 7 Review/Test – Page No. 415
- Chapter 7 Review/Test – Page No. 416
- Chapter 7 Review/Test – Page No. 417
- Chapter 7 Review/Test – Page No. 418
Share and Show – Page No. 359
Question 1.
Write 24 by using repeated multiplication. Then find the value of 24.
___________
Answer: 16
Explanation:
The repeated factor is 2
The number 2 is repeated 4 times.
The repeated multiplication of 24 is 2 × 2 × 2 × 2 = 16
Thus the value of 24 is 16.
Use one or more exponents to write the expression.
Question 2.
7 × 7 × 7 × 7
Type below:
_____________
Answer: 74
Explanation:
The repeated factor is 7.
7 is repeated four times.
The exponent of the repeated multiplication 7 × 7 × 7 × 7 is 74
Question 3.
5 × 5 × 5 × 5 × 5
Type below:
_____________
Answer: 55
Explanation:
The repeated factor is 5. The number 5 is repeated five times.
The exponent of the repeated multiplication 5 × 5 × 5 × 5 × 5 is 55
Question 4.
3 × 3 × 4 × 4
Type below:
_____________
Answer: 32 × 42
Explanation:
The exponent of the repeated multiplication 3 × 3 is 32
The exponent of the repeated multiplication 4 × 4 is 42
Thus the exponent for 3 × 3 × 4 × 4 is 32 × 42
On Your Own
Find the value.
Question 5.
202
______
Answer: 20 × 20 = 400
Explanation:
The repeated factor is 20
Write the factor 2 times.
20 × 20 = 400
The value of 202 = 400
Question 6.
821
______
Answer: 82
Explanation:
The repeated factor is 82
Write the factor 1 time.
The value of 821 is 82
Question 7.
35
Answer: 3 × 3 × 3 × 3 × 3 = 243
Explanation:
The repeated factor is 3
Write the factor 5 times.
The value of 35 is 343
Question 8.
Write 32 as a number with an exponent by using 2 as the base.
Type below:
_____________
Answer: 25
Explanation:
The exponent of 32 by using the base 2 is 2 × 2 × 2 × 2 × 2 = 25
Complete the statement with the correct exponent.
Question 9.
5? = 125
______
Answer: 53
Explanation:
The exponential form of 125 is 5 × 5 × 5 = 53
5? = 125
5? = 53
When bases are equal powers should be equated.
Thus the exponent is 3
Question 10.
16? = 16
______
Answer: 1
Explanation:
The exponential form of 16 is 161
16? = 161
When bases are equal powers should be equated.
Thus the exponent is 1.
Question 11.
30? = 900
______
Answer: 2
Explanation:
The exponential form of 900 is 30 × 30 = 302
30? = 302
When bases are equal powers should be equated.
Thus the exponent is 2.
Question 12.
Use Repeated Reasoning Find the values of 41, 42, 43, 44, and 45. Look for a pattern in your results and use it to predict the ones digit in the value of 46.
Type below:
_____________
Answer:
The value of 41 is 4.
The value of 42 is 4 × 4 = 16
The value of 43 is 4 × 4 × 4 = 64
The value of 44 is 4 × 4 × 4 × 4 = 256
The value of 45 is 4 × 4 × 4 × 4 × 4 = 1024
The value of 46 is 4 × 4 × 4 × 4 × 4 × 4 = 4096
Question 13.
Select the expressions that are equivalent to 32. Mark all that apply.
Options:
a. 25
b. 84
c. 23 × 4
d. 2 × 4 × 4
Answer: 25
Explanation:
The exponent of 32 by using the base 2 is 2 × 2 × 2 × 2 × 2 = 25
32 = 25
Thus the correct answer is option A.
Bacterial Growth – Page No. 360
Bacteria are tiny, one-celled organisms that live almost everywhere on Earth. Although some bacteria cause disease, other bacteria are helpful to humans, other animals, and plants. For example, bacteria are needed to make yogurt and many types of cheese.
Under ideal conditions, a certain type of bacterium cell grows larger and then splits into 2 “daughter” cells. After 20 minutes, the daughter cells split, resulting in 4 cells. This splitting can happen again and again as long as conditions remain ideal.
Complete the table.
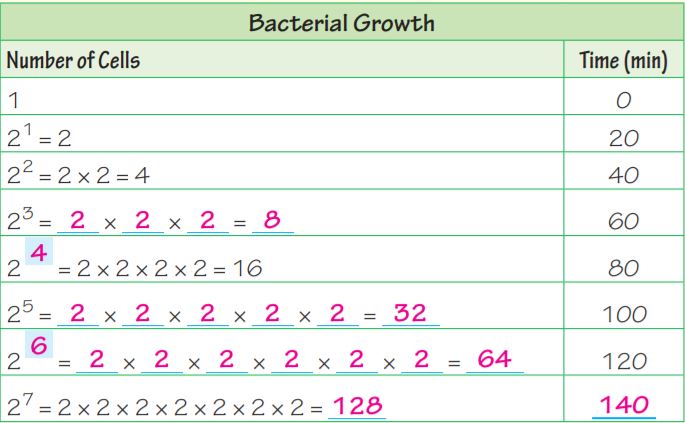
Extend the pattern in the table above to answer 14 and 15.
Question 14.
What power of 2 shows the number of cells after 3 hours? How many cells are there after 3 hours?
Type below:
_____________
Answer: 29
Explanation:
So, each cell doubles every 20 mins. After 20 minutes, you have 1(2) = 2 cells. After 40 minutes, you have 2(2) = 4 cells, etc.
1 hour = 60 minutes
3 hours = 3 × 60 minutes = 180 minutes
180/20 = 9 divisions
Thus 29 cells are there after 3 hours.
Question 15.
How many minutes would it take to have a total of 4,096 cells?
_______ minutes
Answer: 240 minutes
Explanation:
First, convert the cells into the exponential form.
The exponential form of 4096 is 2 × 2 × 2 × 2 × 2 × 2× 2 × 2× 2 × 2× 2 × 2 = 212
Multiply the power with 20
12 × 20 = 240
Thus it would take 240 minutes to have a total of 4,096 cells
Exponents – Page No. 361
Use one or more exponents to write the expression.
Question 1.
6 × 6
Type below:
_____________
Answer:
The number 6 is used as a repeated factor.
6 is used as a factor 2 times.
Now write the base and exponent for 6 × 6 = 62
Question 2.
11 × 11 × 11 × 11
Type below:
_____________
Answer:
The number 11 is used as a repeated factor.
11 is used as a factor 4 times.
Now write the base and exponent for 11 × 11 × 11 × 11 = 114
Question 3.
9 × 9 × 9 × 9 × 7 × 7
Type below:
_____________
Answer:
The number 9 and 7 is used as a repeated factor.
9 is used as a factor 4 times and 7 is used 2 times.
Now write the base and exponent for 9 × 9 × 9 × 9 × 7 × 7 = 94 × 72
Question 4.
64
_______
Answer:
The repeated factor is 6.
Write the factor 4 times.
The value of 64 is 6 × 6 × 6 × 6 = 1296
Question 5.
16
_______
Answer:
The repeated factor is 1.
Write the factor 6 times.
The value of 16 is 1 × 1 × 1 × 1 × 1 × 1 = 1
Question 6.
105
_______
Answer:
The repeated factor is 10.
Write the factor 5 times.
The value of 105 is 10 × 10 × 10 × 10 × 10 = 1,00,000
Question 7.
Write 144 with an exponent by using 12 as the base.
Type below:
_____________
Answer: 12 × 12 = 122
The exponential form of 144 is 12 × 12 = 122
Question 8.
Write 343 with an exponent by using 7 as the base.
Type below:
_____________
Answer: The exponential form of 343 is 7 × 7 × 7 = 73
Question 9.
Each day Sheila doubles the number of push-ups she did the day before. On the fifth day, she does 2 × 2 × 2 × 2 × 2 push-ups. Use an exponent to write the number of push-ups Shelia does on the fifth day.
Type below:
_____________
Answer:
The number 2 is the repeated factor.
2 is repeated 5 times.
The exponential form of 2 × 2 × 2 × 2 × 2 is 25
Question 10.
The city of Beijing has a population of more than 107 people. Write 107 without using an exponent.
_______
Answer:
The repeated factor is 10.
Write the factor 7 times.
The value of 107 is 10 × 10 × 10 × 10 × 10 × 10 × 10 = 10,000,000
Question 11.
Explain what the expression 45 means and how to find its value.
Type below:
_____________
Answer:
The repeated factor is 4.
Write the factor 5 times.
The value of 45 is 4 × 4 × 4 × 4 × 4 = 1024
Lesson Check – Page No. 362
Question 1.
The number of games in the first round of a chess tournament is equal to 2 × 2 × 2 × 2 × 2 × 2. Write the number of games using an exponent.
Type below:
_____________
Answer: 26
Explanation:
The number 2 is the repeated factor.
2 is repeated 6 times.
2 × 2 × 2 × 2 × 2 × 2 = 26
Question 2.
The number of gallons of water in a tank at an aquarium is equal to 83. How many gallons of water are in the tank?
_______ gallons
Answer: 512 gallons
Explanation:
The repeated factor is 8.
Write the factor 3 times.
The value of 83 is 8 × 8 × 8 = 512 gallons
Therefore there are 512 gallons of water in the tank.
Spiral Review
Question 3.
The table shows the amounts of strawberry juice and lemonade needed to make different amounts of strawberry lemonade. Name another ratio of strawberry juice to lemonade that is equivalent to the ratios in the table.
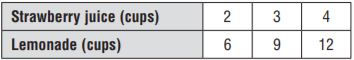
Type below:
_____________
Answer: 5 : 15
Explanation:
By using the above table we can find the ratio of strawberry juice to lemonade.
2 : 6 = 1 : 3
The ratio of strawberry juice to lemonade next to 4 : 12 is 5 : 15
Question 4.
Which percent is equivalent to the fraction \(\frac{37}{50}\)?
_______ %
Answer: 74%
Explanation:
\(\frac{37}{50}\) × 100
0.74 × 100 = 74
Thus 74% is equivalent to the fraction \(\frac{37}{50}\)
Question 5.
How many milliliters are equivalent to 2.7 liters?
_______ milliliters
Answer: 2700 milliliters
Explanation:
Convert from liters to milliliters.
1 liter = 1000 milliliters
2.7 liters = 2.7 × 1000 milliliters = 2700 milliliters
2.7 liters is equivalent to 2700 milliliters.
Question 6.
Use the formula d = rt to find the distance traveled by a car driving at an average speed of 50 miles per hour for 4.5 hours.
_______ miles
Answer: 225 miles
Explanation:
Given,
r = 50 miles/hour
t = 4.5 hours
Use the formula d = rt
d = 50 × 4.5 = 225 miles
Thus the distance traveled by a car driving at an average speed of 50 miles per hour for 4.5 hours is 225 miles.
Share and Show – Page No. 365
Question 1.
Evaluate the expression 9 + (52 − 10)
_______
Answer: 24
Explanation:
First write the square for 52
52 is 25
Now simplify the expression 9 + (25 – 10)
9 + 15 = 24
So, 9 + (52 − 10) = 24
Evaluate the expression.
Question 2.
6 + 33 ÷ 9
_______
Answer: 9
Explanation:
6 + 33 ÷ 9
6 + (33 ÷ 9)
Write the factor for 33
33 = 3 × 3 × 3 = 27
6 + (27 ÷ 9)
27 ÷ 9 = 3
6 + 3 = 9
Thus 6 + 33 ÷ 9 = 9
Question 3.
(15 − 3)2 ÷ 9
_______
Answer: 16
Explanation:
First subtract 15 – 3 = 12
(12)2 ÷ 9
(12)2 = 12 × 12 = 144
144 ÷ 9
9 divides 144 16 times.
144 ÷ 9 = 16
Thus (15 − 3)2 ÷ 9 = 16
Question 4.
(8 + 92) − 4 × 10
_______
Answer: 49
Explanation:
First multiply 9 × 9 = 81
(8 + 81) – (4 × 10)
Multiply 4 and 10.
4 × 10 = 40
(8 + 81) – (40)
89 – 40 = 49
(8 + 92) − 4 × 10 = 49
On Your Own
Evaluate the expression
Question 5.
10 + 62 × 2 ÷ 9
_______
Answer: 18
Explanation:
10 + (62 × 2) ÷ 9
Multipley 6 × 6 = 36
10 + (36 × 2) ÷ 9
Multiply 36 and 2 and then divide by 9.
10 + (72 ÷ 9)
10 + 8 = 18
So, 10 + 62 × 2 ÷ 9 = 18
Question 6.
62 − (23 + 5)
_______
Answer: 23
Explanation:
The value of 62 is 6 × 6 = 36
The value of 23 is 2 × 2 × 2 = 8
36 – (8 + 5)
36 – 13 = 23
Thus the answer for the expression for 62 − (23 + 5) is 23.
Question 7.
16 + 18 ÷ 9 + 34
_______
Answer: 99
Explanation:
16 + (18 ÷ 9) + 34
First divide 18 by 9
16 + 2 + 34
18 + 34
The value of 34 is 3 × 3 × 3 × 3 = 81
18 + 81 = 99
Thus the answer for the expression 16 + (18 ÷ 9) + 34 is 99.
Place parentheses in the expression so that it equals the given value.
Question 8.
102 − 50 ÷ 5
value: 10
Type below:
_____________
Answer: 10
Explanation:
102 − 50 ÷ 5
The factor of 102 is 10 × 10 = 100
(102 − 50) ÷ 5
50 ÷ 5 = 10
102 − 50 ÷ 5 = 10
The value of 102 − 50 ÷ 5 = 10
Question 9.
20 + 2 × 5 + 41
value: 38
Type below:
_____________
Answer: 38
Explanation:
20 + 2 × 5 + 41
The value of 41 is 4.
20 + 2 × (5 + 4)
20 + 2 × 9
Now multiply 2 and 9.
20 + 18 = 38
The value of 20 + 2 × 5 + 41 = 38
Question 10.
28 ÷ 22 + 3
value: 4
Type below:
_____________
Answer: 4
Explanation:
28 ÷ 22 + 3
28 ÷ (22 + 3)
The value of 22 is 4
28 ÷ (4 + 3)
28 ÷ 7 = 4
The value of 28 ÷ 22 + 3 is 4.
Problem Solving + Applications – Page No. 366
Use the table for 11–13.
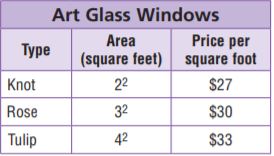
Question 11.
Write an Expression To find the cost of a window, multiply its area in square feet by the price per square foot. Write and evaluate an expression to find the cost of a knot window
$ _______
Answer: 108
Explanation:
To find the cost of the knot window multiply the area with the price per square foot.
Area per square feet is 22
Price per square foot is $27
Cost = 22 × 27 = 4 × 27 = 108
Thus the cost of a knot window is $108
Question 12.
A builder installs 2 rose windows and 2 tulip windows. Write and evaluate an expression to find the combined area of the windows.
_______ square feet
Answer: 50
Explanation:
The area of rose window is 32
The area of tulip windows is 22
Combines area of rose and tulip window is 32 + 22 = 52
52 × 2 = 25 × 2 = 50
Thus the area of the combined windows is 50 square feet.
Question 13.
DeShawn bought a tulip window. Emma bought a rose window. Write and evaluate an expression to determine how much more DeShawn paid for his window than Emma paid for hers.
$ _______
Answer: 258
Explanation:
Given that, DeShawn bought a tulip window.
DeShawn bought it for 42 × $33 = 16 × $33 = 528
Emma bought a rose window
Emma bought it for 32 × 30 = 9 × 30 = 270
$528 – $270 = $258
DeShawn paid $258 for his window than Emma paid for hers.
Question 14.
What’s the Error? Darius wrote 17 − 22 = 225. Explain his error.
Type below:
_____________
Answer: 17 – 4 is actually 13 but not 225.
Question 15.
Ms. Hall wrote the expression 2 × (3 + 5)2÷ 4 on the board. Shyann said the first step is to evaluate 52. Explain Shyann’s mistake. Then evaluate the expression
_______
Answer: 32
Explanation:
2 × (3 + 5)2÷ 4
First, add 3 and 5.
2 × (8)2÷ 4
The square of 8 × 8 is 64.
2 × (64 ÷ 4) = 2 × 16 = 32
Evaluate Expressions Involving Exponents – Page No. 367
Evaluate the expression.
Question 1.
5 + 17 − 102 ÷ 5
_______
Answer: 2
Explanation:
5 + 17 – (100 ÷ 5)
Divide 100 by 5
(5 + 17) – 20
22 – 20 = 2
So, the value for the expression 5 + 17 − 102 ÷ 5 = 2
Question 2.
72 − 32 × 4
_______
Answer: 13
Explanation:
72 − 32 × 4
72 − (32 × 4)
72 − (9 × 4)
49 – 36 = 13
Thus, 72 − 32 × 4 = 13
Question 3.
24 ÷ (7 − 5)
_______
Answer: 8
Explanation:
24 ÷ (7 − 5)
24 ÷ 2
24 = 2 × 2 × 2 × 2 = 16
16 ÷ 2 = 8
24 ÷ (7 − 5) = 8
Question 4.
(82 + 36) ÷ (4 × 52)
_______
Answer: 1
Explanation:
(82 + 36) ÷ (4 × 52)
82 = 8 × 8 = 64
52 = 5 × 5 = 25
(64 + 36) ÷ (4 × 25)
100 ÷ 100 = 1
So, (82 + 36) ÷ (4 × 52) = 1
Question 5.
12 + 21 ÷ 3 + (22 × 0)
_______
Answer: 19
Explanation:
12 + 21 ÷ 3 + 0
12 + (21 ÷ 3)
12 + 7 = 19
12 + 21 ÷ 3 + (22 × 0) = 19
Question 6.
(12 − 8)3 − 24 × 2
_______
Answer: 16
Explanation:
(12 − 8)3 − 24 × 2 = (4)3 − 24 × 2
64 – (24 × 2)
= 64 – 48 = 16
(12 − 8)3 − 24 × 2 = 16
Place parentheses in the expression so that it equals the given value.
Question 7.
12 × 2 + 23
value: 120
Type below:
_____________
Answer:
12 × (2 + 23)
12 × (2 + 8)
12 × 10 = 120
12 × 2 + 23 = 120
Question 8.
72 + 1 − 5 × 3
value: 135
Type below:
_____________
Answer:
(72 + 1 − 5) × 3
(49 + 1 – 5) × 3
(50 – 5) × 3
45 × 3 = 135
72 + 1 − 5 × 3 = 135
Problem Solving
Question 9.
Hugo is saving for a new baseball glove. He saves $10 the first week, and $6 each week for the next 6 weeks. The expression 10 + 62 represents the total amount in dollars he has saved. What is the total amount Hugo has saved?
$ _______
Answer: $46
Explanation:
Hugo is saving for a new baseball glove.
He saves $10 the first week, and $6 each week for the next 6 weeks.
The expression 10 + 62 represents the total amount in dollars he has saved.
10 + 62 = 10 + 36 = 46
total amount Hugo has saved is $46
Question 10.
A scientist placed 5 fish eggs in a tank. Each day, twice the number of eggs from the previous day hatch. The expression 5 × 26 represents the number of eggs that hatch on the seventh day. How many eggs hatch on the seventh day?
_______ eggs
Answer: 320 eggs
Explanation:
A scientist placed 5 fish eggs in a tank.
Each day, twice the number of eggs from the previous day hatch.
The expression 5 × 26 represents the number of eggs that hatch on the seventh day.
5 × 26 = 5 × 64 = 320 eggs
Therefore 320 eggs hatch on the seventh day.
Question 11.
Explain how you could determine whether a calculator correctly performs the order of operations.
Type below:
_____________
Answer: Create a problem that must use the order of operations and isn’t solved by just left to right. Solve it going left to right. Then solve it using the order of operations. Solve it on the calculator. Your answer on the calculator will match the one using the order of operations.
Lesson Check – Page No. 368
Question 1.
Ritchie wants to paint his bedroom ceiling and four walls. The ceiling and each of the walls are 8 feet by 8 feet. A gallon of paint covers 40 square feet. Write an expression that can be used to find the number of gallons of paint Ritchie needs to buy.
Type below:
_____________
Answer:
Ritchie wants to paint his bedroom ceiling and four walls.
The ceiling and each of the walls are 8 feet by 8 feet.
A gallon of paint covers 40 square feet.
8 × 8 × (4 + 1) ÷ 40
82 (4 + 1) ÷ 40
Thus the expression that can be used to find the number of gallons of paint Ritchie needs to buy is 82 (4 + 1) ÷ 40
Question 2.
A Chinese restaurant uses about 225 pairs of chopsticks each day. The manager wants to order a 30-day supply of chopsticks. The chopsticks come in boxes of 750 pairs. How many boxes should the manager order?
_______ boxes
Answer: 9 boxes
Explanation:
A Chinese restaurant uses about 225 pairs of chopsticks each day.
The manager wants to order a 30-day supply of chopsticks.
Multiply the number of pairs with the number of days
225 × 30 = 6750
The chopsticks come in boxes of 750 pairs.
Now divide the number of chopsticks by the number of pairs.
6750 ÷ 750 = 9 boxes.
Spiral Review
Question 3.
Annabelle spent $5 to buy 4 raffle tickets. How many tickets can she buy for $20?
_______ tickets
Answer: 16 tickets
Explanation:
Annabelle spent $5 to buy 4 raffle tickets.
To find the number of tickets she can buy for $20.
($20 ÷ $5) × 4
4 × 4 = 16 tickets
That means she can buy 16 tickets for $20.
Question 4.
Gavin has 460 baseball players in his collection of baseball cards, and 15% of the players are pitchers. How many pitchers are in Gavin’s collection?
_______ pitchers
Answer: 69 pitchers
Explanation:
Gavin has 460 baseball players in his collection of baseball cards, and 15% of the players are pitchers.
The decimal form of 15% is 0.15
Now multiply 460 with 0.15
460 × 0.15 = 69.00
Thus there are 69 pitchers in Gavin’s collection.
Question 5.
How many pounds are equivalent to 40 ounces?
_______ pounds
Answer: 2.5 pounds
Explanation:
Convert from ounces to pounds.
1 pound = 16 ounces
1 ounce = 1/16 pound
40 ounces = 40 × 1/16 pound
40 ounces = 2.5 pounds
Thus, 2.5 pounds are equivalent to 40 ounces
Question 6.
List the expressions in order from least to greatest.
15 33 42 81
Type below:
_____________
Answer:
15 33 42 81
15 = 1 × 1 × 1 × 1 × 1 = 1
33 = 3 × 3 × 3 = 27
42 = 4 × 4 = 16
81 = 8
Thus the order from least to greatest.
15 81 42 33
Share and Show – Page No. 371
Question 1.
Write an algebraic expression for the product of 6 and p.
What operation does the word “product” indicate?
Type below:
_____________
Answer: 6 × p
Explanation:
The word product indicates multiplication.
Multiply 6 with p.
The algebraic expression for the product of 6 and p is 6 × p.
Write an algebraic expression for the word expression.
Question 2.
11 more than e
Type below:
_____________
Answer: 11 + e
Explanation:
The word more than indicates addition operation.
So, the algebraic expression is 11 + e
Question 3.
9 less than the quotient of n and 5
Type below:
_____________
Answer: 9 – (n ÷ 5)
Explanation:
The word “less than” indicates subtraction and the “quotient” indicates division.
So, the expression is 9 – (n ÷ 5)
On Your Own
Write an algebraic expression for the word expression.
Question 4.
20 divided by c
Type below:
_____________
Answer: 20 ÷ c
Explanation:
Here we have to divide 20 by c.
The expression is 20 ÷ c
Question 5.
8 times the product of 5 and t
Type below:
_____________
Answer: 8 × (5t)
Explanation:
The word times indicate multiplication and the product indicates multiplication.
Here we have to multiply 8 with 5 and t.
Thus the expression is 8 × 5 × t = 8 × 5t
Question 6.
There are 12 eggs in a dozen. Write an algebraic expression for the number of eggs in d dozen.
Type below:
_____________
Answer: 12d
Explanation:
Given,
There are 12 eggs in a dozen.
d represents number of eggs in dozen
So, we have to multiply 12 with d.
Thus the algebraic expression is 12d.
Question 7.
A state park charges a $6.00 entry fee plus $7.50 per night of camping. Write an algebraic expression for the cost in dollars of entering the park and camping for n nights.
Type below:
_____________
Answer: $6.00 + $7.50 n
Explanation:
Given that, A state park charges a $6.00 entry fee plus $7.50 per night of camping.
Find the camping for n nights. The product of $7.50 camping for n nights.
$7.50 × n
Now add park charges to the camping nights.
$6.00 + $7.50 n
Thus the algebraic expression for the cost in dollars of entering the park and camping for n nights is $6.00 + $7.50 n
Question 8.
Look for Structure At a bookstore, the expression 2c + 8g gives the cost in dollars of c comic books and g graphic novels. Next month, the store’s owner plans to increase the price of each graphic novel by $3. Write an expression that will give the cost of c comic books and g graphic novels next month.
Type below:
_____________
Answer: 2c + 11g
Explanation:
Look for Structure At a bookstore, the expression 2c + 8g gives the cost in dollars of c comic books and g graphic novels.
Next month, the store’s owner plans to increase the price of each graphic novel by $3.
Here we have to add $3 to 8 g = 3g + 8g = 11g
Sum of cost of c comic books and g graphic novels
Thus the expression is 2c + 11g
Unlock the Problem – Page No. 372
Question 9.
Martina signs up for the cell phone plan described at the right. Write an expression that gives the total cost of the plan in dollars if Martina uses it for m months.

a. What information do you know about the cell phone plan?
Type below:
_____________
Answer: Pay a low monthly fee of $50. Receive $10 off your first month’s fee.
Question 9.
b. Write an expression for the monthly fee in dollars for m months.
Type below:
_____________
Answer:
M is the number of months.
50 × m
Given that $10 off on first-month fee.
50m + (50-10)
50m + $40
Question 9.
c. What operation can you use to show the discount of $10 for the first month?
Type below:
_____________
Answer: We have to use subtraction operations to show a discount of $10 for the first month.
Question 9.
d. Write an expression for the total cost of the plan in dollars for m months
Type below:
_____________
Answer: 50m + 40
Question 10.
A group of n friends evenly share the cost of dinner. The dinner costs $74. After dinner, each friend pays $11 for a movie. Write an expression to represent what each friend paid for dinner and the movie.
Type below:
_____________
Answer: 74 ÷ n + 11n
Explanation:
Given,
A group of n friends evenly share the cost of dinner.
The dinner costs $74. After dinner, each friend pays $11 for a movie.
The word share represents the division operation.
That means we have to divide 74 by n.
74 ÷ n
After that n friends paid $11 for movie
Multiply 11 with n.
Thus the expression to represent what each friend paid for dinner and the movie is 74 ÷ n + 11n
Question 11.
A cell phone company charges $40 per month plus $0.05 for each text message sent. Select the expressions that represent the cost in dollars for one month of cell phone usage and sending m text messages. Mark all that apply.
Options:
a. 40m + 0.05
b. 40 + 0.05m
c. 40 more than the product of 0.05 and m
d. the product of 40 and m plus 0.05
Answer: 40 + 0.05m
Explanation:
A cell phone company charges $40 per month plus $0.05 for each text message sent.
Let m represent the messages sent.
40m + 0.05m
Thus the answer is option B.
Write Algebraic Expressions – Page No. 373
Write an algebraic expression for the word expression.
Question 1.
13 less than p
Type below:
_____________
Answer: 13 – p
Explanation:
Less than is nothing but subtraction.
So the expression for 13 less than p is 13 – p
Question 2.
the sum of x and 9
Type below:
_____________
Answer: x + 9
Explanation:
The sum is nothing but an addition.
Thus the expression for the sum of x and 9 is x + 9.
Question 3.
6 more than the difference of b and 5
Type below:
_____________
Answer: 6 + (b – 5)
Explanation:
More than is nothing but addition and difference means subtraction.
The expression for 6 more than the difference of b and 5 is 6 + (b – 5)
Question 4.
the sum of 15 and the product of 5 and v
Type below:
_____________
Answer: 15 + 5v
Explanation:
Product is nothing but multiplication and sum is nothing but an addition.
So, the expression for the sum of 15 and the product of 5 and v is 15 + 5 × v
Question 5.
the difference of 2 and the product of 3 and k
Type below:
_____________
Answer: 2 – 3k
Explanation:
The difference means subtraction and Product is nothing but the multiplication
So, the difference of 2 and the product of 3 and k is 2 – 3 × k
2 – 3k
Question 6.
12 divided by the sum of h and 2
Type below:
_____________
Answer: 12 ÷ h + 2
Explanation:
12 divided by the sum of h and 2
Divide 12 by sum of h and 2.
12 ÷ (h + 2)
Question 7.
the quotient of m and 7
Type below:
_____________
Answer: m ÷ 7
Explanation:
Given the quotient of m and 7
That means we have to divide m by 7.
Thus the answer is m ÷ 7
Question 8.
9 more than 2 multiplied by f
Type below:
_____________
Answer: 9 + 2f
Explanation:
9 more than 2 multiplied by f
We have to add 9 to 2 × f
So, the expression is 9 + 2f
Question 9.
6 minus the difference of x and 3
Type below:
_____________
Answer: 6 – (x – 3)
Explanation:
First, subtract 3 from x
The expression for 6 minus the difference of x and 3 is 6 – (x – 3)
Question 10.
10 less than the quotient of g and 3
Type below:
_____________
Answer: 10 – (g ÷ 3)
Explanation:
The quotient of g and 3 is nothing but divide g by 3
g ÷ 3
Now subtract g ÷ 3 from 10.
So, the expression for 10 less than the quotient of g and 3 is 10 – (g ÷ 3)
Question 11.
the sum of 4 multiplied by a and 5 multiplied by b
Type below:
_____________
Answer: 4a + 5b
Explanation:
First, multiply 4 with a and then multiply 5 with b
After that add both the expressions.
4a + 5b
So, the sum of 4 multiplied by a and 5 multiplied by b is 4a + 5b
Question 12.
14 more than the difference of r and s
Type below:
_____________
Answer: 14 + (r – s)
Explanation:
Subtract r and s
And then add 14 to that r -s
14 + (r – s)
Problem Solving
Question 13.
Let h represent Mark’s height in inches. Suzanne is 7 inches shorter than Mark. Write an algebraic expression that represents Suzanne’s height in inches.
Type below:
_____________
Answer: h – 7
Explanation:
Let h represent Mark’s height in inches. Suzanne is 7 inches shorter than Mark.
That means we have to subtract 7 from h.
i.e., h – 7
Thus Suzanne’s height is h – 7 inches.
Question 14.
A company rents bicycles for a fee of $10 plus $4 per hour of use. Write an algebraic expression for the total cost in dollars for renting a bicycle for h hours.
Type below:
_____________
Answer: 10 + 4h
Explanation:
A company rents bicycles for a fee of $10 plus $4 per hour of use.
Multiply 4 with hours
And then 10 to 4h
10 + 4h
Thus the total cost in dollars for renting a bicycle for h hours is 10 + 4h
Question 15.
Give an example of a real-world situation involving two unknown quantities. Then write an algebraic expression to represent the situation.
Type below:
_____________
Answer:
Cooper bikes so many miles per day and does it for 7 months.
The expression for the question is 6m × 7
Lesson Check – Page No. 374
Question 1.
The female lion at a zoo weighs 190 pounds more than the female cheetah. Let c represent the weight in pounds of the cheetah. Write an expression that gives the weight in pounds of the lion.
Type below:
_____________
Answer: c + 190
Explanation:
Given that, The female lion at a zoo weighs 190 pounds more than the female cheetah.
Let c represent the weight in pounds of the cheetah.
We have to add 190 to the weight in pounds of the cheetah.
That means c + 190
Thus the expression that gives the weight in pounds of the lion is c + 190.
Question 2.
Tickets to a play cost $8 each. Write an expression that gives the ticket cost in dollars for a group of g girls and b-boys.
Type below:
_____________
Answer: 8 × (g + b)
Explanation:
First add girls group and boys group.
g + b
And then multiply 8 with the group of girls and boys.
8 × (g + b)
So, the expression that gives the ticket cost in dollars for a group of g girls and b-boys is 8 × (g + b).
Spiral Review
Question 3.
A bottle of cranberry juice contains 32 fluid ounces and costs $2.56. What is the unit rate?
$ _______ per fluid ounce
Answer: 0.08
Explanation:
A bottle of cranberry juice contains 32 fluid ounces and costs $2.56.
Divide the number of fluid ounces by the cost.
32 ÷ $2.56
32/2.56 = 0.08
The unit rate is 0.08 per fluid ounce.
Question 4.
There are 32 peanuts in a bag. Elliott takes 25% of the peanuts from the bag. Then Zaire takes 50% of the remaining peanuts. How many peanuts are left in the bag?
_______ peanuts
Answer: 12
Explanation:
First, we have to find 25% of 32.
25% of 32 its 0.25 × 32=8
Now we have to subtract 32 and 8
32 – 8=24
Now we have to find 50% of 24
50% of 24 = 12
24-12=12.
Thus 12 peanuts are left in the bag.
Question 5.
Hank earns $12 per hour for babysitting. How much does he earn for 15 hours of babysitting?
$ _______
Answer: 180
Explanation:
Hank earns $12 per hour for babysitting.
Multiply $12 with 15
12 × 15 = $180
He earned $180 for 15 hours of babysitting.
Question 6.
Write an expression using exponents that represent the area of the figure in square centimeters
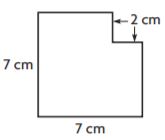
Type below:
_____________
Answer: 72 – 22
Explanation:
The area of the square is 7 cm × 7 cm = 72
The area of the square is 2 cm × 2 cm = 22
Now subtract a small square from the large square.
The expression that represents the area of the figure is 72 – 22
Share and Show – Page No. 377
Identify the parts of the expression. Then, write a word expression for the numerical or algebraic expression.
Question 1.
7 × (9 ÷ 3)
Type below:
_____________
Answer:
The quotient of 9 and 3 and then multiply with 7.
Word expression: Product of 7 with the quotient of 9 and 3.
Question 2.
5m + 2n
Type below:
_____________
Answer:
Product of 5 and m and product of 2 and n
Now add both the product of 5 and m and 2 and n.
Word Expression: Product of 5 and m plus the product of 2 and n.
On Your Own
Practice: Copy and Solve Identify the parts of the expression. Then write a word expression for the numerical or algebraic expression.
Question 3.
8 + (10 − 7)
Type below:
_____________
Answer:
Subtraction is the difference between 10 and 7. Addition to the subtraction of 10 and 7.
Word expression: Add 8 to the difference between 10 and 7.
Question 4.
1.5 × 6 + 8.3
Type below:
_____________
Answer:
The addition is the sum of 6 and 8.3 and then multiply the sum to 1.5.
Word expression: 1.5 times the sum of 6 and 8.3
Question 5.
b + 12x
Type below:
_____________
Answer:
Product of 12 and x. Add b to the product of 12 and x.
Word expression: Sum of b to the product of 12 and x.
Question 6.
4a ÷ 6
Type below:
_____________
Answer:
The division is the quotient of 4a and 6. Multiply 4 and a. The expression is the product of 4 and a divided by 6.
Word expression: The quotient of the product 4 and a and 6.
Identify the terms of the expression. Then, give the coefficient of each term.
Question 7.
k − \(\frac{1}{3}\)d
Type below:
_____________
Answer:
The terms of the expression are k and \(\frac{1}{3}\)d
Coefficients – 1 and \(\frac{1}{3}\)
Question 8.
0.5x + 2.5y
Type below:
_____________
Answer:
The terms of the expression are 0.5x and 2.5y
Coefficients – 0.5 and 2.5
Question 9.
Connect Symbols and Words Ava said she wrote an expression with three terms. She said the first term has the coefficient 7, the second term has the coefficient 1, and the third term has the coefficient 0.1. Each term involves a different variable. Write an expression that could be the expression Ava wrote
Type below:
_____________
Answer:
Connect Symbols and Words Ava said she wrote an expression with three terms.
She said the first term has the coefficient 7, the second term has the coefficient 1, and the third term has the coefficient 0.1.
The expression for the first term is 7x
The expression for the second term is 1y
The expression for the third term is 0.1z
7x + y + 0.1z
Problem Solving + Applications – Page No. 378
Use the table for 10–12.
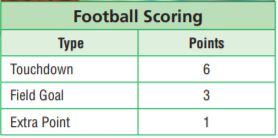
Question 10.
A football team scored 2 touchdowns and 2 extra points. Their opponent scored 1 touchdown and 2 field goals. Write a numerical expression for the points scored in the game.
Type below:
_____________
Answer:
A football team scored 2 touchdowns and 2 extra points.
2 touchdowns = 2 × 6
2 extra points = 2 × 1
Their opponent scored 1 touchdown and 2 field goals.
1 touchdown = 1 × 6
2 field goals = 2 × 3
Thue the numerical expression is 12 + 2 + 6 + 6
14 + 12
The numerical expression for the points scored in the game is 14 + 12.
Question 11.
Write an algebraic expression for the number of points scored by a football team that makes t touchdowns, f field goals, and e extra points
Type below:
_____________
Answer: 6t + 3f + e
Explanation:
The number of points scored by a football team that makes t touchdowns, f field goals, and e extra points.
The table shows that touchdown has 6 points, field goal has 3 points and extra point has 1 point.
So we need to add all the points to make the expressions
That means 6t + 3f + e
Question 12.
Identify the parts of the expression you wrote in Exercise 11.
Type below:
_____________
Question 13.
Give an example of an expression involving multiplication in which one of the factors is a sum. Explain why you do or do not need parentheses in your expression
Type below:
_____________
Answer: 6 × 2 + 3
In this expression, there is no need for parentheses because there are no exponents or multiple operations.
Question 14.
Kennedy bought a pounds of almonds at $5 per pound and p pounds of peanuts at $2 per pound. Write an algebraic expression for the cost of Kennedy’s purchase.
Type below:
_____________
Answer: 5 + 2p = x
Explanation:
Kennedy bought a pounds of almonds at $5 per pound and p pounds of peanuts at $2 per pound.
We have to multiply p with $2 per pound.
The algebraic expression for the cost of Kennedy’s purchase is the sum of 5 and the product of p and 2
Thus the expression is 5 + 2p = x
Identify Parts of Expressions – Page No. 379
Identify the parts of the expression. Then write a word expression for the numerical or algebraic expression.
Question 1.
(16 − 7) ÷ 3
Type below:
_____________
Answer:
Subtraction is the difference between 16 and 7. The division is the quotient of the difference and 3
Word expression: the quotient of the difference 16 and 7 and 3.
Question 2.
8 + 6q + q
Type below:
_____________
Answer:
Sum of 8 and the product of 6 and q added to q.
Addition – Sum of 8 plus the product of 6 and q plus q.
Addition – 6 times q and the sum of q.
Multiply – the product of 6 and q.
Identify the terms of the expression. Then give the coefficient of each term.
Question 3.
11r + 7s
Type below:
_____________
Answer:
The terms of the expression are 11r and 7s
The coefficient of each term is 11 and 7.
Question 4.
6g − h
Type below:
_____________
Answer:
The terms of the expression are 6g and h
The coefficient of each term is 6 and 1.
Problem Solving
Question 5.
Adam bought granola bars at the store. The expression 6p + 5n gives the number of bars in p boxes of plain granola bars and n boxes of granola bars with nuts. What are the terms of the expression?
Type below:
_____________
Answer:
Adam bought granola bars at the store.
The expression 6p + 5n gives the number of bars in p boxes of plain granola bars and n boxes of granola bars with nuts.
The terms of the expression are 6p and 5n.
Question 6.
In the sixth grade, each student will get 4 new books. There is one class of 15 students and one class of 20 students. The expression 4 × (15 + 20) gives the total number of new books. Write a word expression for the numerical expression.
Type below:
_____________
Answer:
In the sixth grade, each student will get 4 new books.
There is one class of 15 students and one class of 20 students.
The expression 4 × (15 + 20) gives the total number of new books.
The product of 4 the sum of 15 and 20.
Question 7.
Explain how knowing the order of operations helps you write a word expression for a numerical or algebraic expression.
Type below:
_____________
Answer: Because if you don’t know and use the order of operations you can get an entirely different answer.
Lesson Check – Page No. 380
Question 1.
A fabric store sells pieces of material for $5 each. Ali bought 2 white pieces and 8 blue pieces. She also bought a pack of buttons for $3. The expression 5 × (2 + 8) + 3 gives the cost in dollars of Ali’s purchase. How can you describe the term (2 + 8) in words?
Type below:
_____________
Answer: the sum of 2 and 8
Explanation:
A fabric store sells pieces of material for $5 each.
Ali bought 2 white pieces and 8 blue pieces.
She also bought a pack of buttons for $3.
The expression 5 × (2 + 8) + 3 gives the cost in dollars of Ali’s purchase.
The word expression for the term 2 + 8 is the sum of 2 and 8.
Question 2.
A hotel offers two different types of rooms. The expression k + 2f gives the number of beds in the hotel where k is the number of rooms with a king-size bed and f is the number of rooms with 2 full-size beds. What are the terms of the expression?
Type below:
_____________
Answer: k and 2f
Explanation:
The terms for the expression k + 2f is k and 2f.
Spiral Review
Question 3.
Meg paid $9 for 2 tuna sandwiches. At the same rate, how much does Meg pay for 8 tuna sandwiches?
$ _______
Answer: 36
Explanation:
Meg paid $9 for 2 tuna sandwiches.
To find how much does Meg pay for 8 tuna sandwiches
2 – $9
8 -?
$9 × 8/2 = 72/2 = 36
Thus Meg pays $36 for 8 tuna sandwiches.
Question 4.
Jan is saving for a skateboard. She has saved $30 already, which is 20% of the total price. How much does the skateboard cost?
$ _______
Answer: 150
Explanation:
Jan is saving for a skateboard. She has saved $30 already, which is 20% of the total price.
Divide $30 by 20%
30 ÷ 20%
30 ÷ 20 × 1/100
30 ÷ 1/5
30 × 5 = 150
Thus the cost of the skateboard is $150.
Question 5.
It took Eduardo 8 hours to drive from Buffalo, NY, to New York City, a distance of about 400 miles. Find his average speed.
_______ miles per hour
Answer: 50
Explanation:
Given,
It took Eduardo 8 hours to drive from Buffalo, NY, to New York City, a distance of about 400 miles.
We can use the formula d = rt
r = d/t
r = 400 miles/8 hours
r = 50 miles per hour
Question 6.
Write an expression that represents the value, in cents, of n nickels.
Type below:
_____________
Answer: 0.05n
Explanation:
An expression does not have an equal sign.
Since the value of a nickel is 5 cents and you want to find out the value of n nickels (which means if you had any number of nickels) the expression would be
.05n
Share and Show – Page No. 383
Question 1.
Evaluate 5k + 6 for k = 4.
_______
Answer: 26
Explanation:
The expression is 5k + 6
Substitute the value k = 4
5(4) + 6 = 20 + 6 = 26
5k + 6 = 26
Evaluate the expression for the given value of the variable.
Question 2.
m − 9 for m = 13
_______
Answer: 4
Explanation:
m – 9
Substitute the value of m in the expression
13 – 9 = 4
Thus m – 9 = 4
Question 3.
16 − 3b for b = 4
_______
Answer: 4
Explanation:
Given the expression 16 – 3b
Now substitute the value of b in the expression.
16 – 3b = 16 – 3(4) = 16 – 12 = 4
16 – 3b = 4
Question 4.
p2 + 4 for p = 6
_______
Answer: 40
Explanation:
Given the expression p2 + 4
Substitute the value of p in the expression
62 + 4 = 36 + 4 = 40
Thus the value of p2 + 4 is 40.
Question 5.
The formula A = lw gives the area A of a rectangle with length l and width w. What is the area in square feet of a United States flag with a length of 12 feet and a width of 8 feet?
_______ square feet
Answer: 96 square feet
Explanation:
Use the formula A = lw
Length = 12 feet
Width = 8 feet
A = lw
A = 12 feet × 8 feet = 96 square feet
Thus the area of the United States flag is 96 square feet.
On Your Own
Practice: Copy and Solve Evaluate the expression for the given value of the variable.
Question 6.
7s + 5 for s = 3
_______
Answer: 26
Explanation:
Given the expression 7s + 5
Substitute the value of S in the above expression
7(3) + 5 = 21 + 5 = 26
Question 7.
21 − 4d for d = 5
_______
Answer: 1
Explanation:
Given the expression 21 – 4d
Substitute the value d = 5 in the above expression
21 – 4(5) = 21 – 20 = 1
Question 8.
(t − 6)2 for t = 11
_______
Answer: 25
Explanation:
Given the expression (t − 6)2
Substitute the value t = 11
Thus (t − 6)2 = (11 − 6)2 = 5 × 5 = 25
9.6 × (2v − 3) for v = 5
_______
Answer: 42
Explanation:
Given the expression 6 × (2v – 3)
Substitute the value of v in the above expression.
6 × (2v – 3) = 6 × (2 × 5 – 3)
6 × (10 – 3)
6 × 7 = 42
Thus the value of 6 × (2v – 3) = 42
Question 10.
2 × (k2 − 2) for k = 6
_______
Answer: 68
Explanation:
Given the expression 2 × (k2 − 2)
Substitute the value of k in the above expression
2 × (k2 − 2) = 2 × (62 − 2)
2 × (36 – 2) = 2 × 34 = 68
Thus the value of 2 × (k2 − 2) is 68
Question 11.
5 × (f − 32) ÷ 9 for f = 95
_______
Answer: 35
Explanation:
The expression is 5 × (f – 32) ÷ 9
Substitute the value f = 95
5 × (f – 32) ÷ 9 = 5 × (95 – 32) ÷ 9
5 × (63 ÷ 9) = 5 × 7 = 35
The value of 5 × (63 ÷ 9) = 35
Question 12.
The formula P = 4s gives the perimeter P of a square with side length s. How much greater is the perimeter of a square with a side length of 5 \(\frac{1}{2}\) inches than a square with a side length of 5 inches?
_______ inches
Answer: 2 inches
Explanation:
We have to use the formula P = 4s to find the perimeter of the square.
4 × 5 \(\frac{1}{2}\)
Convert the mixed fraction to the improper fraction.
4 × 11/2 = 2 × 11 = 22 inches
4 × 5 inches = 20 inches
To find which has the greater perimeter we have to subtract 20 inches from 22 inches
22 inches – 20 inches = 2 inches
Thus the perimeter of a square with 5 \(\frac{1}{2}\) inches is 2 inches greater than a square with a side length of 5 inches.
Problem Solving + Applications – Page No. 384
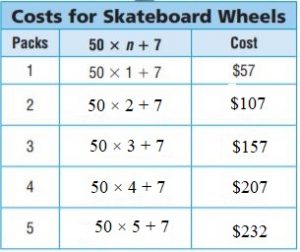
The table shows how much a company charges for skateboard wheels. Each pack of 8 wheels costs $50. Shipping costs $7 for any order. Use the table for 13−15.
Question 13.
Complete the table.
Type below:
_____________
Answer:
Question 14.
A skateboard club has $200 to spend on new wheels this year. What is the greatest number of packs of wheels the club can order?
_______ packs
Answer: 3 packs
Explanation:
A skateboard club has $200 to spend on new wheels this year.
From the above table, we can say that the club can order 3 packs of wheels.
Question 15.
Make Sense of Problems A sporting goods store placed an order for 12 packs of wheels on the first day of each month last year. How much did the sporting goods store spend on these orders last year?
$ _______
Answer: 7284
Explanation:
Make Sense of Problems A sporting goods store placed an order for 12 packs of wheels on the first day of each month last year.
Substitute n = 7 in the expression 50 × n + 7
We get, 50 × 12 + 7
600 + 7 = 607
Now multiply 607 with 12
607 × 12 = 7284
Therefore the sporting goods store spent $7284 on these orders last year.
Question 16.
What’s the Error? Bob used these steps to evaluate 3m − 3 ÷ 3 for m = 8. Explain his error.
3 × 8 − 3 ÷ 3 = 24 − 3 ÷ 3
= 21 ÷ 3
= 7
Type below:
_____________
Answer:
First, he has to subtract 8 and 3. But he first multiplied and then subtracted 24 and 3.
3 × 8 − 3 ÷ 3 = 3 × (8 − 3) ÷ 3
3 × 5 ÷ 3
15 ÷ 3 = 5
Question 17.
The surface area of a cube can be found by using the formula 6s2, where s represents the length of the side of the cube.
The surface area of a cube that has a side length of 3 meters is _____ meters squared.
The surface area of a cube that has a side length meters
of 3 meters is _____________ squared
Answer: 5
Explanation:
The surface area of a cube can be found by using the formula 6s2
he surface area of a cube that has a side length of 3 meters
s2 = 32 = 9
6 × 9 = 54 square meters
Evaluate Algebraic Expressions and Formulas – Page No. 385
Evaluate the expression for the given values of the variables.
Question 1.
w + 6 for w = 11
_______
Answer: 17
Explanation:
Given the expression w + 6
Substitute the value w = 6 in the expression
w + 6 = 11 + 6 = 17
Question 2.
17 − 2c for c = 7
_______
Answer: 3
Explanation:
Substitute the value c = 7 in the given expression
17 – 2(7) = 17 – 14 = 3
Thus the value for 17 – 2c is 3.
Question 3.
b2 − 4 for b = 5
_______
Answer: 21
Explanation:
Substitute the value b = 5 in the expression
b2 − 4 = 52 − 4 = 25 – 4 = 21
Thus the value for the expression b2 − 4 is 21.
Question 4.
(h − 3)2 for h = 5
_______
Answer: 4
Explanation:
We have to substitute the value h = 5
(h − 3)2 = (5 − 3)2
= (2)2 = 4
Therefore the value of (h − 3)2 is 4.
Question 5.
m + 2m + 3 for m = 12
_______
Answer: 39
Explanation:
Given the expression m + 2m + 3
Now substitute the value m = 12 in the above expression.
12 + 2(12) + 3 = 12 + 24 + 3 = 39.
The value for m + 2m + 3 = 39.
Question 6.
4 × (21 − 3h) for h = 5
_______
Answer: 24
Explanation:
Substitute h = 5 in the given expression.
4 × (21 – 3h) = 4 × (21 – 3(5))
4 × (21 – 15) = 4 × 6 = 24
Therefore the value for 4 × (21 – 3h) is 24.
Question 7.
7m − 9n for m = 7 and n = 5
_______
Answer: 4
Explanation:
Substitute the values m = 7 and n = 5 in the above expression.
7m – 9n = 7 × 7 – 9 × 5
= 49 – 45 = 4
Thus 7m – 9n = 4.
Question 8.
d2 − 9k + 3 for d = 10 and k = 9
_______
Answer: 22
Explanation:
Given the expression d2 − 9k + 3
Now substitute d = 10 and k = 9 in the expression.
d2 − 9k + 3 = 102 − 9(9) + 3
100 – 81 + 3 = 22
Thus the value for the expression d2 − 9k + 3 is 22.
Question 9.
3x + 4y ÷ 2 for x = 7 and y = 10
_______
Answer: 41
Explanation:
Substitute the values x = 7 and y = 10 in the expression.
3x + 4y ÷ 2 = 3(7) + 4(10) ÷ 2
21 + 40 ÷ 2 = 21 + 20 = 41
Thus the value for 3x + 4y ÷ 2 is 41.
Problem Solving
Question 10.
The formula P = 2l + 2w gives the perimeter P of a rectangular room with length l and width w. A rectangular living room is 26 feet long and 21 feet wide. What is the perimeter of the room?
_______ feet
Answer: 94 feet
Explanation:
Use the formula of the perimeter of a rectangle P = 2l + 2w
L = 26 feet
W = 21 feet
P = 2(26) + 2(21)
P = 52 feet + 42 feet
P = 94 feet
Therefore the perimeter of a room is 94 feet.
Question 11.
The formula C = 5(F − 32) ÷ 9 gives the Celsius temperature in C degrees for a Fahrenheit temperature of F degrees. What is the Celsius temperature for a Fahrenheit temperature of 122 degrees?
_______ degrees Celsius
Answer: 50
Explanation:
C = 5(F – 32) ÷ 9
We know that F = 122 degrees
Substitute the value of F in the formula
C = 5(122 – 32) ÷ 9
C = 5(90) ÷ 9
C = 450 ÷ 9 = 50
Thus the answer is 50 degrees Celsius.
Question 12.
Explain how the terms variable, algebraic expression, and evaluate are related.
Type below:
_____________
Answer: To evaluate an algebraic expression, you have to substitute a number for each variable and perform the arthematic operations. If we know the variables, we can replace the variables with their values and then evaluate the expression.
Lesson Check – Page No. 386
Question 1.
When Debbie baby-sits, she charges $5 to go to the house plus $8 for every hour she is there. The expression 5 + 8h gives the amount in dollars she charges. How much will she charge to baby-sit for 5 hours?
$ _______
Answer: 45
Explanation:
When Debbie baby-sits, she charges $5 to go to the house plus $8 for every hour she is there. The expression 5 + 8h gives the amount in dollars she charges.
If h = 5 hours
Substitute the value h in the above expression.
5 + 8h = 5 + 8(5) = 5 + 40 = 45
Thus she charges $45 to baby-sit for 5 hours.
Question 2.
The formula to find the cost C in dollars of a square sheet of glass is C = 25s2 where s represents the length of a side in feet. How much will Ricardo pay for a square sheet of glass that is 3 feet on each side?
$ _______
Answer: $225
Explanation:
Use the formula C = 25s2
s represents the length of a side in feet.
s = 3 feet
Substitute the value s in the above formula.
C = 25s2
C = 25(32)
C = 25(9) = 225
Ricardo pays $225 for a square sheet of glass that is 3 feet on each side.
Spiral Review
Question 3.
Evaluate using the order of operations.
\(\frac{3}{4}+\frac{5}{6} \div \frac{2}{3}\)
_______
Answer: 2
Explanation:
\(\frac{3}{4}\) + [/latex]\frac{5}{6}[/latex] ÷ [/latex]\frac{2}{3}[/latex]
[/latex]\frac{5}{6}[/latex] ÷ [/latex]\frac{2}{3}[/latex]
= [/latex]\frac{5}{6}[/latex] × [/latex]\frac{3}{2}[/latex] = [/latex]\frac{15}{12}[/latex] = [/latex]\frac{5}{4}[/latex]
Now convert the improper fraction to the mixed fraction.
[/latex]\frac{5}{4}[/latex] = 1 [/latex]\frac{1}{4}[/latex]
1 [/latex]\frac{1}{4}[/latex] + \(\frac{3}{4}\)
1 + [/latex]\frac{1}{4}[/latex] + \(\frac{3}{4}\) = 1 + 1 = 2
\(\frac{3}{4}+\frac{5}{6} \div \frac{2}{3}\) = 2
Question 4.
Patricia scored 80% on a math test. She missed 4 problems. How many problems were on the test?
_______ problems
Answer: 20
Explanation:
Patricia scored 80% on a math test. She missed 4 problems.
4 ÷ 80%
4 × [/latex]\frac{100}{80}[/latex] = 4 × 5 = 20
Therefore there are 20 questions in the test.
Question 5.
What is the value of 73?
_______
Answer: 343
Explanation:
73 = 7 × 7 × 7 = 49 × 7 = 343
Thus the value of 73 is 343.
Question 6.
James and his friends ordered b hamburgers that cost $4 each and f fruit cups that cost $3 each. Write an algebraic expression for the total cost in dollars of their purchases.
Type below:
_____________
Answer: 4b + 3f
Explanation:
Given that, James and his friends ordered b hamburgers that cost $4 each and f fruit cups that cost $3 each.
Multiply b with $4 and multiply $3 with f
Add 4b and 3f
Thus the expression is 4b + 3f.
Vocabulary – Page No. 387
Choose the best term from the box to complete the sentence.

Question 1.
A(n) _____ tells how many times a base is used as a factor.
Type below:
_____________
Answer: Exponent
An Exponent tells how many times a base is used as a factor.
Question 2.
The mathematical phrase 5+2×18 is an example of a(n) _____.
Type below:
_____________
Answer: Numerical expression
The mathematical phrase 5+2×18 is an example of Numerical expression.
Concepts and Skills
Find the value.
Question 3.
54
________
Answer: 5 × 5 × 5 × 5 = 625
Explanation:
The number 5 is the repeated factor.
5 is used 4 times.
Multiply 5 four times.
5 × 5 × 5 × 5 = 625
Question 4.
212
________
Answer: 21 × 21 = 441
Explanation:
The number 21 is the repeated factor.
21 is used 2 times.
Multiply 21 two times.
21 × 21 = 441
Question 5.
83
________
Answer: 8 × 8 × 8 = 512
Explanation:
The number 8 is the repeated factor.
8 is used 3 times.
8 × 8 × 8 = 512
Evaluate the expression.
Question 6.
92 × 2 − 42
________
Answer: 146
Explanation:
92 × 2 − 42
92 = 9 × 9 = 81
42 = 4 × 4 = 16
81 × 2 – 16 = 162 – 16 = 146
Thus 92 × 2 − 42 = 146
Question 7.
2 × (10 − 2) ÷ 22
________
Answer: 4
Explanation:
2 × (10 − 2) ÷ 22
2 × (10 − 2) ÷ 4
2 × 8 ÷ 4 = 16 ÷ 4 = 4
Thus 2 × (10 − 2) ÷ 22 = 4
Question 8.
30 − (33 − 8)
________
Answer: 11
Explanation:
33 = 3 × 3 × 3 = 27
30 − (33 − 8) = 30 – (27 – 8) = 30 – 19 = 11
30 − (33 − 8) = 11
So, 30 − (33 − 8) is 11.
Write an algebraic expression for the word expression.
Question 9.
the quotient of c and 8
Type below:
_____________
Answer: c ÷ 8
The quotient is nothing but the division of c by 8. So, the expression is c ÷ 8.
Question 10.
16 more than the product of 5 and p
Type below:
_____________
Answer: 16 + 5p
Explanation:
The operation for more than is addition. Here we have to add 16 to the product of 5 and p.
The product is the operation for multiplication. Multiply 5 and p and then add 16 to it.
The expression of the word is 16 + 5p.
Question 11.
9 less than the sum of x and 5
Type below:
_____________
Answer: 9 – x + 5
Explanation:
First, we have to evaluate the expression x and 5.
Sum of is nothing but adding x and 5.
Difference between 9 and x and 5
The expression is 9 – x + 5.
Evaluate the expression for the given value of the variable.
Question 12.
5 × (h + 3) for h = 7
________
Answer: 50
Explanation:
Given expression is 5 × (h + 3)
Substitute h = 7 in the above expression.
5 × (h + 3) = 5 × (7 + 3)
5 × 10 = 50
5 × (h + 3) = 50
Question 13.
2 × (c2 − 5) for c = 4
________
Answer: 22
Explanation:
Given 2 × (c2 − 5)
Substitute c = 4 in the expression
2 × (c2 − 5) = 2 × (42 − 5)
= 2 × (16 – 5) = 2 × 11 = 22
2 × (c2 − 5) = 22
Question 14.
7a − 4a for a = 8
________
Answer: 24
Explanation:
Given, 7a − 4a
Subtract the like terms
7a − 4a = 3a
Now substitute the value a = 8 in the above expression
3a = 3 × 8 = 24
7a − 4a = 24
Page No. 388
Question 15.
The greatest value of any U.S. paper money ever printed is 105 dollars. What is this amount written in standard form?
________
Answer: 100000
Explanation:
105 dollars = 10 × 10 × 10 × 10 × 10
10 is a repeated factor.
10 repeated 5 times.
10 × 10 × 10 × 10 × 10 = 100000 dollars
Question 16.
A clothing store is raising the price of all its sweaters by $3.00. Write an expression that could be used to find the new price of a sweater that originally cost d dollars.
Type below:
_____________
Answer: d + 3
Explanation:
A clothing store is raising the price of all its sweaters by $3.00.
The cost of the sweater is d dollars. The store is going to add $3.
So, the new price of a sweater is the sum of d dollars and $3.
The expression is d + 3.
Question 17.
Kendra bought a magazine for $3 and 4 paperback books for $5 each. The expression 3 + 4 × 5 represents the total cost in dollars of her purchases. What are the terms in this expression?
Type below:
_____________
Answer: 3 and 4 × 5
Explanation:
Kendra bought a magazine for $3 and 4 paperback books for $5 each. The expression 3 + 4 × 5 represents the total cost in dollars of her purchases.
The terms in the expression are 3, 4, and 5.
Question 18.
The expression 5c + 7m gives the number of people who can ride in c cars and m minivans. What are the coefficients in this expression?
Type below:
_____________
Answer: The coefficients in the expression 5c + 7m are 5 and 7.
Question 19.
The formula P = a + b + c gives the perimeter P of a triangle with side lengths a, b, and c. How much greater is the perimeter of a triangular field with sides that measure 33 yards, 56 yards, and 65 yards than the perimeter of a triangular field with sides that measure 26 yards, 49 yards, and 38 yards?
________ yards
Answer: 41 yards
Explanation:
First, we have to calculate the perimeter of the 1st triangle.
Given:
a = 33 yards
b = 56 yards
c = 65 yards
P1 = a + b + c
P1 = 33 + 56 + 65 = 154 yards
Now we have to calculate the perimeter of 2nd triangle.
Given:
a = 26 yards
b = 49 yards
c = 38 yards
P2 = a + b + c
P2 = 26 + 49 + 38 = 113 yards
Now we have to calculate which triangle has greater perimeter and how much greater.
P1 – P2 = 154 yards – 113 yards = 41 yards
Therefore, 41 yards greater is the perimeter of the 1st triangular field than the perimeter of the 2nd triangular field.
Share and Show – Page No. 391
Louisa read that the highest elevation of Mount Everest is 8,848 meters. She wants to know how much higher Mount Everest is than Mount Rainier. Use this information for 1–2.
Question 1.
Write an expression to represent the difference in the heights of the two mountains. Tell what the variable in your expression represents.
Type below:
_____________
Answer: 8848 – h, where h represents the height of the Mount Rainier
Explanation:
Given that, the height of the Mount Everest is 8848 meters
Let the height of the Mount Rainier is h
The difference in height of Mount Everest and height of the Mount Rainier is 8848 – h.
Question 2.
Louisa researches the highest elevation of Mount Rainier and finds that it is 4,392 meters. Use your expression to find the difference in the mountains’ heights.
________ meters
Answer: 4456 meters
Explanation:
The height of the Mount Rainier = 4392 meters
Replace the value of height of the Mount Rainier in the above expression.
8848 – h = 8848 meters – 4392 meters = 4456 meters
Thus the difference between the height of the two mountains is 4456 meters.
On Your Own
A muffin recipe calls for 3 times as much flour as sugar. Use this information for 3–5.
Question 3.
Write an expression that can be used to find the amount of flour needed for a given amount of sugar. Tell what the variable in your expression represents.
Type below:
_____________
Answer:
Let the amount of sugar used represents the variable is s.
The expression to find the amount of flour needed for a given amount of sugar is 3 × m i.e., 3m
Question 4.
Use your expression to find the amount of flour needed when \(\frac{3}{4}\) cup of sugar is used.
______ \(\frac{□}{□}\)
Answer: 2 \(\frac{1}{4}\)
Explanation:
Given that, A muffin recipe calls for 3 times as much flour as sugar.
The amount of flour needed when \(\frac{3}{4}\) cup of sugar used is 3 × \(\frac{3}{4}\) = \(\frac{9}{4}\)
Convert the improper fraction into the mixed fraction.
\(\frac{9}{4}\) = 2 \(\frac{1}{4}\)
Therefore 2 \(\frac{1}{4}\) amount of flour needed when \(\frac{3}{4}\) cup of sugar is used.
Question 5.
Reason Quantitatively Is the value of the variable in your expression restricted to a particular set of numbers? Explain.
Type below:
_____________
Answer: The values that make the denominator equal to zero for a rational expression are known as restricted values. The solutions are the restricted values since they result in a denominator of zero when replaced for the variable(s).
Practice: Copy and Solve Write an algebraic expression for each word expression. Then evaluate the expression for these values of the variable: \(\frac{1}{2}\), 4, and 6.5.
Question 6.
the quotient of p and 4
Type below:
_____________
Answer: p ÷ 4
Explanation:
The expression is p ÷ 4
p = \(\frac{1}{2}\)
\(\frac{1}{2}\) ÷ 4
\(\frac{1}{2}\)/4 = \(\frac{1}{8}\)
p ÷ 4 when p = \(\frac{1}{2}\) is \(\frac{1}{8}\)
p = 4
4 ÷ 4 = 1
p = 6.5
6.5 ÷ 4 = 1.625
Question 7.
4 less than the sum of x and 5
Type below:
_____________
Answer: 4 – (x + 5)
Explanation:
The expression is 4 – (x + 5)
x = 1/2
4 – (x + 5) = 4 – 1/2 + 5
3 1/2 + 5 = 8 1/2
x = 4
4 – x + 5
4 – 4 + 5 = 5
x = 6.5
4 – 6.5 + 5 = 2.5
Problem Solving + Applications – Page No. 392
Use the graph for 8–10.
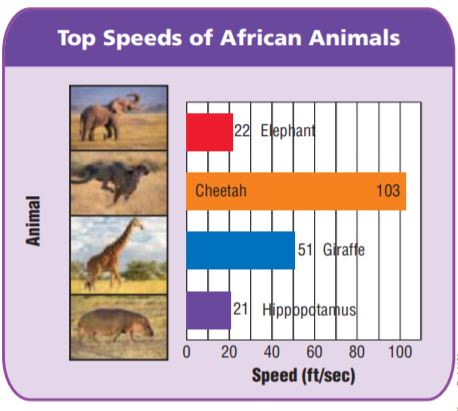
Question 8.
Write expressions for the distance in feet that each animal could run at top speed in a given amount of time. Tell what the variable in your expressions represents.
Type below:
_____________
Answer:
The expression for distance in feet for Elephant = 22t
The expression for distance in feet for Cheetah = 103t
The expression for distance in feet for Giraffe = 51t
The expression for distance in feet for hippopotamus = 21t
Where t represents the time.
Question 9.
How much farther could a cheetah run in 20 seconds at top speed than a hippopotamus could?
______ feet
Answer: 1640 feet
Explanation:
The expression for distance in feet for Cheetah = 103t
where t = 20 sec
103t = 103 × 20 sec = 2060 feet
The expression for distance in feet for hippopotamus = 21t
where t = 20 sec
21t = 21 × 20 = 420 feet
Now we have to find How much farther could a cheetah run in 20 seconds at top speed than a hippopotamus could
2060 feet – 420 feet = 1640 feet
Question 10.
A giraffe runs at top speed toward a tree that is 400 feet away. Write an expression that represents the giraffe’s distance in feet from the tree after s seconds.
Type below:
_____________
Answer:
The expression representing the giraffe’s distance from tree after s seconds, if the rate is 51 ft per second.
7 43/60 seconds in all
Question 11.
A carnival charges $7 for admission and $2 for each ride. An expression for the total cost of going to the carnival and riding n rides is 7 + 2n.
Complete the table by finding the total cost of going to the carnival and riding n rides.
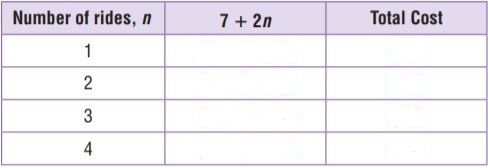
Type below:
_____________
Answer:

Use Algebraic Expressions – Page No. 393
Jeff sold the pumpkins he grew for $7 each at the farmer’s market.
Question 1.
Write an expression to represent the amount of money in dollars Jeff made selling the pumpkins. Tell what the variable in your expression represents
Type below:
_____________
Answer: 7p, where p is the number of pumpkins
Question 2.
If Jeff sold 30 pumpkins, how much money did he make?
$ ________
Answer: 210
Explanation:
The expression is 7p
p = 30 pumpkins
7 × 30 = 210
Thus Jeff sold 30 pumpkins for $210.
An architect is designing a building. Each floor will be 12 feet tall.
Question 3.
Write an expression for the number of floors the building can have for a given building height. Tell what the variable in your expression represents.
Type below:
_____________
Answer: The expression for the number of floors is h/12, where h is the height of the building.
Question 4.
If the architect is designing a building that is 132 feet tall, how many floors can be built?
________ floors
Answer: 11 floors
Explanation:
Given the height of the building is 132 feet
Substitute h in the above expression
h/12 = 132/12 = 11 floors
Thus 11 floors can be built.
Write an algebraic expression for each word expression. Then evaluate the expression for these values of the variable: 1, 6, 13.5.
Question 5.
the quotient of 300 and the sum of b and 24
Type below:
_____________
Answer: 300 ÷ (b + 24)
Explanation:
For b = 1
300 ÷ (b + 24) = 300 ÷ (1 + 24)
300 ÷ 25 = 12
Thus 300 ÷ (b + 24) when b = 1 is 12.
For b = 6
300 ÷ (b + 24) = 300 ÷ (6 + 24)
300 ÷ 30 = 10
Thus 300 ÷ (b + 24) when b = 6 is 10.
For b = 13.5
300 ÷ (b + 24) = 300 ÷ (13.5 + 24)
300 ÷ 37.5 = 8
300 ÷ (b + 24) when b = 13.5 is 8.
Question 6.
13 more than the product of m and 5
Type below:
_____________
Answer: 13 + 5m
Explanation:
For m = 1
13 + 5m = 13 + 5(1) = 13 + 6 = 19
For m = 6
13 + 5m = 13 + 5(6) = 13 + 30 = 43
For m = 13.5
13 + 5m = 13 + 5(13.5) = 13 + 67.5 = 80.5
Problem Solving
Question 7.
In the town of Pleasant Hill, there is an average of 16 sunny days each month. Write an expression to represent the approximate number of sunny days for any number of months. Tell what the variable represents.
Type below:
_____________
Answer: 16m, m for months
Explanation:
In the town of Pleasant Hill, there is an average of 16 sunny days each month. Write an expression to represent the approximate number of sunny days for any number of months.
we have to multiply the number of months with 16
The expression will be 16 times of m = 16m
Question 8.
How many sunny days can a resident of Pleasant Hill expect to have in 9 months?
________ days
Answer: 144 days
Explanation:
The expression to represent the approximate number of sunny days for any number of months is 16m
m = 9
Substitute the value of m in the expression.
16m = 16 × 9 = 144 days
Question 9.
Describe a situation in which a variable could be used to represent any whole number greater than 0.
Type below:
_____________
Answer: To represent the number of people any answer can be accepted.
Lesson Check – Page No. 394
Question 1.
Oliver drives 45 miles per hour. Write an expression that represents the distance in miles he will travel for h hours driven.
Type below:
_____________
Answer: 45h
Explanation:
It is given that Oliver drives 45 miles per hour. Let the number of hours he drove be h. The distance is the product of speed and time. The distance travel by Oliver is defined by the expression is 45h.
Question 2.
Socks cost $5 per pair. The expression 5p represents the cost in dollars of p pairs of socks. Why must p be a whole number?
Type below:
_____________
Answer: p must be a whole number because in almost 100% of all stores it is not allowed to buy a single sock, you must always buy a pair of socks.
Spiral Review
Question 3.
Sterling silver consists of 92.5% silver and 7.5% copper. What decimal represents the portion of the silver in sterling silver?
________
Answer: 0.925
Explanation:
If Sterling silver is 92.5% silver, that means it has 92.5/100 * 100% silver
The fraction 92.5/100 can be simplified by just moving the decimal 2 places to the left:
92.5/100 = .925
Question 4.
How many pints are equivalent to 3 gallons?
________ pints
Answer: 24
Explanation:
Convert from gallons to pints.
1 gallon = 8 pints
3 gallons = 3 × 8 pints = 24 pints
24 pints are equivalent to 3 gallons.
Question 5.
Which operation should be done first to evaluate 10 + (66 – 62)?
Type below:
_____________
Answer: Square 6
Question 6.
Evaluate the algebraic expression h(m + n) ÷ 2 for h = 4, m = 5, and n = 6.
________
Explanation:
Given the expression h(m + n) ÷ 2
h = 4
m = 5
n = 6
h(m + n) ÷ 2 = 4 (5 + 6) ÷ 2
4 (11) ÷ 2 = 44 ÷ 2 = 22
h(m + n) ÷ 2 = 22
Share and Show – Page No. 397
Question 1.
Museum admission costs $7, and tickets to the mammoth exhibit cost $5. The expression 7p + 5p represents the cost in dollars for p people to visit the museum and attend the exhibit. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 12p
Explanation:
7p+5p
When you combine like terms, you just add all the terms that have the same variable
so you get 7p + 5p = 12p
Question 2.
What if the cost of tickets to the exhibit was reduced to $3? Write an expression for the new cost in dollars for p people to visit the museum and attend the exhibit. Then, simplify the expression by combining like terms.
Type below:
_____________
Answer: 10p
Explanation:
Museum admission costs $7, and tickets to the mammoth exhibit cost $5.
The expression 7p + 5p represents the cost in dollars for p people to visit the museum and attend the exhibit.
The cost of tickets to the mammoth exhibit is $5.
If it is reduced to $3 then the cost will be $5 – $2 = $3
12p – 2p = 10p
Question 3.
A store receives tomatoes in boxes of 40 tomatoes each. About 4 tomatoes per box cannot be sold due to damage. The expression 40b − 4b gives the number of tomatoes that the store can sell from a shipment of b boxes. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 36b
Explanation:
Given, A store receives tomatoes in boxes of 40 tomatoes each.
About 4 tomatoes per box cannot be sold due to damage.
The expression 40b − 4b gives the number of tomatoes that the store can sell from a shipment of b boxes.
Subtract 40b and 4b
40b – 4b = 36b
Question 4.
Each cheerleading uniform includes a shirt and a skirt. The shirts cost $12 each, and skirts cost $18 each. The expression 12u + 18u represents the cost in dollars of buying u uniforms. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 30u
Explanation:
The expression 12u + 18u represents the cost in dollars of buying u uniforms.
12u and 18u are the like terms. So add the two terms
12u + 18u = 30u
Question 5.
A shop sells vases holding 9 red roses and 6 white roses. The expression 9v + 6v represents the total number of roses needed for v vases. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 15v
Explanation:
A shop sells vases holding 9 red roses and 6 white roses.
The expression 9v + 6v represents the total number of roses needed for v vases.
The like terms are 9v and 6v
9v + 6v = 15v
On Your Own – Page No. 398
Question 6.
Marco received a gift card. He used it to buy 2 bike lights for $10.50 each. Then he bought a handlebar bag for $18.25. After these purchases, he had $0.75 left on the card. How much money was on the gift card when Marco received it?
$ _______
Answer:
Marco received a gift card. He used it to buy 2 bike lights for $10.50 each.
Then he bought a handlebar bag for $18.25.
After these purchases, he had $0.75 left on the card.
Add total amount = 2 × $10.50 + $18.25 + $0.75
$21 + $19 = $40
$40 was on the gift card when Marco received it.
Question 7.
Lydia collects shells. She has 24 sea snail shells, 16 conch shells, and 32 scallop shells. She wants to display the shells in equal rows, with only one type of shell in each row. What is the greatest number of shells Lydia can put in each row?
_______ shells
Answer: 8 shells
Explanation:
Lydia collects shells. She has 24 sea snail shells, 16 conch shells, and 32 scallop shells.
She wants to display the shells in equal rows, with only one type of shell in each row.
The possible shells in equal rows are 8 because 16, 24, and 32 are the multiples of 8.
Thus the greatest number of shells Lydia can put in each row is 8.
Question 8.
The three sides of a triangle measure 3x + 6 inches, 5x inches, and 6x inches. Write an expression for the perimeter of the triangle in inches. Then simplify the expression by combining like terms.
Type below:
_____________
Answer:
Perimeter of the triangle = a + b + c
Let a = 3x + 6 inches
b = 5x inches
c = 6x inches
P = a + b + c
P = 3x + 6 + 5x + 6x
Combine the like terms 3x, 5x, 6x
P = 14x + 6
Thus the perimeter of the triangle is 14x + 6.
Question 9.
Verify the Reasoning of Others Karina states that you can simplify the expression 20x + 4 by combining like terms to get 24x. Does Karina’s statement make sense? Explain.
Type below:
_____________
Answer: Karina’s statement doesn’t make sense. Because the 20x + 4 are not the like terms.
We can add only the like terms. 20x + 4 ≠ 24x
Question 10.
Vincent is ordering accessories for his surfboard. A set of fins costs $24 each and a leash costs $15. The shipping cost is $4 per order. The expression 24b + 15b + 4 can be used to find the cost in dollars of buying b fins and b leashes plus the cost of shipping.
For numbers 10a–10c, select True or False for each statement.
10a. The terms are 24b, 15b and 4.
10b. The like terms are 24b and 15b.
10c. The simplified expression is 43b.
10a. _____________
10b. _____________
10c. _____________
Answer:
10a. True
10b. True
10c. False
Explanation:
a. The terms of the expression 24b + 15b + 4 area 24b, 15b, 4.
b. The terms are said to be like if they have the common variable. So, the common terms are 24b, 15b.
c. Combine the like terms 24b and 15b
24b + 15b = 39b
Thus the statement is false.
Problem Solving Combine Like Terms – Page No. 399
Read each problem and solve.
Question 1.
A box of pens costs $3 and a box of markers costs $5. The expression 3p + 5p represents the cost in dollars to make p packages that include 1 box of pens and 1 box of markers. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 3p + 5p = 8p
Explanation:
A box of pens costs $3 and a box of markers costs $5.
The expression 3p + 5p represents the cost in dollars to make p packages that include 1 box of pens and 1 box of markers.
Adding the like terms 3p + 5p is 8p.
Question 2.
Riley’s parents got a cell phone plan that has a $40 monthly fee for the first phone. For each extra phone, there is a $15 phone service charge and a $10 text service charge. The expression 40 + 15e + 10e represents the total phone bill in dollars, where e is the number of extra phones. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 25e + 40
Explanation:
Given that,
Riley’s parents got a cell phone plan that has a $40 monthly fee for the first phone.
For each extra phone, there is a $15 phone service charge and a $10 text service charge.
The expression 40 + 15e + 10e represents the total phone bill in dollars,
We have to combine the like terms here
The like terms in the expression are 15e and 10e.
That means 40 + 15e + 10e = 25e + 40
Question 3.
A radio show lasts for h hours. For every 60 minutes of air time during the show, there are 8 minutes of commercials. The expression 60h – 8h represents the air time in minutes available for talk and music. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 52h
Explanation:
A radio show lasts for h hours. For every 60 minutes of air time during the show, there are 8 minutes of commercials.
The expression 60h – 8h represents the air time in minutes available for talk and music.
Now we have to Subtract the like terms 60h – 8h = 52h
Question 4.
A sub shop sells a meal that includes an Italian sub for $6 and chips for $2. If a customer purchases more than 3 meals, he or she receives a $5 discount. The expression 6m + 2m – 5 shows the cost in dollars of the customer’s order for m meals, where m is greater than 3. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 8m – 5
Explanation:
The expression is 6m + 2m – 5
Now combine the like terms 6m + 2m – 5 = 8m – 5
Question 5.
Explain how combining like terms is similar to adding and subtracting whole numbers. How are they different?
Type below:
_____________
Answer: It’s the same because you are adding or subtracting numbers but it’s different because they can only be added or subtracted if the variable attached is the same. There are no variables when adding/subtracting regular whole numbers.
Lesson Check – Page No. 400
Question 1.
For each gym class, a school has 10 soccer balls and 6 volleyballs. All of the classes share 15 basketballs. The expression 10c + 6c + 15 represents the total number of balls the school has for c classes. What is a simpler form of the expression?
Type below:
_____________
Answer: 16c + 15
Explanation:
For each gym class, a school has 10 soccer balls and 6 volleyballs.
All of the classes share 15 basketballs.
c represents classes.
The expression is 10c + 6c + 15
Combine the like terms 10c and 6c
Now add common terms 10c + 6c + 15 = 16c + 15
Question 2.
A public library wants to place 4 magazines and 9 books on each display shelf. The expression 4s + 9s represents the total number of items that will be displayed on s shelves. Simplify this expression.
Type below:
_____________
Answer: 13s
Explanation:
A public library wants to place 4 magazines and 9 books on each display shelf.
The expression is 4s + 9s
Combine the like terms 4s + 9s = 13s
Spiral Review
Question 3.
A bag has 8 bagels. Three of the bagels are cranberry. What percent of the bagels are cranberry?
________ %
Answer: 37.5%
Explanation:
[/latex]\frac{3}{8}[/latex] = 0.375
0.375 × 100 = 37.5 %
37.5% of the bagels are cranberry.
Question 4.
How many kilograms are equivalent to 3,200 grams?
________ kilograms
Answer: 3.2 kg
Explanation:
Convert from grams into kilograms
1000 grams = 1kg
3200 grams = 3200 × 1/1000 kg = 3.2 kg
3.2 kilograms are equivalent to 3,200 grams.
Question 5.
Toni earns $200 per week plus $5 for every magazine subscription that she sells. Write an expression that represents how much she will earn in dollars in a week in which she sells s subscriptions.
Type below:
_____________
Answer: 200 + 5s
Explanation:
Toni earns $200 per week plus $5 for every magazine subscription that she sells.
s represents subscriptions.
200 + 5 × s
Thus the expression that represents how much she will earn in dollars in a week is 200 + 5s
Question 6.
At a snack stand, drinks cost $1.50. Write an expression that could be used to find the total cost in dollars of d drinks.
Type below:
_____________
Answer: 1.5d
Explanation:
At a snack stand, drinks cost $1.50.
To find the total cost in dollars of d drinks we have to multiply 1.50 with d.
1.50 × d
Thus the expression that could be used to find the total cost in dollars of d drinks is 1.5d
Share and Show – Page No. 403
Use properties of operations to write an equivalent expression by combining like terms.
Question 1.
\(3 \frac{7}{10} r-1 \frac{1}{5} r\)
Type below:
_____________
Answer: 2 \(frac{5}{10}\)r
Explanation:
3 \(frac{7}{10}\)r – 1 \(frac{1}{5}\)r
3 + \(frac{7}{10}\)r – 1 – \(frac{1}{5}\)r
3 – 1 = 2
\(frac{7}{10}\)r – \(frac{1}{5}\)r
\(frac{7}{10}\)r – \(frac{2}{10}\)r = \(frac{5}{10}\)r
\(3 \frac{7}{10} r-1 \frac{1}{5} r\) = 2 \(frac{5}{10}\)r
Question 2.
20a + 18 + 16a
Type below:
_____________
Answer: 36a + 18
Explanation:
Combine the like terms first
16a and 20a are like terms in the given expression.
Add 16a and 20a
16a + 20a +18 = 36a + 18
Question 3.
7s + 8t + 10s + 12t
Type below:
_____________
Answer: 17s + 20t
Explanation:
There are 4 terms in the expression they are 7s, 10s, 8t, 12t.
Now combine the like terms
7s + 10s + 8t + 12t = 17s + 20t
Use the Distributive Property to write an equivalent expression.
Question 4.
8(h + 1.5)
Type below:
_____________
Answer: 8h + 12
Explanation:
Here we have to use the distributive property for the above expression.
8(h + 1.5) = 8 × h + 1.5 × 8
= 8h + 12
Thus 8(h + 1.5) is 8h + 12.
Question 5.
4m + 4p
Type below:
_____________
Answer: 4(m + p)
Explanation:
Here we have to take 4 as a common factor from the expression.
4m + 4p = 4 × m + 4 × p
That implies 4 × (m + p)
Question 6.
3a + 9b
Type below:
_____________
Answer: 3(a + 3b)
Explanation:
Let us take 4 as a common factor from the expression.
3a + 9b = 3 × a + 9 × b
3(a + 3b)
3a + 9b = 3(a + 3b)
On Your Own
Practice: Copy and Solve Use the Distributive Property to write an equivalent expression.
Question 7.
3.5(w + 7)
Type below:
_____________
Answer: 3.5w + 24.5
Explanation:
Use the distributive property.
Multiply within the parentheses.
3.5(w + 7) = 3.5 × w + 3.5 × 7
3.5w + 24.5
Thus 3.5(w + 7) = 3.5w + 24.5
Question 8.
\(\frac{1}{2}\)(f + 10)
Type below:
_____________
Answer: \(\frac{1}{2}\)f + 5
Explanation:
\(\frac{1}{2}\)(f + 10)
Use the distributive property.
Multiply within the parentheses.
\(\frac{1}{2}\) × f + \(\frac{1}{2}\) × 10
= \(\frac{1}{2}\)f + 5
Thus \(\frac{1}{2}\)(f + 10) = \(\frac{1}{2}\)f + 5
Question 9.
4(3z + 2)
Type below:
_____________
Answer: 12z + 8
Explanation:
Use the distributive property.
Multiply within the parentheses.
4(3z + 2) = 4 × 3z + 4 × 2
= 12z + 8
So, 4(3z + 2) = 12z + 8
Question 10.
20b + 16c
Type below:
_____________
Answer: 4(5b + 4c)
Explanation:
20b + 16c
Use the distributive property.
Multiply within the parentheses.
Take 4 as a common factor.
20b + 16c = 4 × 5b + 4 × 4c = 4 (5b + 4c)
Thus the expression 20b + 16c = 4 (5b + 4c)
Question 11.
30d + 18
Type below:
_____________
Answer: 6(5d + 3)
Explanation:
30 and 18 are the factors of 6.
So, take 6 as a common factor.
30d + 18 = 6 × 5d + 6 × 3
6 (5d + 3)
30d + 18 = 6 (5d + 3)
Question 12.
24g − 8h
Type below:
_____________
Answer: 8(3g – h)
Explanation:
Given the expression 24g − 8h
24 and 8 are the factors of 8.
So, let us take 8 as a common factor.
24g − 8h = 8 × 3g – 8 × 1h
= 8(3g – h)
Question 13.
Write an Expression The lengths of the sides of a triangle are 3t, 2t + 1, and t + 4. Write an expression for the perimeter (sum of the lengths). Then, write an equivalent expression with 2 terms.
Type below:
_____________
Answer: 6t + 5
Explanation:
Given that, The lengths of the sides of a triangle are 3t, 2t + 1, and t + 4.
We know that the perimeter of the triangle is P = a + b + c
P = 3t + 2t + 1 + t + 4
Combine the like terms.
P = 6t + 5
Question 14.
Use properties of operations to write an expression equivalent to the sum of the expressions 3(g + 5) and 2(3g − 6).
Type below:
_____________
Answer: 3(3g + 1)
Explanation:
Given two expressions 3(g + 5) and 2(3g − 6).
Use the distributive property to simplify the expressions.
3(g + 5) = 3 × g + 3 × 5 = 3g + 15
2(3g − 6) = 2 × 3g – 2 × 6 = 6g – 12
Add both the expressions and combine the like terms
3g + 15 + 6g – 12 = 9g + 3 = 3(3g + 1)
Problem Solving + Applications – Page No. 404
Question 15.
Sense or Nonsense Peter and Jade are using what they know about properties to write an expression equivalent to 2 × (n + 6) + 3. Whose answer makes sense? Whose answer is nonsense? Explain your reasoning.
Peter’s Work:
Expression: 2 × (n + 6) + 3
Associative Property of Addition: 2 × n + (6 + 3)
Add within parentheses: 2 × n + 9
Multiply: 2n + 9
Jade’s Work:
Expression: 2 × (n + 6) + 3
Distributive Property: (2 × n) + (2 × 6) + 3
Multiply within parentheses: 2n + 12 + 3
Associative Property of Addition: 2n + (12 + 3)
Add within parentheses: 2n + 15
For the answer that is nonsense, correct the statement.
Type below:
_____________
Answer: Jade’s Work makes sense. Peter’s Work makes non-sense because
He must have multiplied n + 6 with 2 but he added 6 with 3.
2 × (n + 6) + 3
2 × n + 2 × 6 + 3 = 2n + 12 + 3
= 2n + 15
Question 16.
Write the algebraic expression in the box that shows an equivalent expression.

Type below:
_____________
Answer:
6(z + 5) = 6 × z + 6 × 5 = 6z + 30
6z + 5z = z(6 + 5) = 11z
2 + 6z + 3 = 6z + 5
Generate Equivalent Expressions – Page No. 405
Use properties of operations to write an equivalent expression by combining like terms.
Question 1.
7h − 3h
Type below:
_____________
Answer: 4h
Explanation:
Combine the like terms
7h and 3h are the common terms
Now subtract 3h from 7h
7h – 3h = 4h
Question 2.
5x + 7 + 2x
Type below:
_____________
Answer: 7x + 7
Explanation:
The given expression is 5x + 7 + 2x
The common terms are 5x and 2x
Combine the like terms 5x + 7 + 2x = 7x + 7
Question 3.
16 + 13p − 9p
Type below:
_____________
Answer: 16 + 4p
Explanation:
Combine the like terms for the above expressions.
The like terms are 13p and 9p
16 + 13p − 9p = 16 + 4p
Question 4.
y2 + 13y − 8y
Type below:
_____________
Answer: y2 + 5y
Explanation:
The given expression is y2 + 13y − 8y
The like terms are 13y and 8y
y2 + 13y − 8y = y2 + 5y
Question 5.
5(2h + 3) + 3h
Type below:
_____________
Answer: 13h + 15
Explanation:
5(2h + 3) + 3h = 10h + 15 + 3h
The like terms are 10h and 3h
10h + 15 + 3h = 13h + 15
Question 6.
12 + 18n + 7 − 14n
Type below:
_____________
Answer: 19 + 4n
Explanation:
The expression is 12 + 18n + 7 − 14n
The like terms are 18n and 14n
12 + 18n + 7 − 14n = 19 + 4n
Use the Distributive Property to write an equivalent expression.
Question 7.
2(9 + 5k)
Type below:
_____________
Answer: 18 + 10k
Explanation:
Use the Distributive property
Multiply within the parentheses.
2(9 + 5k) = (2 × 9) + (2 × 5k)
(2 × 9) + (2 × 5k) = 18 + 10k
Question 8.
4d + 8
Type below:
_____________
Answer: 4(d + 2)
Explanation:
Use the Distributive property
Multiply within the parentheses.
4d + 8 = 4 × d + 4 × 2
The common term is 4.
Take 4 as a common factor.
4d + 8 = 4 (d + 2)
Question 9.
21p + 35q
Type below:
_____________
Answer: 7(3p + 5q)
Explanation:
Use the Distributive property
Multiply within the parentheses.
7 × 3p + 7 × 5q
The common term is 7.
7(3p + 5q)
21p + 35q = 7(3p + 5q)
Problem Solving
Question 10.
The expression 15n + 12n + 100 represents the total cost in dollars for skis, boots, and a lesson for n skiers. Simplify the expression 15n + 12n + 100. Then find the total cost for 8 skiers.
Type below:
_____________
Answer: 27n + 100, $316
Explanation:
The terms that have n can be operated:
15n +12n + 100 = 27n +100. Then, we have that
total cost = 27n +100 for n skiers. So, for 8 skiers we have
total cost = 27(8) +100 = 216 + 100 = 316.
Then, the total cost of 8 skiers is $316.
Question 11.
Casey has n nickels. Megan has 4 times as many nickels as Casey has. Write an expression for the total number of nickels Casey and Megan have. Then simplify the expression.
Type below:
_____________
Answer: n + 4n; 5n
Explanation:
Casey has n nickels. Megan has 4 times as many nickels as Casey has.
Sum of n and 4n
Add the common terms n and 4n.
n + 4n = 5n
Question 12.
Explain how you would use properties to write an expression equivalent to 7y + 4b – 3y.
Type below:
_____________
Answer:
1st you combine like terms so subtract 7y and 3y and you get 4y.
So this is the final answer: 4y+4b.
Lesson Check – Page No. 406
Question 1.
A ticket to a museum costs $8. A ticket to the dinosaur exhibit costs $5. The expression 8n + 5n represents the cost in dollars for n people to visit the museum and the exhibit. What is a simpler form of the expression 8n + 5n?
Type below:
_____________
Answer: 13n
Explanation:
A ticket to a museum costs $8. A ticket to the dinosaur exhibit costs $5.
The expression is the sum of 8n and 5n.
Thus the simpler form of the expression is 8n + 5n = 13n
Question 2.
What is an expression that is equivalent to 3(2p – 3)?
Type below:
_____________
Answer: 6p – 9
Explanation:
Use the distributive property to find the equivalent expression.
3(2p – 3) = 3 × 2p – 3 × 3
= 6p – 9
Thus the expression that is equivalent to 3(2p – 3) is 6p – 9.
Question 3.
A Mexican restaurant received 60 take-out orders. The manager found that 60% of the orders were for tacos and 25% of the orders were for burritos. How many orders were for other items?
______ orders
Answer: 9 orders
Explanation:
Given,
A Mexican restaurant received 60 take-out orders.
The manager found that 60% of the orders were for tacos and 25% of the orders were for burritos.
The answer is 9 because 25% of 60 is 15 plus 60% of 60 is 36 so 36+15=51 and 60-51=9
Thus 9 orders were for other items.
Question 4.
The area of a rectangular field is 1,710 square feet. The length of the field is 45 feet. What is the width of the field?
______ feet
Answer: 38 feet
Explanation:
The area of a rectangular field is 1,710 square feet.
The length of the field is 45 feet.
The width of the field is x feet
A = l × w
1710 square feet = 45 feet × x
x = 1710/45 = 38 feet
Thus the width of the rectangular field is 38 feet.
Question 5.
How many terms are in 2 + 4x + 7y?
______ terms
Answer: 3
Explanation:
Given expression 2 + 4x + 7y
There are 3 terms in the expression 2, 4x, 7y.
Question 6.
Boxes of cereal usually cost $4, but they are on sale for $1 off. A gallon of milk costs $3. The expression 4b – 1b + 3 can be used to find the cost in dollars of buying b boxes of cereal and a gallon of milk. Write the expression in a simpler form.
Type below:
_____________
Answer: 3b + 3
Explanation:
Boxes of cereal usually cost $4, but they are on sale for $1 off. A gallon of milk costs $3.
The expression is 4b – 1b + 3
Combine the like terms for the above expression.
4b – 1b + 3 = 3b + 3 = 3(b + 1)
Share and Show – Page No. 409
Use properties of operations to determine whether the expressions are equivalent.
Question 1.
7k + 4 + 2k and 4 + 9k
The expressions are _____________
Answer: equivalent
Explanation:
7k + 4 + 2k
Combine the like terms 7k and 2k
Add the like terms 7k + 2k + 4 = 9k + 4
9k + 4 and 4 + 9k are equivalent.
Question 2.
9a × 3 and 12a
The expressions are _____________
Answer: not equivalent
Explanation:
Multiply 9a with 3.
9a × 3 = 27a
27a and 12a are not equivalent.
Thus the expressions are not equivalent.
Question 3.
8p + 0 and 8p × 0
The expressions are ______
Answer: not equivalent
Explanation:
8p + 0 = 8p
8p × 0 = 0
8p and 0 are not equivalent.
The expressions 8p + 0 and 8p × 0 are not equivalent.
Question 4.
5(a + b) and (5a + 2b) + 3b
The expressions are _____________
Answer: equivalent
Explanation:
5(a + b) = 5a + 5b
(5a + 2b) + 3b
The like terms are 5a and 2b, 3b
Add the combine terms 5a + 2b + 3b = 5a + 5b
Thus the expressions 5(a + b) and (5a + 2b) + 3b are equivalent.
On Your Own
Use properties of operations to determine whether the expressions are equivalent.
Question 5.
3(v + 2) + 7v and 16v
The expressions are _____________
Answer: not equivalent
Explanation:
3(v + 2) + 7v
Combine the like terms 3v and 7v
3(v + 2) + 7v = 3v + 6 + 7v = 10v + 6
The expressions 10v + 6 and 16v are not equivalent.
Question 6.
14h + (17 + 11h) and 25h + 17
The expressions are _____________
Answer: equivalent
Explanation:
14h + (17 + 11h)
Combine the like terms 14h and 11h.
14h + 17 + 11h = 25h + 17
The expressions 14h + (17 + 11h) and 25h + 17 are equivalent.
Question 7.
4b × 7 and 28b
The expressions are _____________
Answer: equivalent
Explanation:
Multiply 4b with 7.
4b × 7 = 28b
The expressions 4b × 7 and 28b are equivalent.
Question 8.
Each case of dog food contains c cans. Each case of cat food contains 12 cans. Four students wrote the expressions below for the number of cans in 6 cases of dog food and 1 case of cat food. Which of the expressions are correct?
6c + 12 6c × 12 6(c + 2) (2c + 4) × 3
Type below:
_____________
Answer: The correct expressions are 6c + 12, 6(c + 2), (2c + 4) × 3
6(c + 2) is the distributive form of the expression.
Problem Solving + Applications – Page No. 410
Use the table for 9–11.
Question 9.
Marcus bought 4 packets of baseball cards and 4 packets of animal cards. Write an algebraic expression for the total number of cards Marcus bought.
Type below:
_____________
Answer: 4a + 4b
Explanation:
Marcus bought 4 packets of baseball cards and 4 packets of animal cards.
b represents the number per packet
Multiply 4 with b
4 × b = 4b
a represents the number per packet of animal cards.
Multiply 4 with a.
4 × a = 4a
Therefore the algebraic expression for the total number of cards Marcus bought is the sum of 4a and 4b.
The expression is 4a + 4b
Question 10.
Make Arguments Is the expression for the number of cards Marcus bought equivalent to 4(a + b)? Justify your answer.
Type below:
_____________
Answer: Yes
Use the distributive property to simplify the expression 4a + 4b.
Take 4 as the common factor for the expression 4a + 4b.
4a + 4b = 4(a + b)
Question 11.
Angelica buys 3 packets of movie cards and 6 packets of cartoon cards and adds these to the 3 packets of movie cards she already has. Write three equivalent algebraic expressions for the number of cards Angelica has now
Type below:
_____________
Answer: 3m + 6c + 3m
Explanation:
Angelica buys 3 packets of movie cards and 6 packets of cartoon cards and adds these to the 3 packets of movie cards she already has.
The expression for 3 packets of movie cards is 3m
The expression for 6 packets of cartoon cards is 6c.
Now we have to add 3m to the expression.
3m + 6c + 3m
Thus the three equivalent algebraic expressions for the number of cards Angelica has now is 3m + 6c + 3m
Question 12.
Select the expressions that are equivalent to 3(x + 2). Mark all that apply.
Options:
a. 3x + 6
b. 3x + 2
c. 5x
d. x + 5
Answer: 3x + 6
Explanation:
Use distributive property to solve the expression 3(x + 2).
3(x + 2) = 3 × x + 3 × 2 = 3x + 6
Thus the correct answer is option A.
Identify Equivalent Expressions – Page No. 411
Use properties of operations to determine whether the expressions are equivalent.
Question 1.
2s + 13 + 15s and 17s + 13
The expressions are _____________
Answer: equivalent
Explanation:
2s + 13 + 15s
Combine the like terms
2s + 13 + 15s = 17s + 13
17s + 13 = 17s + 13
Thus the expressions 2s + 13 + 15s and 17s + 13 are equivalent.
Question 2.
5 × 7h and 35h
The expressions are _____________
Answer: equivalent
Explanation:
5 × 7h = 35h
35h = 35h
The expressions 5 × 7h and 35h are equivalent.
Question 3.
10 + 8v − 3v and 18 − 3v
The expressions are _____________
Answer: not equivalent
Explanation:
Combine the like terms 8v and 3v
10 + 8v − 3v = 10 + 5v
10 + 5v ≠ 18 − 3v
Thus the expressions 10 + 8v − 3v and 18 − 3v are not equivalent.
Question 4.
(9w × 0)−12 and 9w – 12
The expressions are _____________
Answer: not equivalent
Explanation:
(9w × 0)−12 = 0 – 12 = – 12
– 12 ≠ 9w – 12
So, the expressions (9w × 0)−12 and 9w – 12 are not equivalent.
Question 5.
11(p + q) and 11p + (7q + 4q)
The expressions are _____________
Answer: equivalent
Explanation:
11(p + q) = 11p + 11q
Combine the terms 7q and 4q
11p + (7q + 4q) = 11p + 11q = 11(p + q)
So, the expressions 11(p + q) and 11p + (7q + 4q) are equivalent.
Question 6.
6(4b + 3d) and 24b + 3d
The expressions are _____________
Answer: not equivalent
Explanation:
6(4b + 3d) = 24b + 18d
24b + 18d ≠ 24b + 3d
So, the expressions 6(4b + 3d) and 24b + 3d are not equivalent.
Question 7.
14m + 9 − 6m and 8m + 9
The expressions are _____________
Answer: equivalent
Explanation:
Combine the like terms 14m and 6m
14m + 9 − 6m = 8m + 9
8m + 9 = 8m + 9
Thus the expressions are equivalent.
Question 8.
(y × 1) + 2 and y + 2
The expressions are _____________
Answer: equivalent
Explanation:
(y × 1) + 2 = y + 2
y + 2 = y + 2
Thus the expressions (y × 1) + 2 and y + 2 are equivalent.
Question 9.
4 + 5(6t + 1) and 9 + 30t
The expressions are _____________
Answer: equivalent
Explanation:
4 + 5(6t + 1) = 4 + 30t + 5 = 9 + 30t
9 + 30t = 9 + 30t
Thus the expressions 4 + 5(6t + 1) and 9 + 30t are equivalent.
Question 10.
9x + 0 + 10x and 19x + 1
The expressions are _____________
Answer: not equivalent
Explanation:
9x + 0 + 10x
Combine the like terms 9x and 10x.
9x + 10x = 19x
19x ≠ 19x + 1
Thus the expressions 9x + 0 + 10x and 19x + 1 are not equivalent.
Question 11.
12c − 3c and 3(4c − 1)
The expressions are _____________
Answer: not equivalent
Explanation:
12c − 3c
Take 3 as a common factor.
3c(4 – 1) or 3 (4c – 1c)
3 (4c – 1c) ≠ 3(4c − 1)
Thus the expressions 12c − 3c and 3(4c − 1) are not equivalent.
Question 12.
6a × 4 and 24a
The expressions are _____________
Answer: equivalent
Explanation:
6a × 4 = 24a
24a = 24a
The expressions 6a × 4 and 24a are equivalent.
Problem Solving
Question 13.
Rachel needs to write 3 book reports with b pages and 3 science reports with s pages during the school year. Write an algebraic expression for the total number of pages Rachel will need to write.
Type below:
_____________
Answer: 3b + 3s
Explanation:
Rachel needs to write 3 book reports with b pages and 3 science reports with s pages during the school year.
Multiply 3 book reports with b pages = 3b.
Multiply 3 science books with s pages = 3s.
The algebraic expression for the total number of pages Rachel will need to write is 3b + 3s.
Question 14.
Rachel’s friend Yassi has to write 3(b + s) pages for reports. Use properties of operations to determine whether this expression is equivalent to the expression for the number of pages Rachel has to write.
This expression is _____________
Answer: equivalent
Explanation:
Rachel’s friend Yassi has to write 3(b + s) pages for reports.
The equivalent expression of 3(b + s) = 3b + 3s
Question 15.
Use properties of operations to show whether 7y + 7b + 3y and 7(y + b) + 3b are equivalent expressions. Explain your reasoning.
Type below:
_____________
Answer:
Use Distributive property to simplify the expressions.
The equivalent expression of 7y + 7b + 3y = 7(y + b) + 3y
Thus 7y + 7b + 3y and 7(y + b) + 3b are equivalent.
Lesson Check – Page No. 412
Question 1.
Ian had 4 cases of comic books and 6 adventure books. Each case holds c comic books. He gave 1 case of comic books to his friend. Write an expression that gives the total number of books Ian has left.
Type below:
_____________
Answer: 3c + 6
Explanation:
Ian had 4 cases of comic books and 6 adventure books. Each case holds c comic books. He gave 1 case of comic books to his friend.
4c + 6 – 1c
Combine the like terms
3c + 6
Question 2.
In May, Xia made 5 flower planters with f flowers in each planter. In June, she made 8 flower planters with f flowers in each planter. Write an expression in the simplest form that gives the number of flowers Xia has in the planters.
Type below:
_____________
Answer: 13f
Explanation:
In May, Xia made 5 flower planters with f flowers in each planter.
The expression is 5f
In June, she made 8 flower planters with f flowers in each planter.
The expression is 8f.
Sum of 5f and 8f is 8f + 5f = 13f
Spiral Review
Question 3.
Keisha wants to read for 90 minutes. So far, she has read 30% of her goal. How much longer does she need to read to reach her goal?
________ minutes
Answer: 63 min
Explanation:
Keisha wants to read for 90 minutes.
So far, she has read 30% of her goal.
30% = 30/100 = 0.3
Multiply 90 with 0.3
90 × 0.3 = 27
Subtract 27 from 90
90 – 27 = 63
She needs to read 63 minutes to reach her goal.
Question 4.
Marvyn travels 105 miles on his scooter. He travels for 3 hours. What is his average speed?
________ miles per hour
Answer: 35 miles per hour
Explanation:
Divide the number of miles by hours traveled.
Average speed = 105 miles/3 hours = 35 miles per hour
Thus the average speed is 35 miles per hour.
Question 5.
The expression 5(F − 32) ÷ 9 gives the Celsius temperature for a Fahrenheit temperature of F degrees. The noon Fahrenheit temperature in Centerville was 86 degrees. What was the temperature in degrees Celsius?
________ degrees Celsius
Answer: 30 degrees Celsius
Explanation:
The expression is 5(F − 32) ÷ 9
F = 86 degrees
Substitute F in the above expression.
5(86 − 32) ÷ 9 = 5(54) ÷ 9
270 ÷ 9 = 30
The temperature is 30 degrees Celsius
Question 6.
At the library book sale, hardcover books sell for $4 and paperbacks sell for $2. The expression 4b + 2b represents the total cost for b hardcover books and b paperbacks. Write a simpler expression that is equivalent to 4b + 2b.
Type below:
_____________
Answer: 6b
Explanation:
Given expression is 4b + 2b
The terms are 4b and 2b
Now combine the like terms
That means 4b + 2b = 6b
Chapter 7 Review/Test – Page No. 413
Question 1.
Use exponents to rewrite the expression.
3 × 3 × 3 × 3 × 5 × 5
Type below:
_____________
Answer: 34 × 52
Explanation:
3 is a repeated factor.
The number 3 is repeated four times.
5 is a repeated factor.
The number 5 is repeated two times.
The exponential form of 3 × 3 × 3 × 3 × 5 × 5 is 34 × 52
Question 2.
A plumber charges $10 for transportation and $55 per hour for repairs. Write an expression that can be used to find the cost in dollars for a repair that takes h hours.
Type below:
_____________
Answer: 10 + 55h
Explanation:
A plumber charges $10 for transportation and $55 per hour for repairs.
Multiply 55 with an hour
Sum of 10 and product of 55 and h.
The expression is 10 + 55h.
Question 3.
Ellen is 2 years older than her brother Luke. Let k represent Luke’s age. Identify the expression that can be used to find Ellen’s age.
Options:
a. k−2
b. k+2
c. 2k
d. \(\frac{k}{2}\)
Answer: k+2
Explanation:
Given, Ellen is 2 years older than her brother Luke. Let k represent Luke’s age.
Older is nothing but more so we have to add 2 years to k.
That means k + 2.
Thus the correct answer is option B.
Question 4.
Write 43 using repeated multiplication. Then find the value of 43.
________
Answer:
43 = 4 × 4 × 4 = 64
The value of 43 is 64.
Question 5.
Jasmine is buying beans. She bought r pounds of red beans that cost $3 per pound and b pounds of black beans that cost $2 per pound. The total amount of her purchase is given by the expression 3r + 2b. Select the terms of the expression. Mark all that apply
Options:
a. 2
b. 2b
c. 3
d. 3r
Answer: B, D
Explanation:
The expression is 3r + 2b
The terms of the expressions are 3r and 2b.
Thus the correct answers are B and D.
Chapter 7 Review/Test – Page No. 414
Question 6.
Choose the number that makes the sentence true. The formula V= s3 gives the volume V of a cube with side length s.
The volume of a cube that has a side length of 8 inches
inches is _____________ cubed
Answer: 512
Explanation:
Use the formula V= s3
s = 8
V = 83 = 8 × 8 × 8 = 512
Question 7.
Liang is ordering new chairs and cushions for his dining room table. A new chair costs $88 and a new cushion costs $12. Shipping costs $34. The expression 88c + 12c + 34 gives the total cost for buying c sets of chairs and cushions. Simplify the expression by combining like terms.
Type below:
_____________
Answer: 100c + 34
Explanation:
Liang is ordering new chairs and cushions for his dining room table.
A new chair costs $88 and a new cushion costs $12. Shipping costs $34.
The expression is 88c + 12c + 34.
Combine the like terms
88c + 12c + 34 = 100c + 34
Question 8.
Mr. Ruiz writes the expression 5 × (2 + 1)2 ÷ 3 on the board. Chelsea says the first step is to evaluate 12. Explain Chelsea’s mistake. Then, evaluate the expression
_____________
Answer:
She should have done what was in the parentheses (2 + 1) and then the exponent 32= 9
5 × (2 + 1)2 ÷ 3 = 5 × 9 ÷ 3
5 × 3 = 15
Question 9.
Jake writes this word expression.

Write an algebraic expression for the word expression. Then, evaluate the expression for m = 4. Show your work.
________
Answer:
The expression is 7m
Replace m = 4 with m
7m = 7 × 4 = 28
Chapter 7 Review/Test – Page No. 415
Question 10.
Sora has some bags that each contain 12 potatoes. She takes 3 potatoes from each bag. The expression 12p – 3p represents the number of potatoes p left in the bags. Simplify the expression by combining like terms. Draw a line to match the expression with the simplified expression.
Type below:
_____________
Answer: 9p
Question 11.
Logan works at a florist. He earns $600 per week plus $5 for each floral arrangement he delivers. Write an expression that gives the amount in dollars that Logan earns for delivering f floral arrangements. Use the expression to find the amount Logan will earn if he delivers 45 floral arrangements in one week. Show your work.
$ ________
Answer: $825
Explanation:
Logan works at a florist. He earns $600 per week plus $5 for each floral arrangement he delivers. Write an expression that gives the amount in dollars that Logan earns for delivering f floral arrangements.
The expression is 600 + 5f
f = 45
600 + 5f = 600 + 5(45)
600 + 225 = 825
Thus Logan earned $825 for delivering f floral arrangements.
Question 12.
Choose the word that makes the sentence true.
Dara wrote the expression 7 × (d + 4) in her notebook. She used the _____ Property to write the equivalent expression 7d + 28.
Answer: Dara wrote the expression 7 × (d + 4) in her notebook. She used the Distributive Property to write the equivalent expression 7d + 28.
Use the distributive property to simplify the expression.
7 × (d + 4) = 7d + 28
Chapter 7 Review/Test – Page No. 416
Question 13.
Use properties of operations to determine whether 5(n + 1) + 2n and 7n + 1 are equivalent expressions.
The expressions are _____________
Answer:
5n + 5 + 2n is Distributive property
5n + 2n + 5 is Commutative property of addition
7n + 5 combine like term
5n + 5 + 2n is equivalent to 7n + 5
Since it is not equivalent to 7n + 1, 7n + 5 is not equivalent to 7n.
Question 14.
Alisha buys 5 boxes of peanut butter granola bars and 5 boxes of cinnamon granola bars. Let p represent the number of bars in a box of peanut butter granola bars and c represents the number of bars in a box of cinnamon granola bars. Jaira and Emma each write an expression that represents the total number of granola bars Alisha bought. Are the equivalent of the expression? Justify your answer
Jaira
5p + 5c
Emma
5(p + c)
Type below:
_____________
Answer:
They are equivalent statements.
5p + 5c = 5(p + c) by the distributive property.
Question 15.
Abe is 3 inches taller than Chen. Select the expressions that represent Abe’s height if Chen’s height is h inches. Mark all that apply
Options:
a. h−3
b. h+3
c. the sum of h and 3
d. the difference between h and 3
Answer:
Abe is 3 inches taller than Chen.
Let Chen’s height is h.
The expression is the sum of Chen’s height and 3.
So, the suitable answers are h + 3 and the sum of h and 3.
Thus the correct answers are option B and C.
Question 16.
Write the algebraic expression in the box that shows an equivalent expression.
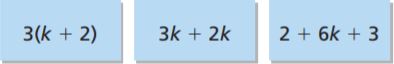
Type below:
_____________
Answer:
3(k + 2) = 3k + 6
3k + 2k = 5k
2 + 6k + 3 = 6k + 5
Chapter 7 Review/Test – Page No. 417
Question 17.
Draw a line to match the property with the expression that shows the property.

Type below:
_____________
Answer:
Question 18.
A bike rental company charges $10 to rent a bike plus $2 for each hour the bike is rented. An expression for the total cost of renting a bike for h hours is 10 + 2h. Complete the table to find the total cost of renting a bike for h hours.
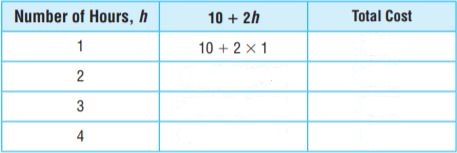
Type below:
_____________
Answer:
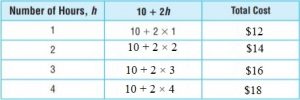
Question 19.
An online sporting goods store charges $12 for a pair of athletic socks. Shipping is $2 per order
Part A
Write an expression that Hana can use to find the total cost in dollars for ordering n pairs of socks.
Type below:
_____________
Answer: 12n + 2
Explanation:
Let n represents a pair of socks.
Multiply the price of pair of athletic socks with pair of socks = 12 × n
Shipping is $2 per order
The expression for the total cost in dollars for ordering n pairs of socks is 12n + 2
Question 19.
Part B
Hana orders 3 pairs of athletic socks and her friend, Charlie, order 2 pairs of athletic socks. What is the total cost, including shipping, for both orders? Show your work.
$ ________
Answer:
The cost of Hannah’s order is 12 × 3 + 2 = 36 + 2 = 38
The cost of Charlie’s order is 12 × 2 + 2 = 24 + 2 = 26
The total cost for both is 38 + 26 = 64
Chapter 7 Review/Test – Page No. 418
Question 20.
Fernando simplifies the expression (6 + 2)2 – 4 × 3.
Part A
Fernando shows his work on the board. Use numbers and words to explain his mistake.
(6 + 2)2 – 4 × 3
(6 + 4) – 4 × 3
10 − 4 × 3
6 × 3
18
Type below:
_____________
Answer: Fernando did not use the correct order of operations. He should have added 6 and 2, then evaluate the exponent. He also subtracted before multiplying. He should have multiplied first.
Question 20.
Part B
Simplify the expression (6 + 2)2 − 4 × 3 using the order of operations.
_______
Answer: 52
Explanation:
(6 + 2)2 − 4 × 3
First, add 6 and 2 and then subtract with 12.
82 – 4 × 3
= 64 – 12 = 52
(6 + 2)2 − 4 × 3 = 52
Conclusion:
In addition to the exercise and homework problems, we have given the solutions for the mid-chapter and review test. Hence the students of grade 6 can check whether the answers are right or wrong. Feel free to post your comments in the below comment box if you have any queries. Bookmark our ccssmathanswers.com to get the go math answer key for all grade 6 chapters.
