Engage NY Eureka Math 3rd Grade Module 5 Lesson 23 Answer Key
Eureka Math Grade 3 Module 5 Lesson 23 Sprint Answer Key
A
Add by Six
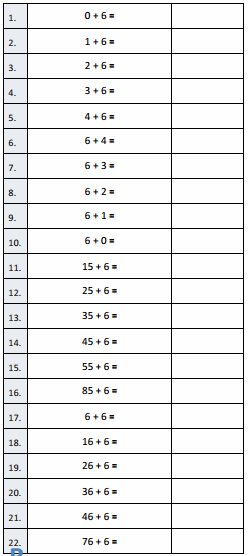
Question 1.
0 + 6 =
Question 2.
1 + 6 =
Question 3.
2 + 6 =
Question 4.
3 + 6 =
Question 5.
4 + 6 =
Question 6.
6 + 4 =
Question 7.
6 + 3 =
Question 8.
6 + 2 =
Question 9.
6 + 1 =
Question 10.
6 + 0 =
Question 11.
15 + 6 =
Question 12.
25 + 6 =
Question 13.
35 + 6 =
Question 14.
45 + 6 =
Question 15.
55 + 6 =
Question 16.
85 + 6 =
Question 17.
6 + 6 =
Question 18.
16 + 6 =
Question 19.
26 + 6 =
Question 20.
36 + 6 =
Question 21.
46 + 6 =
Question 22.
76 + 6 =
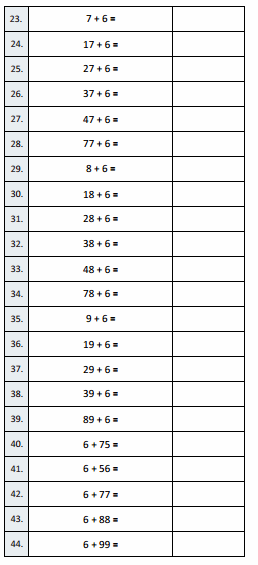
Question 23.
7 + 6 =
Question 24.
17 + 6 =
Question 25.
27 + 6 =
Question 26.
37 + 6 =
Question 27.
47 + 6 =
Question 28.
77 + 6 =
Question 29.
8 + 6 =
Question 30.
18 + 6 =
Question 31.
28 + 6 =
Question 32.
38 + 6 =
Question 33.
48 + 6 =
Question 34.
78 + 6 =
Question 35.
9 + 6 =
Question 36.
19 + 6 =
Question 37.
29 + 6 =
Question 38.
39 + 6 =
Question 39.
89 + 6 =
Question 40.
6 + 75 =
Question 41.
6 + 56 =
Question 42.
6 + 77 =
Question 43.
6 + 88 =
Question 44.
6 + 99 =
B
Add by Six
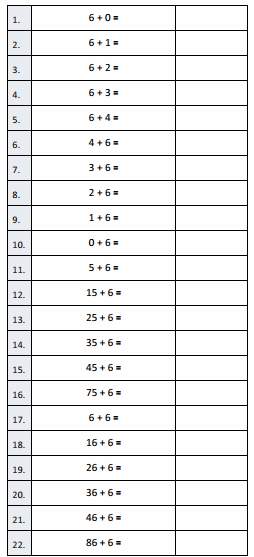
Question 1.
6 + 0 =
Question 2.
6 + 1 =
Question 3.
6 + 2 =
Question 4.
6 + 3 =
Question 5.
6 + 4 =
Question 6.
4 + 6 =
Question 7.
3 + 6 =
Question 8.
2 + 6 =
Question 9.
1 + 6 =
Question 10.
0 + 6 =
Question 11.
5 + 6 =
Question 12.
15 + 6 =
Question 13.
25 + 6 =
Question 14.
35 + 6 =
Question 15.
45 + 6 =
Question 16.
75 + 6 =
Question 17.
6 + 6 =
Question 18.
16 + 6 =
Question 19.
26 + 6 =
Question 20.
36 + 6 =
Question 21.
46 + 6 =
Question 22.
86 + 6 =
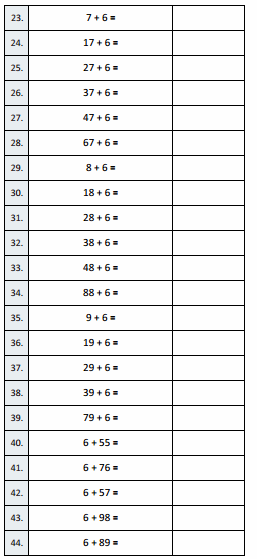
Question 23.
7 + 6 =
Question 24.
17 + 6 =
Question 25.
27 + 6 =
Question 26.
37 + 6 =
Question 27.
47 + 6 =
Question 28.
67 + 6 =
Question 29.
8 + 6 =
Question 30.
18 + 6 =
Question 31.
28 + 6 =
Question 32.
38 + 6 =
Question 33.
48 + 6 =
Question 34.
88 + 6 =
Question 35.
9 + 6 =
Question 36.
19 + 6 =
Question 37.
29 + 6 =
Question 38.
39 + 6 =
Question 39.
79 + 6 =
Question 40.
6 + 55 =
Question 41.
6 + 76 =
Question 42.
6 + 57 =
Question 43.
6 + 98 =
Question 44.
6 + 89 =

Eureka Math Grade 3 Module 5 Lesson 23 Problem Set Answer Key
Question 1.
On the number line above, use a red colored pencil to divide each whole into fourths, and label each fraction above the line. Use a fraction strip to help you estimate, if necessary.
Answer :
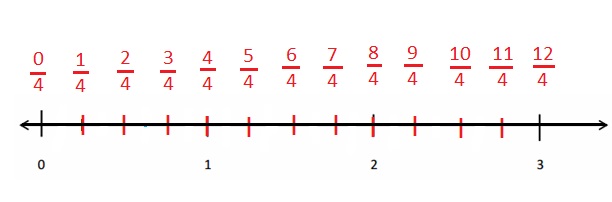
Question 2.
On the number line above, use a blue colored pencil to divide each whole into eighths, and label each fraction below the line. Refold your fraction strip from Problem 1 to help you estimate.
Answer :
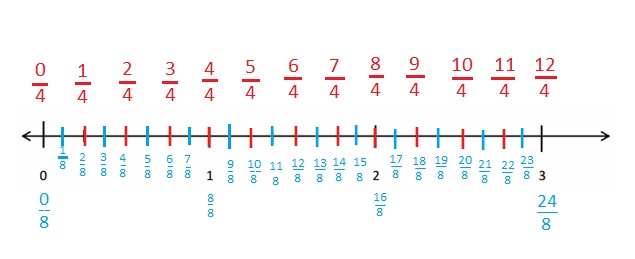
Question 3.
List the fractions that name the same place on the number line.
Answer :
The Fractions that have same place on the number line are
\(\frac{1}{4}\) = \(\frac{2}{8}\)
\(\frac{2}{4}\) = \(\frac{4}{8}\)
\(\frac{3}{4}\) = \(\frac{6}{8}\)
\(\frac{4}{4}\) = \(\frac{8}{8}\)
\(\frac{5}{4}\) = \(\frac{10}{8}\)
\(\frac{6}{4}\) = \(\frac{12}{8}\)
\(\frac{7}{4}\) = \(\frac{14}{8}\)
\(\frac{8}{4}\) = \(\frac{16}{8}\)
\(\frac{9}{4}\) = \(\frac{18}{8}\)
\(\frac{10}{4}\) = \(\frac{20}{8}\)
\(\frac{11}{4}\) = \(\frac{22}{8}\)
\(\frac{12}{4}\) = \(\frac{24}{8}\)
Question 4.
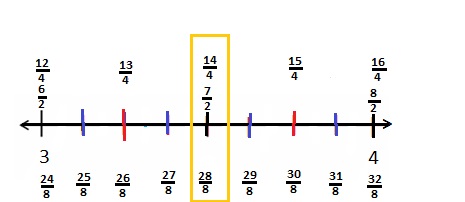
Using your number line to help, what red fraction and what blue fraction would be equal to \(\frac{7}{2}\)? Draw the part of the number line below that would include these fractions, and label it.
Answer :
\(\frac{7}{2}\) = \(\frac{14}{4}\) = \(\frac{28}{8}\).
Question 5.
Write two different fractions for the dot on the number line. You may use halves, thirds, fourths, fifths, sixths, or eighths. Use fraction strips to help you, if necessary.
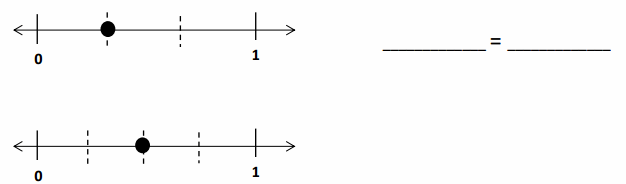
_____________ = _____________
_____________ = _____________
_____________ = _____________
Answer :
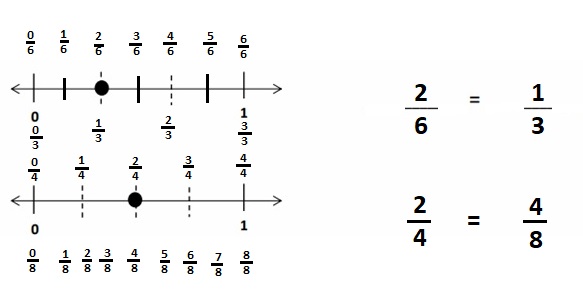
Explanation :
The given 1 st number line is divided into 3 parts that means it can be partitioned into thirds and sixths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{2}{6}\) = \(\frac{1}{3}\) .
The given 2nd number line is divided into 4 parts that means it can be partitioned intoFourths and Eighths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{2}{4}\) = \(\frac{4}{8}\) .
Question 6.
Cameron and Terrance plan to run in the city race on Saturday. Cameron has decided that he will divide his race into 3 equal parts and will stop to rest after running 2 of them. Terrance divides his race into 6 equal parts and will stop and rest after running 2 of them. Will the boys rest at the same spot in the race? Why or why not? Draw a number line to explain your answer.
Answer :
No, they don’t spot in the same place .
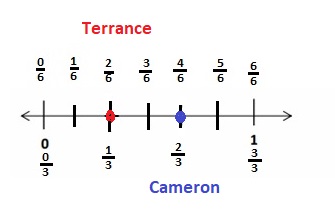
Explanation :
The number line is divided into thirds and sixths .
The Cameron partitioned the race into 3 parts and fraction values are written below the number line .
The Cameron stop and rest after running 2 of them = \(\frac{2}{3}\) .
The Terrance partitioned the race into 6 parts and fraction values are written above the number line .
The Terrance stop and rest after running 2 of them = \(\frac{2}{6}\) .
That means they don’t spot the same place for rest .
Question 7.
Henry and Maddie were in a pie-eating contest. The pies were cut either into thirds or sixths. Henry picked up a pie cut into sixths and ate \(\frac{4}{6}\) of it in 1 minute. Maddie picked up a pie cut into thirds. What fraction of her pie does Maddie have to eat in 1 minute to tie with Henry? Draw a number line, and use words to explain your answer.
Answer :
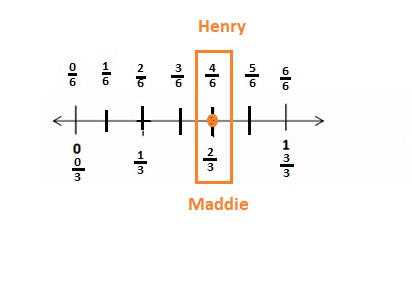
Explanation :
Henry pie is cut in sixths.
Number of of pies ate by henry in 1 minute= \(\frac{4}{6}\)
Maddie pie cut in thirds.
Number of of pies ate by Maddie in 1 minute= ?
For tie Maddie should eat same quantity as Henry that means the point \(\frac{4}{6}\) is marked says number of pies Maddie should eat in thirds .
Number of of pies ate by Maddie in 1 minute=\(\frac{2}{3}\) .
Eureka Math Grade 3 Module 5 Lesson 23 Homework Answer Key

Question 1.
On the number line above, use a colored pencil to divide each whole into thirds and label each fraction above the line.
Answer :
Explanation :
Number line from 0 to 3 is partitioned into thirds . and all the fraction values are written above the number line .
Question 2.
On the number line above, use a different colored pencil to divide each whole into sixths and label each fraction below the line.
Answer :
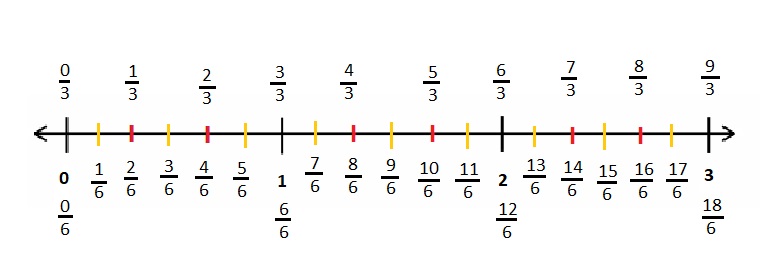
Explanation :
Number line from 0 to 3 is partitioned into sixths . and all the fraction values are written below the number line .
Question 3.
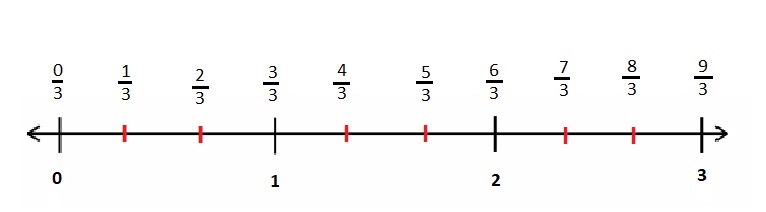
Write the fractions that name the same place on the number line.
Answer :
The Fractions that have same place on the number line are
\(\frac{1}{3}\) = \(\frac{2}{6}\)
\(\frac{2}{3}\) = \(\frac{4}{6}\)
\(\frac{3}{3}\) = \(\frac{6}{6}\)
\(\frac{4}{3}\) = \(\frac{8}{6}\)
\(\frac{5}{3}\) = \(\frac{10}{6}\)
\(\frac{6}{3}\) = \(\frac{12}{6}\)
\(\frac{7}{3}\) = \(\frac{14}{6}\)
\(\frac{8}{3}\) = \(\frac{16}{6}\)
\(\frac{9}{3}\) = \(\frac{18}{6}\)
Question 4.
Using your number line to help, name the fraction equivalent to \(\frac{20}{6}\). Name the fraction equivalent to \(\frac{12}{3}\). Draw the part of the number line that would include these fractions below, and label it.

Answer :
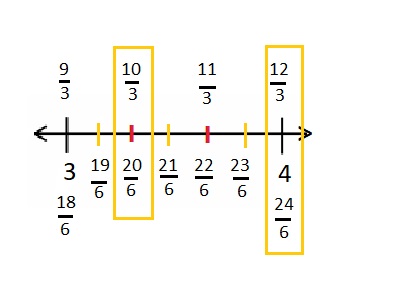
Explanation :
Number line is partitioned from 3 to 4 into thirds and sixths and all the thirds fraction values are written above number line and sixths fraction values are written below the number line .
We notice \(\frac{20}{6}\) = \(\frac{10}{3}\) and \(\frac{12}{3}\) = \(\frac{24}{6}\) .
Question 5.
Write two different fraction names for the dot on the number line. You may use halves, thirds, fourths, fifths, sixths, eighths, or tenths.
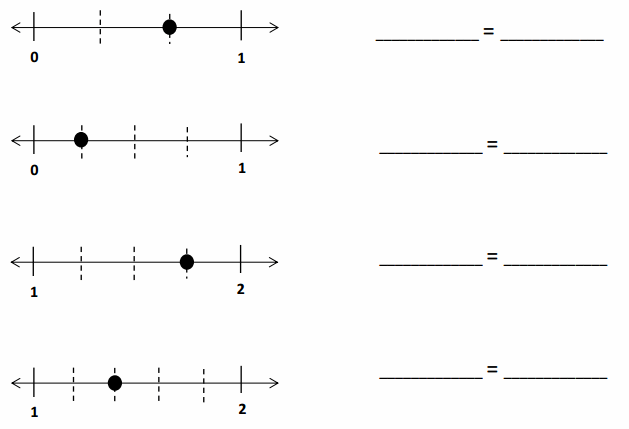
Answer :
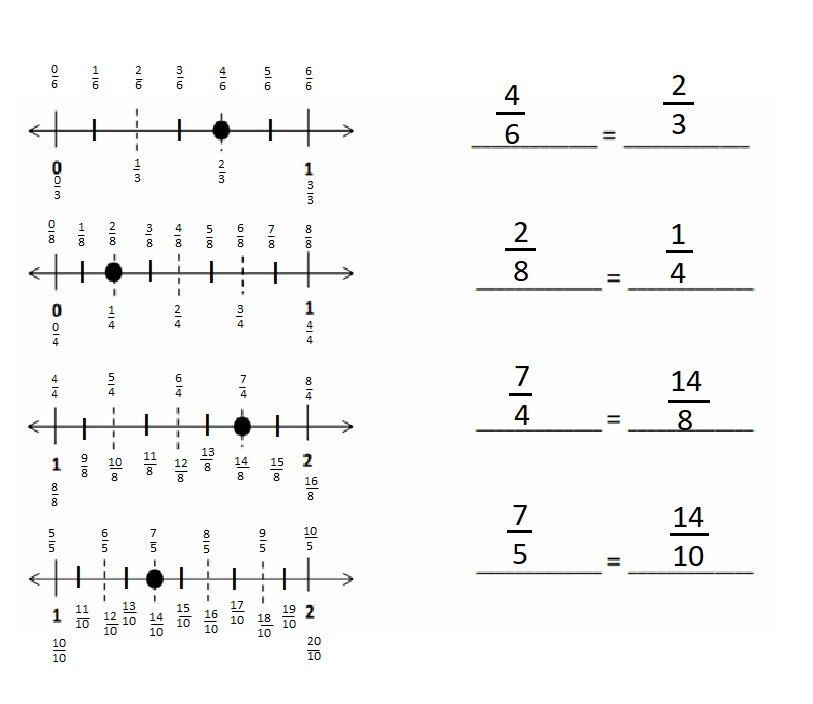
Explanation :
The given 1 st number line is divided into 3 parts that means it can be partitioned into thirds and sixths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{4}{6}\) = \(\frac{2}{3}\) .
The given 2nd number line is divided into 4 parts that means it can be partitioned into Fourths and Eighths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{2}{8}\) = \(\frac{1}{4}\) .
The given 3rd number line is divided into 4 parts that means it can be partitioned into Fourths and Eighths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{7}{4}\) = \(\frac{14}{8}\) .
The given 4th number line is divided into 5 parts that means it can be partitioned into fifths and tenths .
All fraction numbers are written and at a given point we notice the fraction value is \(\frac{7}{5}\) = \(\frac{14}{10}\) .
Question 6.
Danielle and Mandy each ordered a large pizza for dinner. Danielle’s pizza was cut into sixths, and Mandy’s pizza was cut into twelfths. Danielle ate 2 sixths of her pizza. If Mandy wants to eat the same amount of pizza as Danielle, how many slices of pizza will she have to eat? Write the answer as a fraction. Draw a number line to explain your answer.
Answer :
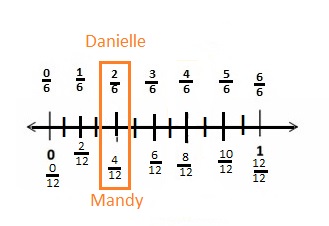
Explanation :
Danielle pizza is cut in sixths.
Number of pizza pieces ate by Danielle = \(\frac{2}{6}\)
Mandy pizza cut in twelfths.
Number of pizza pieces ate by Mandy= ?
For same Quantity Mandy should eat same quantity as Danielle that means the point \(\frac{2}{6}\) is marked says number of pizza pieces Mandy should eat in twelfths .
Number of pizza pieces should eat by mandy =\(\frac{4}{12}\) .