Engage NY Eureka Math 7th Grade Module 5 Lesson 9 Answer Key
Eureka Math Grade 7 Module 5 Lesson 9 Exploratory Challenge Answer Key
Exploratory Challenge: Game Show—Picking Blue!
Imagine, for a moment, the following situation: You and your classmates are contestants on a quiz show called Picking Blue! There are two bags in front of you, Bag A and Bag B. Each bag contains red and blue chips. You are told that one of the bags has exactly the same number of blue chips as red chips. But you are told nothing about the ratio of blue to red chips in the other bag.
Each student in your class will be asked to select either Bag A or Bag B. Starting with Bag A, a chip is randomly selected from the bag. If a blue chip is drawn, all of the students in your class who selected Bag A win a blue token. The chip is put back in the bag. After mixing up the chips in the bag, another chip is randomly selected from the bag. If the chip is blue, the students who picked Bag A win another blue token. After the chip is placed back into the bag, the process continues until a red chip is picked. When a red chip is picked, the game moves to Bag B. A chip from Bag B is then randomly selected. If it is blue, all of the students who selected Bag B win a blue token. But if the chip is red, the game is over. Just like for Bag A, if the chip is blue, the process repeats until a red chip is picked from the bag. When the game is over, the students with the greatest number of blue tokens are considered the winning team.
Without any information about the bags, you would probably select a bag simply by guessing. But surprisingly, the show’s producers are going to allow you to do some research before you select a bag. For the next 20 minutes, you can pull a chip from either one of the two bags, look at the chip, and then put the chip back in the bag. You can repeat this process as many times as you want within the 20 minutes. At the end of 20 minutes, you must make your final decision and select which of the bags you want to use in the game.
Getting Started
Assume that the producers of the show do not want to give away a lot of their blue tokens. As a result, if one bag has the same number of red and blue chips, do you think the other bag would have more or fewer blue chips than red chips? Explain your answer.
Answer:
The producers would likely want the second bag to have fewer blue chips. If a participant selects that bag, it would mean the participant is more likely to lose this game.
Eureka Math Grade 7 Module 5 Lesson 9 Examining Your Results Answer Key
At the end of the game, your teacher will open the bags and reveal how many blue and red chips were in each bag. Answer the questions that follow. After you have answered these questions, discuss them with your class.
Question 1.
Before you played the game, what were you trying to learn about the bags from your research?
Answer:
I was trying to learn the estimated probability of picking a blue chip without knowing the theoretical probability.
Question 2.
What did you expect to happen when you pulled chips from the bag with the same number of blue and red chips? Did the bag that you thought had the same number of blue and red chips yield the results you expected?
Answer:
I was looking for an estimated probability that was close to 0.5. That would connect the estimated probability of picking blue with the bag that had the same number of red and blue chips.
Question 3.
How confident were you in predicting which bag had the same number of blue and red chips? Explain.
Answer:
Answers will vary. Students’ confidence is based on the data collected. The more data collected, the closer the estimates are likely to be to the actual probabilities.
Question 4.
What bag did you select to use in the competition, and why?
Answer:
Answers will vary. It is anticipated that students would pick the bag with the larger estimated probability of picking a blue chip based on the many chips they selected during the research stage. Evidence of choices might include I picked 50 chips from Bag A, and 24 were blue, and 26 were red. The results are very close to 0.5 probability of picking each color. I think this indicates that there were likely an equal number of each color in this bag.
Question 5.
If you were the show’s producers, how would you make up the second bag? (Remember, one bag has the same number of red and blue chips.)
Answer:
Answers will vary. Most students should indicate a second bag that had fewer blue chips, but some may speculate on a second bag that is nearly the same (to make the game more of a guessing game) or a bag with very few blue (thus providing a clearer indication which bag had the same number of red and blue chips).
Question 6.
If you picked a chip from Bag B 100 times and found that you picked each color exactly 50 times, would you know for sure that Bag B was the one with equal numbers of each color?
Answer:
Student answers should indicate that they are quite confident they have the right bag, as getting that result is likely to occur from the bag with equal numbers of each colored chip. However, answers should represent understanding that even a bag with a different number of colored chips could have that outcome.
Eureka Math Grade 7 Module 5 Lesson 9 Problem Set Answer Key
Jerry and Michael played a game similar to Picking Blue! The following results are from their research using the same two bags:
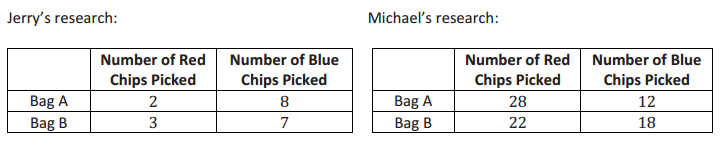
Question 1.
If all you knew about the bags were the results of Jerry’s research, which bag would you select for the game? Explain your answer.
Answer:
Using only Jerry’s research, I would select Bag A because the greater relative frequency of picking a blue chip would be Bag A, or 0.8. There were 10 selections. Eight of the selections resulted in picking blue.
Question 2.
If all you knew about the bags were the results of Michael’s research, which bag would you select for the game? Explain your answer.
Answer:
Using Michael’s research, I would select Bag B because the greater relative frequency of picking a blue chip would be Bag B, or \(\frac{18}{40}\) = 0.45. There were 40 selections. Eighteen of the selections resulted in picking blue.
Question 3.
Does Jerry’s research or Michael’s research give you a better indication of the makeup of the blue and red chips in each bag? Explain why you selected this research.
Answer:
Michael’s research would provide a better indication of the probability of picking a blue chip, as it was carried out 40 times compared to 10 times for Jerry’s research. The more outcomes that are carried out, the closer the relative frequencies approach the theoretical probability of picking a blue chip.
Question 4.
Assume there are 12 chips in each bag. Use either Jerry’s or Michael’s research to estimate the number of red and blue chips in each bag. Then, explain how you made your estimates.
Bag A
Number of red chips:
Number of blue chips:
Bag B
Number of red chips:
Number of blue chips:
Answer:
Answers will vary. Anticipate that students see Bag B as the bag with nearly the same number of blue and red chips and Bag A as possibly having a third of the chips blue. Answers provided by students should be based on the relative frequencies. A sample answer based on this reasoning is
Bag A: 4 blue chips and 8 red chips
Bag B: 6 blue chips and 6 red chips
Question 5.
In a different game of Picking Blue!, two bags each contain red, blue, green, and yellow chips. One bag contains the same number of red, blue, green, and yellow chips. In the second bag, half of the chips are blue. Describe a plan for determining which bag has more blue chips than any of the other colors.
Answer:
Students should describe a plan similar to the plan implemented in the lesson. Students would collect data by selecting chips from each bag. After several selections, the estimated probabilities of selecting blue from each bag would suggest which bag has more blue chips than chips of the other colors.
Eureka Math Grade 7 Module 5 Lesson 9 Exit Ticket Answer Key
As this is an exploratory lesson, the Exit Ticket is incorporated into the closing questions of Examining Your Results.