Students who are looking for the practice material on linear equations can stay tuned to this page. Here, we are giving several problems on linear equations. Interested students can solve them by using addition, subtraction, multiplication, and division operation. You can also find the step by step solution guide for each and every question. While practicing this Linear Equations Word Problems just have a look at the important notes mentioned below.
How to Solve Linear Equations?
Go through the below steps on solving linear equations. Follow them and arrive at the solutions easily. They are listed as under
- A linear equation in one variable is one that contains only one variable and its highest power is 1.
- One can add or subtract the same number to both sides of the equation.
- One can divide or multiply by both sides of the equation by the same non-zero integer.
- The process in which any term in the equation can be moved to the other side of the equal symbol by changing its sign from (+ to -), (- to +), (x to ÷), and (÷ to x) is called the transposition.
- Cross multiplication means the process of multiplying the numerator of L.H.S with the denominator of the R.H.S and multiplying the denominator of L.H.S with the numerator of R.H.S.
Linear Equations Questions and Answers
Example 1.
Solve the following linear equations.
(a) (3 – 7x)/(15 + 2x) = 1
(b) 8x + 9 – 3x = 8 + 4x + 1
(c) 3x – 12 = 0
Solution:
(a) (3 – 7x)/(15 + 2x) = 1
Multiply both sides by (15 + 2x).
(3 – 7x) = 1(15 + 2x)
3 – 7x = 15 + 2x
Transfer – 7x to R.H.S becomes 7x
3 = 15 + 2x + 7x
3 = 15 + 9x
Transfer 15 from R.H.S to L.H.s becomes -15.
3 – 15 = 9x
-12 = 9x
Divide both sides by 9
9x / 9 = -12/9
x = -4/3
Therefore required solution is x = -4/3.
(b) 8x + 9 – 3x = 8 + 4x + 1
5x + 9 = 9 + 4x
Transfer 4x from R.H.S to L.H.S becomes -4x
5x – 4x + 9 = 0
x + 9 = 0
Subtract both sides from -9
x + 9 – 9 = 0 – 9
x = -9
Therefore, required solution set is x = -9.
(c) 3x – 12 = 0
3x = 12
Divide both sides of the equation by 3.
3x/3 = 12/3
x = 4
Therefore, required solution set is x = 4.
Example 2.
Solve the following equations and represent them on a graph.
(a) (3y – 2)/3 + (2y + 3)/3 = (y + 7)/6
(b) 5x – 11 = 3x + 9
(c) (0.5y – 9)/0.25 = 4y – 3
Solution:
(a) (3y – 2)/3 + (2y + 3)/3 = (y + 7)/6
[(3y – 2) + (2y + 3)] / 3 = (y + 7) / 6
(5y + 1) = (y + 7) / 2
Multiply both sides by 2.
2(5y + 1) = (y+7) / 2 x 2
10y + 2 = y + 7
Transferring y from R.H.S to L.H.S becomes -y, 2 from L.H.S to R.H.S becomes -2.
10y – y = 7 – 2
9y = 5
y = 5/9
Therefore, the required solution set is y = 5/9.

(b) 5x – 11 = 3x + 9
Transferring 3x from R.H.S to L.H.S becomes -3x, -11 from L.H.S to R.H.S becomes 11.
5x – 3x = 9 + 11
2x = 20
Divide both sides by 2.
2x/2 = 20/2
x = 10.
Therefore, the required solution set is x = 10
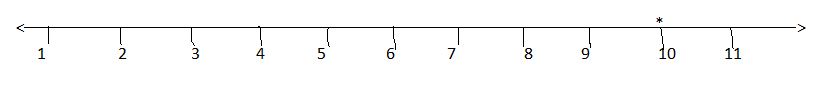
(c) (0.5y – 9)/0.25 = 4y – 3
Multiply both sides by 0.25.
(0.5y – 9)/0.25 x 0.25 = (4y – 3)0.25
0.5y – 9 = y – 0.75
Transferring 0.5y from L.H.S to R.H.S becomes -0.5y, -0.75 from R.h.s to L.H.S becomes 0.75.
-9 + 0.75 = y – 0.5y
-8.25 = 0.5y
Divide both sides by 0.5.
0.5y/0.5 = -8.25/0.5
y = -16.5
Therefore, the required solution set is y = -16.5
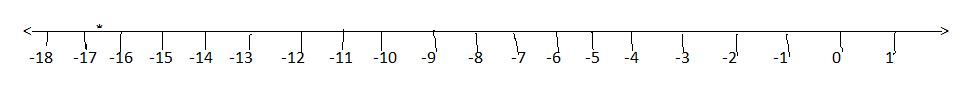
Example 3.
Solve the equations and verify them.
(a) (x – 3)/4 + (x – 1)/5 – (x – 2)/3 = 1
(b) y/2 – 1/2 = y/3 + 1/4
Solution:
(a) (x – 3)/4 + (x – 1)/5 – (x – 2)/3 = 1
L.C.M of 4, 5, 3 is 60.
[15(x – 3) + 12(x – 1) – 20(x – 2)] / 60 = 1
Multiply both sides of the equation by 60.
[15(x – 3) + 12(x – 1) – 20(x – 2)] / 60 x 60 = 1 x 60
[15(x – 3) + 12(x – 1) – 20(x – 2)] = 60
15x – 45 + 12x – 12 – 20x + 40 = 60
27x – 20x – 57 + 40 = 60
7x – 17 = 60
7x = 60 + 17
7x = 77
x = 77/7
x = 11
Therefore, the required solution set is x = 11.
Verification:
L.H.S = (x – 3)/4 + (x – 1)/5 – (x – 2)/3
Substitute x = 11
L.H.S = (11 – 3)/4 + (11 – 1)/5 – (11 – 2)/3
= 8/4 + 10/5 – 9/3
= 2 + 2 – 3
= 4 – 3 = 1
Hence, L.H.S = R.H.S
(b) y/2 – 1/2 = y/3 + 1/4
(y – 1)/2 = (4y + 3)/12
Cross multiply the fractions.
12(y – 1) = 2(4y + 3)
12y – 12 = 8y + 6
12y – 8y = 6 + 12
4y = 18
y = 18/4
y = 4.5
Verification:
L.H.S = y/2 – 1/2
Substitute y = 4.5
L.H.S = 4.5/2 – 1/2
= (4.5 – 1)/2
= 3.5/2 = 1.75
R.H.S = y/3 + 1/4
Substitute y = 4.5
R.H.S = 4.5/3 + 1/4
= (4.5 x 4 + 3) / 12
= (18 + 3) / 12
= 21/12 = 1.75
Hence, L.H.S = R.H.S
Example 4.
Solve the below-mentioned linear equations.
(a) 8a – (4a + 32) = 16
(b) 4(x + 5) = 3(x – 2) – 2(x + 2)
Solution:
(a) 8a – (4a + 32) = 16
8a – 4a – 32 = 16
4a – 32 = 16
Transferring -32 from L.H.S to R.H.S becomes +32.
4a = 16 + 32
4a = 48
Divide both sides of the equation by 4.
4a/4 = 48/4
a = 12
Therefore, the required solution set a = 12.
(b) 4(x + 5) = 3(x – 2) – 2(x + 2)
4x + 20 = 3x – 6 – 2x – 4
4x + 20 = x – 10
Transferring x from R.H.S to L.H.S becomes -x, 20 from L.H.S to R.H.S becomes -20.
4x – x = -10 – 20
3x = -30
Divide both sides by 3.
3x/3 = -30/3
x = -10
Therefore, the required solution set x = -10.
Example 5.
Solve the following equations and verify them.
(a) ⅓ (21 – 3x) = ½ (8 – 4x)
(b) (0.4y – 3)/(1.5y + 9) = -7/5
Solution:
(a) ⅓ (21 – 3x) = ½ (8 – 4x)
Cross-multiply the fractions.
2(21 – 3x) = 3(8 – 4x)
42 – 6x = 24 – 12x
Transferring -12x from R.H.S to L.H.S becomes 12x, 42 from L.H.S to R.H.S becomes -42.
-6x + 12x = 24 – 42
6x = -18
Divide both sides by 6.
6x/6 = -18/6
x = -3
Verification:
L.H.S = ⅓ (21 – 3x)
Put x = -3
L.H.S = ⅓ (21 – 3(-3))
= ⅓ (21 + 9)
= ⅓ (30)
= 10
R.H.S = ½ (8 – 4x)
Put x = -3
R.H.S = ½ (8 – 4(-3))
= ½ (8 + 12)
= ½ (20)
= 10
L.H.S = R.H.S
Hence proved.
(b) (0.4y – 3)/(1.5y + 9) = -7/5
Cross multiply the fractions.
5(0.4y – 3) = -7(1.5y + 9)
2y – 15 = -10.5y – 63
Transferring -10.5y from R.H.S to L.H.S becomes 10.5y, -15 from L.H.S to R.H.S becomes 15.
2y + 10.5y = 15 – 63
12.5y = -48
Divide both sides by 12.5
12.5y/12.5 = -48/12.5
y = -3.84
Verification:
L.H.S = (0.4y – 3)/(1.5y + 9)
Substitute y = -3.84
L.H.S = (0.4(-3.84) – 3) / (1.5(-3.84) + 9)
= (-1.536 – 3) / (-5.76 + 9)
= -4.536 / 3.24
= -1.4
R.H.S = -7/5
= -1.4
L.H.S = R.H.S.
Hence proved.