Proportion in Maths is an equation used to find that the two given ratios are equivalent to each other. Generally, we say that proportion defines that the equality of the two fractions of the ratios. If two sets of given numbers are increasing or decreasing in the same ratio with respect to each other, then the ratios are said to be directly proportional to each other. For example, the time taken by car to cover 200km per hour is equal to the time taken by it to cover the distance of 1200km for 6 hours. Such as 200km/hr = 1200km/6hrs.
Also, See:
Proportion – Formula
Let us take, in proportion, the two ratios are x:y and m:n. The two terms m and n are called means or mean terms and x and y are called extremes or extreme terms.
x : y :: m: n
\(\frac { x }{ y } \) = \(\frac { m }{ n } \)
Example:
Let us consider the number of persons in a theater. Our first ratio of the number of girls to boys is 5:7 and that of the other is 3:5, then the proportion can be written as:
5 : 7 :: 3 : 5 or 5/7 = 3/5
Here, 5 & 5 are the extremes, while 7 & 3 are the means.
Note: The ratio value does not affect when the same non-zero number is multiplied or divided on each term.
Important Properties of Proportion
The below are the important properties of proportions.
- Addendo – If a : b = c : d, then a + c : b + d
- Subtrahendo – If a : b = c : d, then a – c : b – d
- Componendo – If a : b = c : d, then a + b : b = c+d : d
- Dividendo – If a : b = c : d, then a – b : b = c – d : d
- Invertendo – If a : b = c : d, then b : a = d : c
- Alternendo – If a : b = c : d, then a : c = b: d
- Componendo and dividendo – If a : b = c : d, then a + b : a – b = c + d : c – d
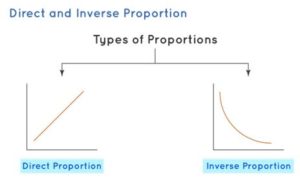
Types of Proportions
The Proportions are classified into two types. They are
- Direct Proportion
- Inverse Proportion
Direct Proportion: The Direct Proportion describes the direct relationship between two quantities. If one of the quantities increases, the other quantity increases and if one of the quantities decreases, the other quantity also decreases.
Example: If the speed of a vehicle increased, then it covers more distance in a fixed amount of time. It is denoted as y ∝ x.
Inverse Proportion: The Inverse Proportion describes the indirect relationship between two quantities. If one quantity increases, the other quantity decreases, and If one quantity decreases the other quantity increases. It is denoted as y ∝ 1/x.
Example: If a vehicle speed increases, then the result in converting a fixed distance in less time.
Important Points on Proportion
Check out the important points that need to remember in the proportion concept.
- Proportion is the comparison between two quantities.
- The proportions are two types. One is direct proportions and inverse proportions.
- Formula of proportion is \(\frac { x }{ y } \) = \(\frac { m }{ n } \)
- The proportion is an equation.
How to Solve Proportions?
Finding proportion is easy if the ratios are given. Follow the below procedure and find out the process to calculate proportions.
1. Multiply the first term with the last term: x x n
2. Multiply the second term with the third term: y x m
3. If the product of extreme terms is equal to the product of mean terms, then the ratios are proportional: x x n = y x m.
Continued Proportions
If we considered three quantities and the ratio of the first and second quantities is equal to the ratio between the second and the third quantities, then the three quantities are in Continued Proportions.
Example:
Let us take the ratios a:b and c:d
If a: b :: b: c, then we can say that a, b, c quantities are in continued proportion. Also, c is the third proportional of a and b.
b is called the mean proportional between a and C.
If a, b, c are in continued proportion then b² = ac or b = √ac.
Proportion Examples with Answers
Example 1.
Determine if 4, 7, 8, 14 are in proportion?
Solution:
Given numbers are 4, 7, 8, 14.
From the given data, extreme terms are 4 and 13, mean terms are 7 and 6.
Find the Product of extreme terms and mean terms.
Product of extreme terms = 4 × 14 = 56
Product of mean terms = 7 × 8 = 56.
Compare the Product of extreme terms and the Product of mean terms.
The product of means = product of extremes
56 = 56
Therefore, 4, 7, 8, 14 are in proportion.
Example 2.
Check if 3, 6, 12 are in proportion.
Solution:
Given numbers are 3, 6, 12.
From the given data, 3 is the first term, 6 is the middle term, and 12 is the third term.
Find the Product of the first term and third term.
Product of first and third term = 3 × 12 = 36
Square of the middle terms = 6 × 6 = 36 = 3 × 12.
Compare the Product of the first and third term and Square of the middle terms.
The Product of first and third term = Square of the middle terms
56 = 56
Therefore, 3, 6, 12 are in proportion, and 6 is called the mean proportional between 3 and 12.
Example 3.
Find the fourth proportional to 3, 19, 21?
Solution:
Given numbers are 3, 19, 21.
To find the fourth Proportional, let us assume the fourth proportional is x.
Then, 3: 19 :: 21: x
Compare the Product of extreme terms and Product of mean terms.
3x = 19 × 21
3x = 399
x = 399/3
x = 133.
Hence, the fourth proportional to 3, 19, 21 is 133.
Example 4.
Find the third proportional to 4 and 8?
Solution:
Given numbers are 4 and 8.
Let the third proportional to 4 and 8 be x.
Compare the Product of the first and third term and Square of the middle terms.
4x = 8 × 8
4x = 64
x = 64/4
x = 16
Therefore, the third proportional to 4 and 8 is 16.
Example 5.
The ratio of income to expenditure is 3: 4. Find the savings if the expenditure is $24,000.
Solution:
Given that the ratio of income to expenditure is 3: 4.
Therefore, income = $ (3 × 24000)/4 = $18000
Savings = Income – Expenditure = $24,000 – $18000 = $6000
The savings are $6000 if the expenditure is $24,000.
Example 6.
Find the mean proportional between 3 and 27?
Solution:
Given numbers are 3 and 27.
Let the mean proportional between 3 and 27 be x.
Then, x × x = 3 × 27
x² = 81
x = √81
x = 9.
Therefore, the mean proportion between 3 and 27 is 9.