Do you want to learn about the Relation among All Trigonometric Ratios of (180° – θ)? Then, halt your search here as we have explained the Relationship between Trigonometric Functions of 180 Minus Theta in detail along with their proofs. Find Solved Examples on finding the Trigonometric Ratios with Step by Step Explanation making it easy for you to solve related problems in no time. Know a Simple Formula to memorize the Trigonometric Functions.
How to Determine the Trigonometric Ratios of (180° – θ)?
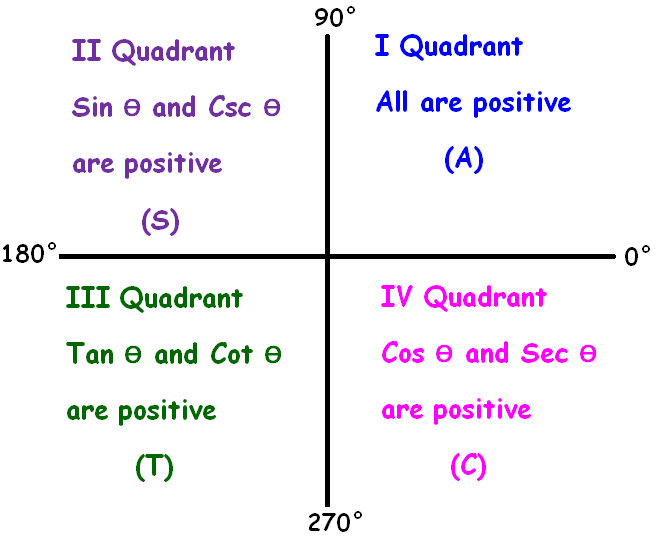
Before diving deep into the article to understand how trigonometric ratios of (180° – θ) are determined you need to understand the ASTC Formula.
The ASTC Formula can be easily remembered by considering the Phrases provided below
“All Silver Tea Cups” or ” All Students Take Calculus”
You will better understand the concept by having a glance at the below picture. From the picture, it is clearly evident that 180 degrees minus theta fall under the 2nd Quandrant. In the 2nd Quadrant, only sin and cosecant are Positive.
sin(180° – θ)=sinθ
cos(180° – θ)=−cosθ
tan(180° – θ)=−tanθ
cosec (180° – θ)=cosec θ
sec(180° – θ)=−secθ
cot(180° – θ)=−cotθ
Read More Articles:
- Trigonometrical Ratios of 90 Degree Plus Theta
- Trigonometrical Ratios Table
- Worksheet on Trigonometric Identities
Evaluate Trigonometric Functions of (180° – θ)
1. Evaluate Sin(180° – θ)?
Solution:
Sin(180° – θ) = Sin (90° + 90° – θ)
= Sin [90° + (90° – θ)]
= Cos (90° – θ), [since Sin (90° + θ) = Cos θ]
= Sin θ[since Cos (90° – θ) = Sin θ]
Therefore, Sin (180° – θ) = Sin θ
2. Evaluate Cos(180° – θ)?
Cos (180° – θ) = Cos (90° + 90° – θ)
= Cos [90° + (90° – θ)]
= – Sin (90° – θ), [since Cos (90° + θ) = -Sin θ]
= -Cos θ [since sin (90° – θ) = cos θ]
Therefore, Cos (180° – θ) = – Cos θ
3. Evaluate Tan (180° – θ)?
Solution:
Tan (180° – θ) = Tan (90° + 90° – θ)
= Tan [90° + (90° – θ)] [since Tan (90° + θ) = -Cot θ]
= – Cot (90° – θ)
= Tan θ [since Cot (90° – θ) = Tan θ]
Therefore, Tan (180° – θ) = – Tan θ
4. Evaluate Csc (180° – θ)?
Solution:
Csc (180° – θ) = \(\frac { 1 }{ Sin(180° – θ) } \)
= \(\frac { 1 }{ Sin θ } \) [Since Sin(180° – θ) = Sin θ]
Therefore, Csc (180° – θ) = \(\frac { 1 }{ Sin θ } \)
5. Evaluate Sec (180° – θ)?
Solution:
Sec (180° – θ) = \(\frac { 1 }{ Cos(180° – θ) } \)
= = \(\frac { 1 }{ -Cos θ } \) [Since Cos(180° – θ) = -Cos θ]
= -Sec θ
Therefore, Sec (180° – θ) = -Sec θ
6. Evaluate Cot (180° – θ)?
Solution:
Cot (180° – θ) = \(\frac { 1 }{ Tan(180° – θ) } \)
= \(\frac { 1 }{ -Tan θ } \) [Since Tan(180° – θ) = -Tan θ]
= -Cot θ
Solved Examples on Trigonometric Ratios
1. Find the Value of Cos 150°?
Solution:
Cos 150° = Cos(180° – 30°)
= – Cos 30°
= – \(\frac { √3 }{ 2 } \)
2. Find the value of Cot 135°?
Solution:
Cot 135° = Cot(180° – 45°)
= -Cot 45°
= -1