Engage NY Eureka Math 8th Grade Module 4 Lesson 24 Answer Key
Eureka Math Grade 8 Module 4 Lesson 24 Exercise Answer Key
Exercises
Exercise 1.
Derek scored 30 points in the basketball game he played, and not once did he go to the free throw line. That means that Derek scored two-point shots and three-point shots. List as many combinations of two- and three-pointers as you can that would total 30 points.
Answer:
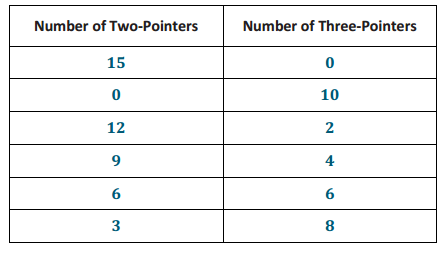
Write an equation to describe the data.
Answer:
Let x represent the number of 2-pointers and y represent the number of 3-pointers.
30 = 2x + 3y
Exercise 2.
Derek tells you that the number of two-point shots that he made is five more than the number of three-point shots. How many combinations can you come up with that fit this scenario? (Don’t worry about the total number of points.)
Answer:
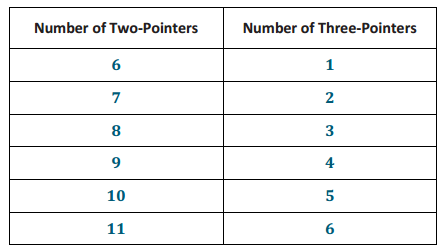
Write an equation to describe the data.
Answer:
Let x represent the number of two-pointers and y represent the number of three-pointers.
x = 5 + y
Exercise 3.
Which pair of numbers from your table in Exercise 2 would show Derek’s actual score of 30 points?
Answer:
The pair 9 and 4 would show Derek’s actual score of 30 points.
Exercise 4.
Efrain and Fernie are on a road trip. Each of them drives at a constant speed. Efrain is a safe driver and travels 45 miles per hour for the entire trip. Fernie is not such a safe driver. He drives 70 miles per hour throughout the trip. Fernie and Efrain left from the same location, but Efrain left at 8:00 a.m., and Fernie left at 11:00 a.m. Assuming they take the same route, will Fernie ever catch up to Efrain? If so, approximately when?
a. Write the linear equation that represents Efrain’s constant speed. Make sure to include in your equation the extra time that Efrain was able to travel.
Answer:
Efrain’s rate is \(\frac{45}{1}\) miles per hour, which is the same as 45 miles per hour. If he drives y miles in x hours at that constant rate, then y = 45x. To account for his additional 3 hours of driving time that Efrain gets, we write the equation y = 45(x + 3).
y = 45x + 135
b. Write the linear equation that represents Fernie’s constant speed.
Answer:
Fernie’s rate is \(\frac{70}{1}\) miles per hour, which is the same as 70 miles per hour. If he drives y miles in x hours at that constant rate, then y = 70x.
c. Write the system of linear equations that represents this situation.
Answer:
y = 45x + 135
y = 70x
d. Sketch the graphs of the two linear equations.
Answer:
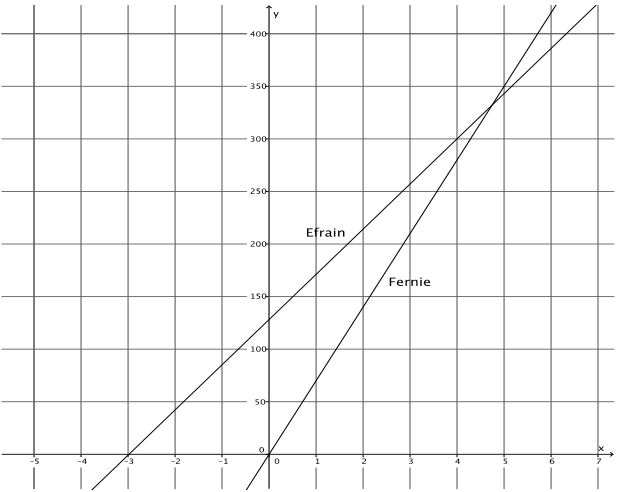
e. Will Fernie ever catch up to Efrain? If so, approximately when?
Answer:
Yes, Fernie will catch up to Efrain after about 4 \(\frac{1}{2}\) hours of driving or after traveling about 325 miles.
f. At approximately what point do the graphs of the lines intersect?
Answer:
The lines intersect at approximately (4.5, 325).
Exercise 5.
Jessica and Karl run at constant speeds. Jessica can run 3 miles in 24 minutes. Karl can run 2 miles in 14 minutes. They decide to race each other. As soon as the race begins, Karl trips and takes 2 minutes to recover.
a. Write the linear equation that represents Jessica’s constant speed. Make sure to include in your equation the extra time that Jessica was able to run.
Answer:
Jessica’s rate is \(\frac{3}{24}\) miles per minute, which is equivalent to \(\frac{1}{8}\) miles per minute. If Jessica runs y miles x minutes at that constant speed, then y = \(\frac{1}{8}\) x. To account for her additional 2 minute of running that Jessica gets, we write the equation
y = \(\frac{1}{8}\) (x + 2)
y = \(\frac{1}{8}\) x + \(\frac{1}{4}\)
b. Write the linear equation that represents Karl’s constant speed.
Answer:
Karl’s rate is \(\frac{2}{14}\) miles per minute, which is the same as \(\frac{1}{7}\) miles per minute. If Karl runs y miles in x minutes at that constant speed, then y = \(\frac{1}{7}\) x.
c. Write the system of linear equations that represents this situation.
Answer:
y = \(\frac{1}{8}\) x + \(\frac{1}{8}\)
y = \(\frac{1}{7}\) x
d. Sketch the graphs of the two linear equations.
Answer:
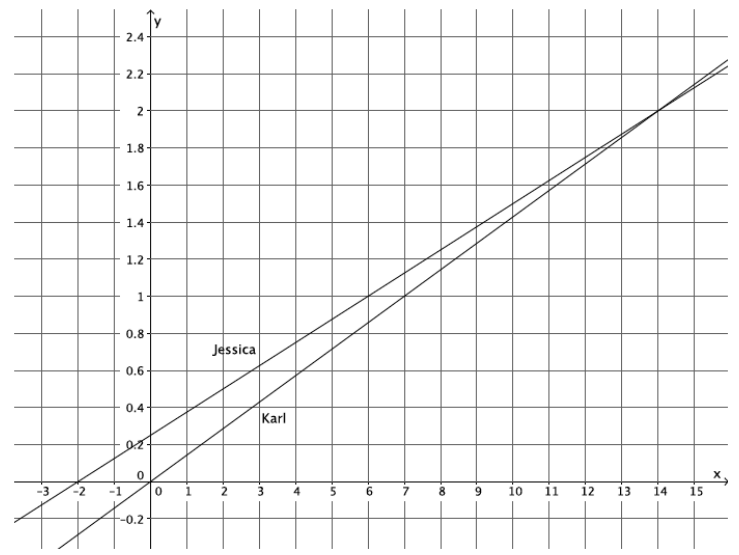
e. Use the graph to answer the questions below.
i. If Jessica and Karl raced for 3 miles, who would win? Explain.
Answer:
If the race were 3 miles, then Karl would win. It only takes Karl 21 minutes to run 3 miles, but it takes Jessica 24 minutes to run the distance of 3 miles.
ii. At approximately what point would Jessica and Karl be tied? Explain.
Answer:
Jessica and Karl would be tied after about 4 minutes or a distance of 1 mile. That is where the graphs of the lines intersect.
Eureka Math Grade 8 Module 4 Lesson 24 Problem Set Answer Key
Question 1.
Jeremy and Gerardo run at constant speeds. Jeremy can run 1 mile in 8 minutes, and Gerardo can run 3 miles in 33 minutes. Jeremy started running 10 minutes after Gerardo. Assuming they run the same path, when will Jeremy catch up to Gerardo?
a. Write the linear equation that represents Jeremy’s constant speed.
Answer:
Jeremy’s rate is \(\frac{1}{8}\) miles per minute. If he runs y miles in x minutes, then y = \(\frac{1}{8}\) x.
b. Write the linear equation that represents Gerardo’s constant speed. Make sure to include in your equation the extra time that Gerardo was able to run.
Answer:
Gerardo’s rate is \(\frac{3}{33}\) miles per minute, which is the same as \(\frac{1}{11}\) miles per minute. If he runs y miles in x minutes, then y = \(\frac{1}{11}\) x. To account for the extra time that Gerardo gets to run, we write the equation
y = \(\frac{1}{11}\) (x + 10)
y = \(\frac{1}{11}\) x + \(\frac{10}{11}\)
c. Write the system of linear equations that represents this situation.
Answer:
y = \(\frac{1}{8}\) x
y = \(\frac{1}{11}\) x + \(\frac{10}{11}\)
d. Sketch the graphs of the two equations.
Answer:
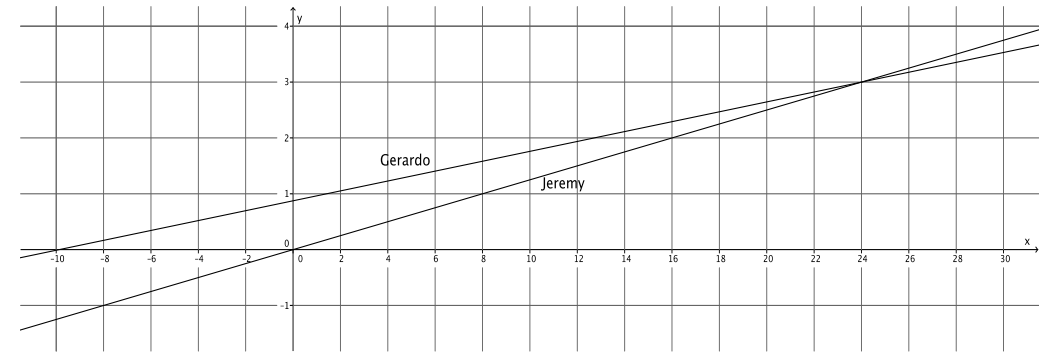
e. Will Jeremy ever catch up to Gerardo? If so, approximately when?
Answer:
Yes, Jeremy will catch up to Gerardo after about 24 minutes or about 3 miles.
f. At approximately what point do the graphs of the lines intersect?
Answer:
The lines intersect at approximately (24, 3).
Question 2.
Two cars drive from town A to town B at constant speeds. The blue car travels 25 miles per hour, and the red car travels 60 miles per hour. The blue car leaves at 9:30 a.m., and the red car leaves at noon. The distance between the two towns is 150 miles.
a. Who will get there first? Write and graph the system of linear equations that represents this situation.
Answer:
The linear equation that represents the distance traveled by the blue car is y = 25(x + 2.5), which is the same as y = 25x + 62.5. The linear equation that represents the distance traveled by the red car is
y = 60x. The system of linear equations that represents this situation is
y = 25x + 62.5
y = 60x
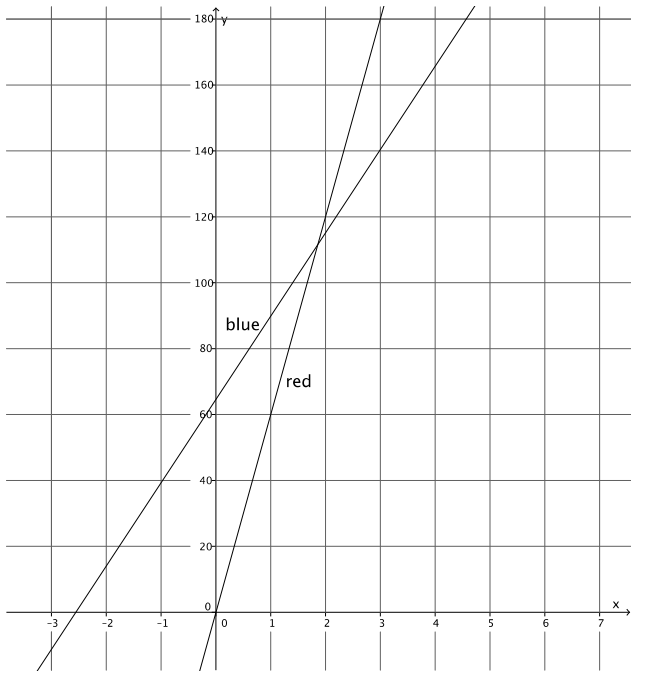
The red car will get to town B first.
b. At approximately what point do the graphs of the lines intersect?
Answer:
The lines intersect at approximately (1.8, 110).
Eureka Math Grade 8 Module 4 Lesson 24 Exit Ticket Answer Key
Question 1.
Darnell and Hector ride their bikes at constant speeds. Darnell leaves Hector’s house to bike home. He can bike the 8 miles in 32 minutes. Five minutes after Darnell leaves, Hector realizes that Darnell left his phone. Hector rides to catch up. He can ride to Darnell’s house in 24 minutes. Assuming they bike the same path, will Hector catch up to Darnell before he gets home?
a. Write the linear equation that represents Darnell’s constant speed.
Answer:
Darnell’s rate is \(\frac{1}{4}\) miles per minute. If he bikes y miles in x minutes at that constant speed, then y = \(\frac{1}{4}\) x.
b. Write the linear equation that represents Hector’s constant speed. Make sure to take into account that Hector left after Darnell.
Answer:
Hector’s rate is \(\frac{1}{3}\) miles per minute. If he bikes y miles in x minutes, then y = \(\frac{1}{3}\) x. To account for the extra time Darnell has to bike, we write the equation
y = \(\frac{1}{3}\) (x – 5)
y = \(\frac{1}{3}\) x-\(\frac{5}{3}\)
c. Write the system of linear equations that represents this situation.
Answer:
y = \(\frac{1}{4}\) x
y = \(\frac{1}{3}\) x-\(\frac{5}{3}\)
d. Sketch the graphs of the two equations.
Answer:
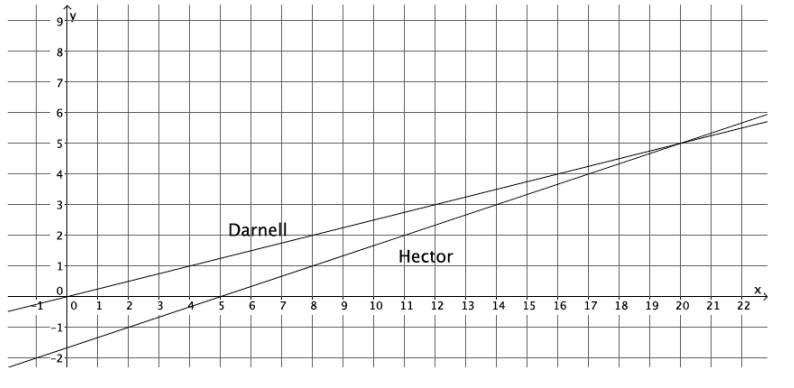
e. Will Hector catch up to Darnell before he gets home? If so, approximately when?
Answer:
Hector will catch up 20 minutes after Darnell left his house (or 15 minutes of biking by Hector) or approximately 5 miles.
f. At approximately what point do the graphs of the lines intersect?
Answer:
The lines intersect at approximately (20, 5).