Eureka Math Grade 6 Module 4 Lesson 5 Answer Key

Engage NY Eureka Math 6th Grade Module 4 Lesson 5 Answer Key

Eureka Math Grade 6 Module 4 Lesson 5 Example Answer Key

Write each expression in exponential form.
Example 1.
5 × 5 × 5 × 5 × 5 =
Answer:
5⁵

Example 2.
2 × 2 × 2 × 2 =
Answer:
2⁴

Write each expression in expanded form.
Example 3.
8³ =
Answer:
8 × 8 × 8

Example 4.
10⁶ =
Answer:
10 × 10 × 10 × 10 × 10 × 10

Example 5.
g³ =
Answer:
g × g × g

Go back to Examples 1 – 4, and use a calculator to evaluate the expressions.

Example 1.
5 × 5 × 5 × 5 × 5 = 5⁵
Answer:
3,125

Example 2.
2 × 2 × 2 × 2 = 2⁴
Answer:
16

Example 3.
8³ = 8 × 8 × 8
Answer:
512

Example 4.
10⁶ = 10 × 10 × 10 × 10 × 10 × 10
Answer:
1,000,000

Example 5.
What is the difference between 3g and g³?
Answer:
3g = g + g + g or 3 times g; g³ = g × g × g

Example 6.
Write the expression in expanded form, and then evaluate.
(3.8)⁴ =
Answer:
3.8 × 3.8 × 3.8 × 3.8 = 208.5136

Example 7.
Write the expression in exponential form, and then evaluate.
Answer:
2.1 × 2.1 = (2.1)² = 4.41

Example 8.
Write the expression in exponential form, and then evaluate.
0.75 × 0.75 × 0.75
Answer:
= (0.75)³ = 0.421875

The base number can also be a fraction. Convert the decimals to fractions in Examples 7 and 8 and evaluate. Leave your answer as a fraction. Remember how to multiply fractions!

Example 7.
Answer:
\(\frac{21}{10} \times \frac{21}{10}=\left(\frac{21}{10}\right)^{2}=\frac{441}{100}=4 \frac{41}{100}\)

Example 8.
Answer:
\(\frac{3}{4} \times \frac{3}{4} \times \frac{3}{4}=\left(\frac{3}{4}\right)^{3}=\frac{27}{64}\)

Example 9.
Write the expression in exponential form, and then evaluate.
Answer:
\(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}=\left(\frac{1}{2}\right)^{3}=\frac{1}{8}\)

Example 10.
Write the expression in expanded form, and then evaluate.
Answer:
\(\left(\frac{2}{3}\right)^{2}=\frac{2}{3} \times \frac{2}{3}=\frac{4}{9}\)

Eureka Math Grade 6 Module 4 Lesson 5 Exercise Answer Key

Exercise 1.
Fill in the missing expressions for each row. For whole number and decimal bases, use a calculator to find the standard form of the number. For fraction bases, leave your answer as a fraction.

Exponential Form	Expanded Form	Standard Form
32	3 × 3	9
	2 × 2 × 2 × 2 × 2 × 2
45
	\( \frac{3}{4} \times \frac{3}{4} \)
	1.5 × 1.5

Answer:

Exponential Form	Expanded Form	Standard Form
32	3 × 3	9
26	2 × 2 × 2 × 2 × 2 × 2	64
45	4 × 4 × 4 × 4 × 4	1,024
\(\left(\frac{3}{4}\right)^{2}\)	\( \frac{3}{4} \times \frac{3}{4} \)	\( \frac{9}{16} \)
(1.5)2	1.5 × 1.5	2.25

Exercise 2.
Write five cubed in all three forms: exponential form, expanded form, and standard form.
Answer:
5³; 5 × 5 × 5; 125

Exercise 3.
Write fourteen and seven-tenths squared in all three forms.
Answer:
(14.7)²; 14.7 × 14.7; 216.09

Exercise 4.
One student thought two to the third power was equal to six. What mistake do you think he made, and how would you help him fix his mistake?
Answer:
The student multiplied the base, 2, by the exponent, 3. This is wrong because the exponent never multiplies the base; the exponent tells how many copies of the base are to be used as factors.

Eureka Math Grade 6 Module 4 Lesson 5 Problem Set Answer Key

Question 1.
Complete the table by filling in the blank cells. Use a calculator when needed.

Exponential Form	Expanded Form	Standard Form
35
	4 × 4 × 4
(1.9)2
\(\left(\frac{1}{2}\right)^{5}\)

Answer:

Exponential Form	Expanded Form	Standard Form
35	3 × 3 × 3 × 3 × 3	243
43	4 × 4 × 4	64
(1.9)2	1.9 × 1.9	3.61
\(\left(\frac{1}{2}\right)^{5}\)	\( \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \)	\( \frac{1}{32} \)

Question 2.
Why do whole numbers raised to an exponent get greater, while fractions raised to an exponent get smaller?
Answer:
As whole numbers are multiplied by themselves, products are larger because there are more groups. As fractions of fractions are taken, the product is smaller. A part of a part is less than how much we started with.

Question 3.
The powers of 2 that are in the range 2 through 1,000 are 2, 4, 8, 16, 32, 64, 128, 256, and 512. Find all the powers of that are in the range 3 through 1,000.
Answer:
3, 9, 27, 81, 243, 729

Question 4.
Find all the powers of 4 in the range 4 through 1,000.
Answer:
4, 16, 64, 256

Question 5.
Write an equivalent expression for n × a using only addition.
Answer:

Question 6.
Write an equivalent expression for w^b using only multiplication.
Answer:

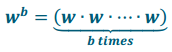
a. Explain what w is in this new expression.
Answer:
w is the factor that will be repeatedly multiplied by itself.

b. Explain what b is in this new expression.
Answer:
b is the number of times w will be multiplied.

Question 7.
What is the advantage of using exponential notation?
Answer:
It is a shorthand way of writing a multiplication expression if the factors are all the same.

Question 8.
What is the difference between 4x and x⁴? Evaluate both of these expressions when x = 2
Answer:
4x means four times x; this is the same as x + x + x + x. On the other hand, x⁴ means x to the fourth power, or x × x × x × x.
When x = 2, 4x = 4 × 2 = 8.
When x = 2, x⁴ = 2 × 2 × 2 × 2 = 16.

Eureka Math Grade 6 Module 4 Lesson 5 Exit Ticket Answer Key

Question 1.
What is the difference between 6z and z⁶?
Answer:
6z = z + z + z + z + z + z or 6 times z; z⁶ = z × z × z × z × z × z

Question 2.
Write 10³ as a multiplication expression having repeated factors.
Answer:
10 × 10 × 10

Question 3.
Write 8 × 8 × 8 × 8 using an exponent.
Answer:
8⁴

Eureka Math Grade 6 Module 4 Lesson 5 Opening Exercise Answer Key

As you evaluate these expressions, pay attention to how you arrive at your answers.

Question 1.
4 + 4 + 4 + 4 + 4 + 4 + 4 + 4 + 4 + 4
Answer:
4 + 4 + 4 + 4 + 4 + 4 + 4 + 4 + 4 + 4 = 4 × 10
= 4¹⁰

Question 2.
9 + 9 + 9 + 9 + 9
Answer:
9 + 9 + 9 + 9 + 9 = 9 × 5
= 9⁵

Question 3.
10 + 10 + 10 + 10 + 10
Answer:
10 + 10 + 10 + 10 + 10 = 10 × 5
= 10⁵

Eureka Math Grade 6 Module 4 Lesson 5 Multiplication of Decimals Answer Key

Progression of Exercises

Question 1.
0.5 × 0.5 =
Answer:
0.25

Question 2.
0.6 × 0.6 =
Answer:
0.36

Question 3.
0.7 × 0.7
Answer:
0.49

Question 4.
0.5 × 0.6 =
Answer:
0.3

Question 5.
1.5 × 1.5 =
Answer:
0.25

Question 6.
2.5 × 2.5 =
Answer:
6.25

Question 7.
0.25 × 0.25 =
Answer:
0.0625

Question 8.
0.1 × 0.1 =
Answer:
0.01

Question 9.
0.1 × 123.4 =
Answer:
12.34

Question 10.
0.01 × 123.4 =
Answer:
1.234