Engage NY Eureka Math 8th Grade Module 2 Lesson 11 Answer Key
Eureka Math Grade 8 Module 2 Lesson 11 Example Answer Key
Example 1.
The advantage of the scientific notation becomes even more pronounced when we have to compute how many times heavier a proton is than an electron. Instead of writing the value of the ratio, r, as
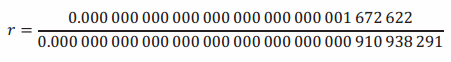
we express it as
r=\(\frac{1.672622 \times 10^{-27}}{9.10938291 \times 10^{-31}}\).
→ Should we eliminate the power of 10 in the numerator or denominator? Explain.
→ Using the theorem on generalized equivalent fractions, we can eliminate the negative power of 10 in the numerator and denominator to see what we are doing more clearly. Anticipating that
10-31×1031=1, we can multiply the numerator and denominator of the (complex) fraction by 1031
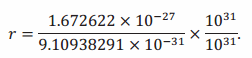
Using the first law of exponents (10) presented in Lesson 5, we get
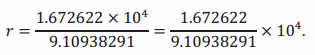
Note that since we are using scientific notation, we can interpret an approximate value of r right away. For example, we see
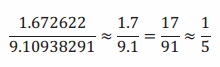
so that r is approximately \(\frac{1}{5}\) ×10,000, which is 2,000. Thus, we expect a proton to be about two thousand times heavier than an electron.
Example 2.
The U.S. national debt as of March 23, 2013, rounded to the nearest dollar, is $16,755,133,009,522. According to the 2012 U.S. census, there are about 313,914,040 U.S. citizens. What is each citizen’s approximate share of the debt?
Answer:
\(\frac{1.6755 \times 10^{13}}{3.14 \times 10^{8}}\)=\(\frac{1.6755}{3.14}\)×\(\frac{10^{13}}{10^{8}}\)
=\(\frac{1.6755}{3.14}\)×105
=0.533598…×105
≈0.5336×105
=53360
Each U.S. citizen’s share of the national debt is about $53,360.
Eureka Math Grade 8 Module 2 Lesson 11 Exercise Answer Key
Exercise 1.
The mass of a proton is
0.000 000 000 000 000 000 000 000 001 672 622 kg.
In scientific notation it is
Answer:
1.672622×10-27 kg.
Exercise 2.
The mass of an electron is
0.000 000 000 000 000 000 000 000 000 000 910 938 291 kg.
In scientific notation it is
Answer:
9.10938291×10-31 kg.
Exercise 3.
Write the ratio that compares the mass of a proton to the mass of an electron.
Answer:
Ratio: (1.672622×10-27) :(9.10938291×10-31)
Exercise 4.
Compute how many times heavier a proton is than an electron (i.e., find the value of the ratio). Round your final answer to the nearest one.
Answer:
Let r= the value of the ratio, then:
r=\(\frac{1.672622 \times 10^{-27}}{9.10938291 \times 10^{-31}}\)
=\(\frac{1.672622 \times 10^{-27} \times 10^{31}}{9.10938291 \times 10^{-31} \times 10^{31}}\)
=\(\frac{1.672622 \times 10^{4}}{9.10938291}\)×104
=\(\frac{1.672622 \times 10^{8}}{9.10938291 \times 10^{8}}\) ×104
=\(\frac{167,262,200}{910,938,291}\)×104
=0.183615291675×104
=1836.15291675
≈1836
Exercise 5.
The geographic area of California is 163,696 sq.mi., and the geographic area of the U.S. is 3,794,101 sq.mi. Let’s round off these figures to 1.637×105 and 3.794×106. In terms of area, roughly estimate how many Californias would make up one U.S. Then compute the answer to the nearest ones.
Answer:
\(\frac{3.794 \times 10^{6}}{1.637 \times 10^{5}}\)=\(\frac{3.794}{1.637}\)×\(\frac{10^{6}}{10^{5}}\)
=\(\frac{3.794}{1.637}\)×10
=2.3176…×10
≈2.318×10
=23.18
It would take about 23 Californias to make up one U.S.
Exercise 6.
The average distance from Earth to the moon is about 3.84×105 km, and the distance from Earth to Mars is approximately 9.24×107 km in year 2014. On this simplistic level, how much farther is traveling from Earth to Mars than from Earth to the moon?
9.24×107-3.84×105=924×105-3.84×105
=(924-3.84)×105
=920.16×105
=92 016 000
It is 92,016,000 km further to travel from Earth to Mars than from Earth to the moon.
Eureka Math Grade 8 Module 2 Lesson 11 Problem Set Answer Key
Question 1.
There are approximately 7.5×1018 grains of sand on Earth. There are approximately 7×1027 atoms in an average human body. Are there more grains of sand on Earth or atoms in an average human body? How do you know?
Answer:
There are more atoms in the average human body. When comparing the order of magnitude of each number,
27>18; therefore, 7×1027>7.5×1018.
Question 2.
About how many times more atoms are in a human body compared to grains of sand on Earth?
Answer:
\(\frac{7 \times 10^{27}}{7.5 \times 10^{18}}\)=\(\frac{7}{7.5}\)×\(\frac{10^{27}}{10^{18}}\)
≈1×1027-18
≈1×109
≈109
There are about 1,000,000,000 times more atoms in the human body compared to grains of sand on Earth.
Question 3.
Suppose the geographic areas of California and the U.S. are 1.637×105 and 3.794×106 sq.mi., respectively. California’s population (as of 2012) is approximately 3.804×107 people. If population were proportional to area, what would be the U.S. population?
Answer:
We already know from Exercise 5 that it would take about 23 Californias to make up one U.S. Then the population of the U.S. would be 23 times the population of California, which is
23×3.804×107=87.492×107
=8.7492×108
=874,920,000.
Question 4.
The actual population of the U.S. (as of 2012) is approximately 3.14×108. How does the population density of California (i.e., the number of people per square mile) compare with the population density of the U.S.?
Answer:
Population density of California per square mile:
\(\frac{3.804 \times 10^{7}}{1.637 \times 10^{5}}\)=\(\frac{3.804}{1.637}\)×\(\frac{10^{7}}{10^{5}}\)
=2.32376…×102
≈2.32×102
=232
Population density of the U.S. per square mile:
\(\frac{3.14 \times 10^{8}}{3.794 \times 10^{6}}\)=\(\frac{3.14}{3.794}\)×\(\frac{10^{8}}{10^{6}}\)
=0.8276…×102
≈0.83×102
=83
Population density of California compared to the population density of the U.S.:
\(\frac{232}{83}\)=2.7951…
≈2.8
California is about 3 times as dense as the U.S. in terms of population.
Eureka Math Grade 8 Module 2 Lesson 11 Exit Ticket Answer Key
Question 1.
Two of the largest mammals on earth are the blue whale and the elephant. An adult male blue whale weighs about 170 tonnes or long tons. (1 tonne = 1000 kg)
Show that the weight of an adult blue whale is 1.7×105 kg.
Answer:
Let x (or any other symbol) represent the number of kilograms an adult blue whale weighs.
170×1000=x
1.7×105=x
Question 2.
An adult male elephant weighs about 9.07×103 kg.
Compute how many times heavier an adult male blue whale is than an adult male elephant (i.e., find the value of the ratio). Round your final answer to the nearest one.
Answer:
Let r be the value of the ratio.
r=\(\frac{1.7 \times 10^{5}}{9.07 \times 10^{3}}\)
=\(\frac{1.7}{9.07}\)×102
=0.18743×102
=18.743
≈19
The blue whale is 19 times heavier than the elephant.